Next: Linearized Reduced Drift-MHD Equations Up: Linear Resonant Response Model Previous: Reduced Drift-MHD Model Contents
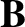 |
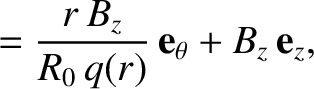 |
(5.18) |
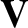 |
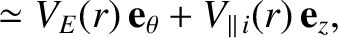 |
(5.19) |
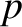 |
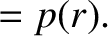 |
(5.20) |
 -component of the) E-cross-B velocity [see Equation (2.138)], and
-component of the) E-cross-B velocity [see Equation (2.138)], and
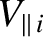 the ion parallel fluid velocity.
the ion parallel fluid velocity.
Now, the resonant layer is assumed to have a radial thickness that is
much smaller than 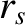 . (See Section 5.5.) Hence, we only need to evaluate plasma equilibrium quantities in the immediate vicinity of the rational
surface. Equations (4.1), (4.23), (4.24), and (5.1)–(5.4) imply that
. (See Section 5.5.) Hence, we only need to evaluate plasma equilibrium quantities in the immediate vicinity of the rational
surface. Equations (4.1), (4.23), (4.24), and (5.1)–(5.4) imply that
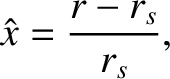 |
(5.26) |
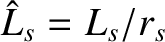 ,
is the magnetic shear length,
,
is the magnetic shear length,
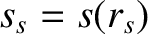 ,
the magnetic shear,
,
the magnetic shear,
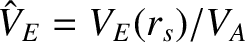 ,
,
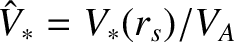 ,
the (dominant
,
the (dominant  -component of the) diamagnetic velocity [see Equation (4.11)],
and
-component of the) diamagnetic velocity [see Equation (4.11)],
and
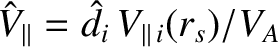 . We also have
Note that we are neglecting any radial gradients in the equilibrium MHD fluid velocity because, in conventional tokamak plasmas, such gradients do not significantly affect the linear response of a resonant
layer, due to the fact that the gradients are comparatively weak (i.e.,
. We also have
Note that we are neglecting any radial gradients in the equilibrium MHD fluid velocity because, in conventional tokamak plasmas, such gradients do not significantly affect the linear response of a resonant
layer, due to the fact that the gradients are comparatively weak (i.e.,
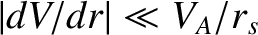 ) combined with the fact that a linear resonant layer is
very narrow [6]. (See Section 5.5.)
) combined with the fact that a linear resonant layer is
very narrow [6]. (See Section 5.5.)