Next: Magnetic Reconnection Up: Linear Resonant Response Model Previous: Numerical Solution of Resonant Contents
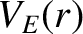 , will be similar in magnitude to the diamagnetic velocity,
, will be similar in magnitude to the diamagnetic velocity, 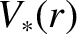 , in a tokamak fusion
reactor. [See Equations (5.21) and (5.29).] Given that most existing tokamak plasmas are characterized
by levels of plasma rotation such that
, in a tokamak fusion
reactor. [See Equations (5.21) and (5.29).] Given that most existing tokamak plasmas are characterized
by levels of plasma rotation such that
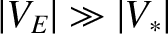 , this assumption needs justification.
, this assumption needs justification.
Now, as was explained
in Section 1.8, a tokamak fusion reactor requires auxiliary heating in order to attain plasma temperatures
sufficient for nuclear fusion. Most existing tokamaks employ neutral beam injection as their primary auxiliary heating method [20]. In this heating scheme, high energy (i.e., 100 keV) neutral particles are injected into the plasma. The neutral
particles are ionized within the plasma to form energetic ions and low energy electrons. The energetic ions are confined by the tokamak's
magnetic field, and subsequently slow down and heat the plasma. It is vitally important that the neutral particles
are ionized within the plasma, otherwise they will pass completely through it and damage the plasma facing
components. In order to avoid this problem, which is known as shine-through, most existing neutral beam injection systems angle the beam so that it
passes through the plasma tangentially, rather than radially, in order to maximize the path-length of the beam through the
plasma. Neutral particle ions have relatively large gyroradii and banana radii before they slow down. In order to minimize fast ion losses, the neutral particle beam is usually angled through the plasma in the direction such that,
immediately after ionization, the interaction between the fast ions and the tokamak's magnetic field
causes the ions to move radially inwards, rather than outwards. It follows from the analysis of Section 2.7
that, in a tokamak in which the toroidal magnetic field and plasma current are both directed in the 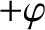 direction,
the beam needs to be angled such that fast ions are injected in the
direction,
the beam needs to be angled such that fast ions are injected in the 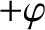 , rather than the
, rather than the 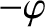 , direction.
This scheme is known as co-injection, because the beam injection direction is parallel to the toroidal plasma
current.
, direction.
This scheme is known as co-injection, because the beam injection direction is parallel to the toroidal plasma
current.
A co-injected neutral beam imparts 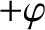 toroidal momentum, as well as energy, to a tokamak plasma. Roughly
speaking, the ratio of the momentum to the energy injected by the beam is
toroidal momentum, as well as energy, to a tokamak plasma. Roughly
speaking, the ratio of the momentum to the energy injected by the beam is
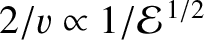 , where
, where 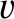 and
and
 are the speed and energy, respectively, of the injected particles. The injected toroidal momentum causes the plasma to spin rapidly in the ion diamagnetic direction (i.e., in the same direction as ion diamagnetic flows).
Plasmas in most existing tokamak experiments are characterized by
are the speed and energy, respectively, of the injected particles. The injected toroidal momentum causes the plasma to spin rapidly in the ion diamagnetic direction (i.e., in the same direction as ion diamagnetic flows).
Plasmas in most existing tokamak experiments are characterized by
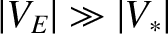 as a consequence of co-injected neutral beams.
as a consequence of co-injected neutral beams.
The low-field tokamak fusion reactor (i.e., ITER–see Section 1.5 and [19]) considered in this book is sufficiently large and high density than only exceptionally high energy (i.e. 1000 keV) neutral particles can penetrate to its core. Given that the ratio of the momentum to the energy injected by a neutral beam system is inversely proportional to the square root of the neutral particle injection energy, it follows that only a relatively small amount of momentum will be injected into ITER plasmas by its neutral beam heating system, compared to existing tokamaks.
The high-field tokamak fusion reactor (i.e., SPARC [8]) considered in this book will not possess a neutral particle heating system at all. Instead, it will rely on solely on auxiliary heating by means of radio-frequency electromagnetic waves injected into the plasma. Such heating schemes do not inject any appreciable momentum into the plasma.
In the absence of a significant toroidal momentum source, there is no reason to suppose that a tokamak plasma
will rotate in such a manner that 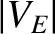 greatly exceeds
greatly exceeds 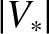 . Given that the two planned tokamak
fusion reactors lack significant toroidal momentum sources, the assumption that
. Given that the two planned tokamak
fusion reactors lack significant toroidal momentum sources, the assumption that
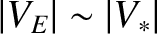 in tokamak
fusion reactors is reasonable, and will adopted throughout the remainder of this book.
in tokamak
fusion reactors is reasonable, and will adopted throughout the remainder of this book.