Potential Formulation of Maxwell's Equations
We saw in Section 2.3.2 that the second and third Maxwell equations, (2.485) and (2.486), are automatically satisfied
if we write the electric and magnetic fields in terms of scalar and vector potentials; that is,
As was discussed in Section 2.3.3, this prescription is not unique, but we can make it unique by adopting the
following conventions:
The previous equation is known as the Lorenz gauge.
The previous equation can be combined with Equation (2.495) and the first Maxwell equation, (2.484), to give
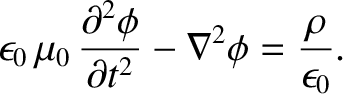 |
(2.499) |
Let us now consider the fourth Maxwell equation, (2.487). Substitution of Equations (2.495) and (2.496) into this equation
yields
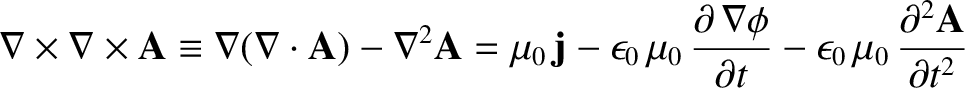 |
(2.500) |
(see Section A.24),
or
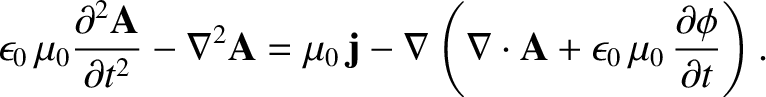 |
(2.501) |
We can now see quite clearly
where the Lorenz gauge, (2.498), comes from. The previous
equation is, in general, very complicated, because it involves both the vector and
scalar potentials. However, if we adopt the Lorenz gauge then the last term on
the right-hand side becomes zero, and the equation simplifies considerably, and ends up
only involving the vector potential. Thus, we find that Maxwell's equations
reduce to the following equations:
Of course, this is the same (scalar) equation written four times over. In a non-time-varying situation (i.e.,
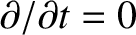 ), the equation in question reduces to Poisson's equation (see Section 2.1.9), which we know
how to solve. With the
), the equation in question reduces to Poisson's equation (see Section 2.1.9), which we know
how to solve. With the
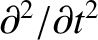 terms included,
the equation becomes a slightly more complicated equation (in fact, it is an inhomogeneous three-dimensional wave equation).
terms included,
the equation becomes a slightly more complicated equation (in fact, it is an inhomogeneous three-dimensional wave equation).
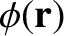
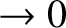
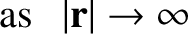

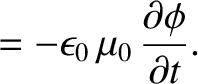
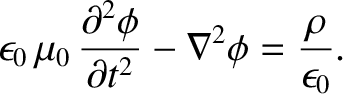
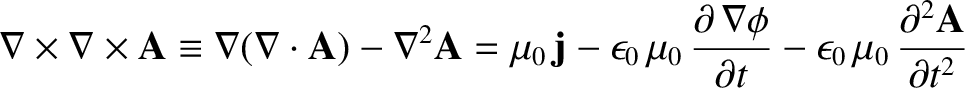
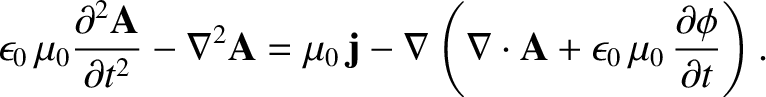
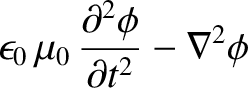
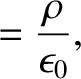
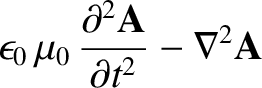
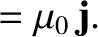
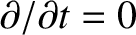 ), the equation in question reduces to Poisson's equation (see Section 2.1.9), which we know
how to solve. With the
), the equation in question reduces to Poisson's equation (see Section 2.1.9), which we know
how to solve. With the
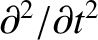 terms included,
the equation becomes a slightly more complicated equation (in fact, it is an inhomogeneous three-dimensional wave equation).
terms included,
the equation becomes a slightly more complicated equation (in fact, it is an inhomogeneous three-dimensional wave equation).