Solution in Presence of External Magnetic Field-Coil

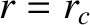Suppose that the perfectly conducting wall at is replaced by a radially-thin, magnetic field-coil that carries a helical current possessing periods in the poloidal direction, and periods in the toroidal direction. Let the current density in the field-coil take the form

$\displaystyle \delta\! j_r$	$\displaystyle =0,$	(3.109)
$\displaystyle \delta \! j_\theta$	$\displaystyle = \frac{n\,\epsilon_c}{m}\,\frac{I_c(t)}{r_c}\,\delta(r-r_c),$	(3.110)
$\displaystyle \delta \! j_z$	$\displaystyle = \frac{I_c(t)}{r_c}\,\delta(r-r_c),$	(3.111)

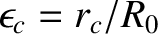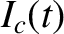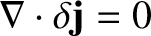where $\epsilon_c=r_c/R_0$ . Here, the complex quantity specifies the amplitude and phase of the helical current flowing in the field-coil. Note that $\nabla\cdot\delta{\bf j}=0$ , as is required by charge conservation.

The most general solution to the cylindrical tearing mode equation, (3.60), in the outer region can now be written

$\displaystyle \delta\psi(r,t) = {\mit\Psi}_s(t)\,\hat{\psi}_s(r) + {\mit\Psi}_w(t)\,\hat{\psi}_w(r) + {\mit\Psi}_c(t)\,\hat{\psi}_c(r),$

(3.112)

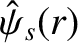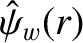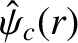where the real functions $\hat{\psi}_s(r)$ and $\hat{\psi}_w(r)$ are specified in Sections 3.8 and 3.9, respectively. Moreover, the real function $\hat{\psi}_c(r)$ is a solution of

$\displaystyle \frac{d^2\hat{\psi}_c}{dr^2} + \frac{1}{r}\,\frac{d\hat{\psi}_c}{dr}-\frac{m^2}{r^2}\,\hat{\psi}_c= 0$

(3.113)

that satisfies

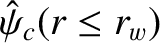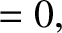$\displaystyle \hat{\psi}_c(r\leq r_w)$	$\displaystyle =0,$	(3.114)
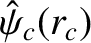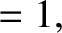$\displaystyle \hat{\psi}_c(r_c)$	$\displaystyle =1,$	(3.115)
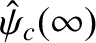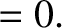$\displaystyle \hat{\psi}_c(\infty)$	$\displaystyle = 0.$	(3.116)

It is easily seen that

$\displaystyle \hat{\psi}_c(r\leq r_w)$	$\displaystyle =0,$	(3.117)
$\displaystyle \hat{\psi}_c(r_w<r \leq r_c)$	$\displaystyle = \frac{(r/r_w)^m-(r/r_w)^{-m}}{(r_c/r_w)^m - (r_c/r_w)^{-m}},$	(3.118)
$\displaystyle \hat{\psi}_c(r> r_c)$	$\displaystyle =\left(\frac{r}{r_c}\right)^{-m}.$	(3.119)

In general, 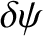$\delta\psi$ is continuous across the field-coil (in accordance with Maxwell's equations), whereas $\partial\delta\psi/\partial r$ is discontinuous. The discontinuity in $\partial\delta\psi/\partial r$ is caused by the helical current flowing in the field-coil. The complex quantity

$\displaystyle {\mit\Psi}_c(t)=\delta\psi(r_c,t)$

(3.120)

determines the amplitude and phase of the perturbed magnetic flux at the field-coil. The complex quantity

$\displaystyle {\mit\Delta\Psi}_c(t) = \left[r\,\frac{\partial\delta\psi}{\partial r}\right]_{r_{c-}}^{r_{c+}}$

(3.121)

parameterizes the amplitude and phase of the helical current sheet flowing in the field-coil. It follows from Equations (3.37), (3.38), (3.110), and (3.111) that

$\displaystyle {\mit\Delta\Psi}_c = -\mu_0\,I_c.$

(3.122)

Simultaneously matching the outer solution, (3.112), across the rational surface, the resistive wall, and the field-coil, we obtain

$\displaystyle {\mit\Delta\Psi}_s$	$\displaystyle = E_{ss}\,{\mit\Psi}_s + E_{sw}\,{\mit\Psi}_w,$	(3.123)
$\displaystyle {\mit\Delta\Psi}_w$	$\displaystyle = E_{ws}\,{\mit\Psi}_s + E_{ww}\,{\mit\Psi}_w + E_{wc}\,{\mit\Psi}_c,$	(3.124)
$\displaystyle {\mit\Delta\Psi}_c$	$\displaystyle = E_{cw}\,{\mit\Psi}_w + E_{cc}\,{\mit\Psi}_c.$	(3.125)

Here,

$\displaystyle E_{cc}$	$\displaystyle = \left[r\,\frac{d\hat{\psi}_c}{dr}\right]_{r_{c-}}^{r_{c+}}=- \frac{2m}{1-(r_w/r_c)^{2m}},$	(3.126)
$\displaystyle E_{wc}$	$\displaystyle = \left[r\,\frac{d\hat{\psi}_c}{dr}\right]_{r_{w+}} = \frac{2m\,(r_w/r_c)^m}{1-(r_w/r_c)^{2m}},$	(3.127)
$\displaystyle E_{cw}$	$\displaystyle =- \left[r\,\frac{d\hat{\psi}_w}{dr}\right]_{r_{c-}} = \frac{2m\,(r_w/r_c)^m}{1-(r_w/r_c)^{2m}},$	(3.128)

where use has been made of Equations (3.81), (3.118), and (3.119).