Next: Constant- Magnetic Island Evolution Up: Magnetic Reconnection Previous: Resistive Kink Modes Contents
 Magnetic Islands
Magnetic Islands
![\includegraphics[height=3.75in]{Chapter09/fig9_5.eps}](img3451.png) |
Suppose that the constant- approximation is valid. According to Section 9.5, this implies that
the perturbed magnetic flux,
approximation is valid. According to Section 9.5, this implies that
the perturbed magnetic flux, 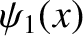 , is approximately constant in the vicinity of the resonant
layer. Let
, is approximately constant in the vicinity of the resonant
layer. Let
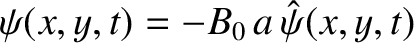 and
and
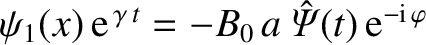 , where
, where
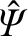 is real and positive, and
is real and positive, and 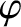 is real. The physical
magnetic flux, which is the real part of
Equation (9.18), reduces to
is real. The physical
magnetic flux, which is the real part of
Equation (9.18), reduces to
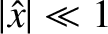 ,
where
,
where
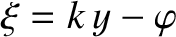 .
Let
Equation (9.83) yields
.
Let
Equation (9.83) yields
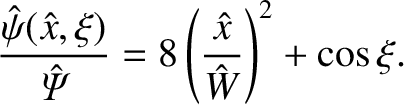 |
(9.85) |
Figure 9.5 shows the contours of
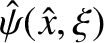 specified in the previous equation. Recall that the
contours of
specified in the previous equation. Recall that the
contours of
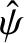 correspond to magnetic field-lines. It can be seen that the tearing mode has changed the
topology of the magnetic field in the immediate vicinity of the resonant surface,
correspond to magnetic field-lines. It can be seen that the tearing mode has changed the
topology of the magnetic field in the immediate vicinity of the resonant surface,  . In fact, as the
tearing mode grows in amplitude (i.e., as
. In fact, as the
tearing mode grows in amplitude (i.e., as
 increases), magnetic field-lines pass through
the magnetic “X-points” (which are located at
increases), magnetic field-lines pass through
the magnetic “X-points” (which are located at 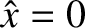 ,
,
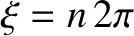 , where
, where 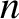 is an integer), at which time they break (or “tear”) and then reconnect to form new field-lines that do not extend over all values of
is an integer), at which time they break (or “tear”) and then reconnect to form new field-lines that do not extend over all values of 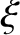 . The magnetic field-line
that forms the boundary between the unreconnected and reconnected regions is known as the magnetic
separatrix, and corresponds to the contour
. The magnetic field-line
that forms the boundary between the unreconnected and reconnected regions is known as the magnetic
separatrix, and corresponds to the contour
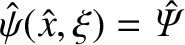 . The reconnected regions within the
magnetic separatrix are termed magnetic islands. The full width (in
. The reconnected regions within the
magnetic separatrix are termed magnetic islands. The full width (in 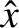 ) of the magnetic
separatrix, which is known as the magnetic island width, is
) of the magnetic
separatrix, which is known as the magnetic island width, is 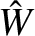 . It can be seen
from Equation (9.84) that the magnetic island width is proportional to the square-root of the quantity
. It can be seen
from Equation (9.84) that the magnetic island width is proportional to the square-root of the quantity
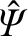 , which is termed the (normalized) reconnected magnetic flux. (In fact, the magnetic
flux, per unit length in the
, which is termed the (normalized) reconnected magnetic flux. (In fact, the magnetic
flux, per unit length in the 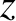 -direction, that passes through a surface (whose normal lies in the
-direction, that passes through a surface (whose normal lies in the 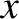 -
-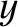 plane) linking the center of a magnetic
island to the separatrix is
plane) linking the center of a magnetic
island to the separatrix is
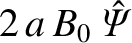 .)
.)
Consider the term
![$[\phi,\psi]$](img3467.png) , appearing in the reduced-MHD Ohm's law, (9.11). With
, appearing in the reduced-MHD Ohm's law, (9.11). With
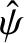 specified
by Equation (9.83), the term in question reduces to
specified
by Equation (9.83), the term in question reduces to
![$\displaystyle [\phi,\psi] = B_0\,k\,\hat{x}\,\frac{\partial\phi}{\partial\xi} + B_0\,k\,\frac{\partial\phi}{\partial \hat{x}}\,\skew{3}\hat{\mit\Psi}\,\sin\xi.$](img3468.png) |
(9.86) |
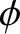 and
and
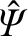 ), whereas the second is nonlinear (i.e., it is second order in perturbed quantities). Thus, linear layer
theory is only valid when the second term is negligible with respect to the first. Estimating both
), whereas the second is nonlinear (i.e., it is second order in perturbed quantities). Thus, linear layer
theory is only valid when the second term is negligible with respect to the first. Estimating both
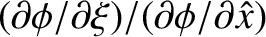 and
and 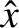 as
as
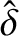 , where
, where
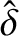 is the normalized constant-
is the normalized constant- linear layer width, and recalling that
linear layer width, and recalling that
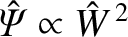 , the criterion for the validity of
linear layer theory becomes
, the criterion for the validity of
linear layer theory becomes
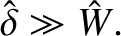 |
(9.87) |
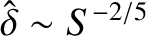 ), this implies that
linear layer theory breaks down before the tearing mode has significantly modified the topology of the magnetic field.
), this implies that
linear layer theory breaks down before the tearing mode has significantly modified the topology of the magnetic field.