


Next: Exercises
Up: Heat and Work
Previous: Quasi-Static Processes
Exact and Inexact Differentials
In our investigation of heat and work, we have come across
various infinitesimal objects, such as
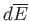 and
and
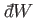 .
It is instructive to examine these infinitesimals more closely.
.
It is instructive to examine these infinitesimals more closely.
Consider the purely mathematical problem where 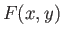 is some general
function of two
independent variables,
is some general
function of two
independent variables,  and
and  . Consider the change in
. Consider the change in 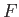 in
going from the point
in
going from the point 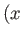 ,
, 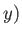 in the
in the  -
- plane to the neighboring point
(
plane to the neighboring point
(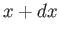 ,
, 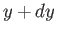 ). This is given by
). This is given by
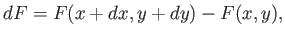 |
(4.22) |
which can also be written
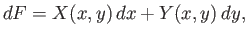 |
(4.23) |
where
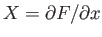 and
and
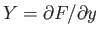 . Clearly,
. Clearly, 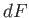 is
simply the infinitesimal difference between two adjacent values of the function
is
simply the infinitesimal difference between two adjacent values of the function 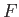 .
This type of infinitesimal quantity is termed an exact differential to
distinguish it from another type to be discussed presently.
If we move in the
.
This type of infinitesimal quantity is termed an exact differential to
distinguish it from another type to be discussed presently.
If we move in the  -
- plane from an initial point
plane from an initial point
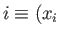 ,
, 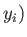 to a final point
to a final point
 ,
, 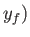 then the corresponding change in
then the corresponding change in 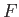 is
given by
is
given by
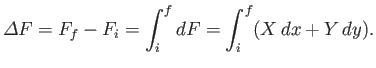 |
(4.24) |
Note that because the difference on the left-hand side depends only on the initial
and final points, the integral on the right-hand side can only depend on
these points as well.
In other words, the value of the
integral is independent of the path taken in going from the initial to the final
point. This is the distinguishing feature of an exact differential.
Consider an integral taken around a closed circuit in the  -
- plane. In this
case, the initial and final points correspond to the same point, so the difference
plane. In this
case, the initial and final points correspond to the same point, so the difference
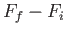 is clearly zero. It follows that the integral of an exact differential
over a closed circuit is always zero:
is clearly zero. It follows that the integral of an exact differential
over a closed circuit is always zero:
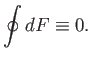 |
(4.25) |
Of course, not every infinitesimal quantity is an exact differential. Consider
the infinitesimal object
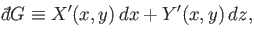 |
(4.26) |
where 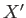 and
and 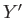 are two
general functions of
are two
general functions of  and
and  . It is easy to test whether or not
an infinitesimal quantity is an exact differential. Consider the expression
(4.23).
It is clear that because
. It is easy to test whether or not
an infinitesimal quantity is an exact differential. Consider the expression
(4.23).
It is clear that because
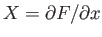 and
and
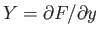 then
then
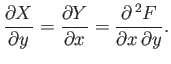 |
(4.27) |
Thus,
if
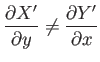 |
(4.28) |
(as is assumed to be the case), then
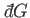 cannot be an exact differential, and
is instead termed an inexact differential.
The special symbol
cannot be an exact differential, and
is instead termed an inexact differential.
The special symbol
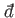 is used to denote an inexact differential.
Consider the integral of
is used to denote an inexact differential.
Consider the integral of
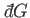 over some path in the
over some path in the  -
- plane. In general, it is not true
that
plane. In general, it is not true
that
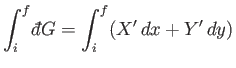 |
(4.29) |
is independent of the path taken between the initial and final points.
This is the distinguishing feature of an inexact differential. In particular,
the integral of an inexact differential around a closed circuit is not necessarily
zero, so
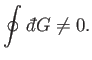 |
(4.30) |
Consider, for the moment, the solution of
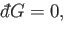 |
(4.31) |
which reduces to the ordinary differential equation
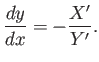 |
(4.32) |
Because the right-hand side is a known function of  and
and  , the previous
equation defines
a definite direction (i.e., gradient) at each point in the
, the previous
equation defines
a definite direction (i.e., gradient) at each point in the  -
- plane. The
solution simply consists of drawing a system of curves in the
plane. The
solution simply consists of drawing a system of curves in the
 -
- plane such that, at any point, the tangent to the curve is as specified
by Equation (4.32). This defines a set of curves that can be written
plane such that, at any point, the tangent to the curve is as specified
by Equation (4.32). This defines a set of curves that can be written
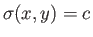 , where
, where 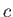 is a labeling parameter.
It follows that, on a particular curve,
is a labeling parameter.
It follows that, on a particular curve,
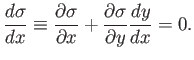 |
(4.33) |
The elimination of 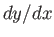 between Equations (4.32) and (4.33) yields
between Equations (4.32) and (4.33) yields
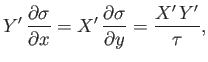 |
(4.34) |
where
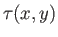 is function of
is function of  and
and  . The previous equation could equally
well be written
. The previous equation could equally
well be written
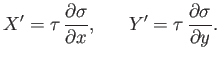 |
(4.35) |
Inserting Equation (4.35) into Equation (4.26) gives
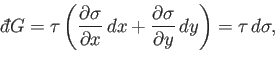 |
(4.36) |
or
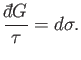 |
(4.37) |
Thus, dividing the inexact differential
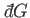 by
by 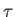 yields the exact
differential
yields the exact
differential 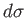 . A factor
. A factor 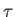 that possesses this property is
termed an integrating factor. Because the previous analysis is quite general,
it is clear that an inexact differential involving two independent variables always
admits of an integrating factor. Note, however,
this is not generally the case for inexact
differentials involving more than two variables.
that possesses this property is
termed an integrating factor. Because the previous analysis is quite general,
it is clear that an inexact differential involving two independent variables always
admits of an integrating factor. Note, however,
this is not generally the case for inexact
differentials involving more than two variables.
After this mathematical excursion, let us return to a physical situation of interest.
The macrostate of a macroscopic system can be specified by the values of the
external parameters (e.g., the volume), and the mean energy,
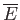 . This, in
turn, fixes other parameters, such as the mean pressure,
. This, in
turn, fixes other parameters, such as the mean pressure, 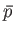 . Alternatively,
we can specify the external parameters and the mean pressure, which fixes the
mean energy. Quantities such as
. Alternatively,
we can specify the external parameters and the mean pressure, which fixes the
mean energy. Quantities such as 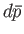 and
and
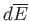 are infinitesimal
differences between well-defined quantities: that is,
they are exact differentials.
For example,
are infinitesimal
differences between well-defined quantities: that is,
they are exact differentials.
For example,
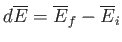 is just the difference between the
mean energy of the system in the final macrostate
is just the difference between the
mean energy of the system in the final macrostate 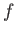 and the
initial macrostate
and the
initial macrostate 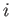 , in the limit where these two states are nearly the same.
It follows that if the system is taken from an initial macrostate
, in the limit where these two states are nearly the same.
It follows that if the system is taken from an initial macrostate 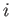 to any
final macrostate
to any
final macrostate 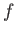 then the mean energy change is given by
then the mean energy change is given by
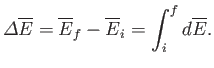 |
(4.38) |
However, because the mean energy is just a function of the macrostate under consideration,
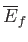 and
and
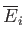 depend only on the initial and final
states, respectively.
Thus, the integral
depend only on the initial and final
states, respectively.
Thus, the integral
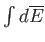 depends only on the initial and final
states, and not on the particular process used to get between them.
depends only on the initial and final
states, and not on the particular process used to get between them.
Consider, now, the infinitesimal work done by the system in going from some
initial macrostate 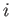 to some neighbouring final macrostate
to some neighbouring final macrostate 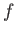 . In general,
. In general,
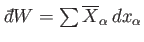 is not the difference between two
numbers referring to the properties of two neighboring macrostates. Instead,
it is merely an infinitesimal quantity characteristic of the process of going
from state
is not the difference between two
numbers referring to the properties of two neighboring macrostates. Instead,
it is merely an infinitesimal quantity characteristic of the process of going
from state 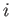 to state
to state 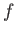 . In other words, the work
. In other words, the work
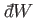 is in general
an inexact differential. The total work done by the system in going from any
macrostate
is in general
an inexact differential. The total work done by the system in going from any
macrostate 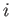 to some other macrostate
to some other macrostate 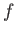 can be written as
can be written as
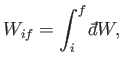 |
(4.39) |
where the integral represents the sum of the infinitesimal amounts of work
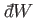 performed at each stage of the process. In general, the value of
the integral does
depend on the particular process used in going from macrostate
performed at each stage of the process. In general, the value of
the integral does
depend on the particular process used in going from macrostate 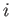 to macrostate
to macrostate 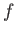 .
.
Recall that, in going from macrostate 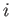 to macrostate
to macrostate 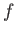 , the change in energy,
, the change in energy,
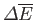 ,
does not
depend on the process used, whereas the work done,
,
does not
depend on the process used, whereas the work done, 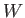 , in general, does.
Thus, it follows from
the first law of thermodynamics, Equation (4.10),
that the heat absorbed,
, in general, does.
Thus, it follows from
the first law of thermodynamics, Equation (4.10),
that the heat absorbed, 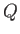 , in general, also depends on the
process used. It follows that
, in general, also depends on the
process used. It follows that
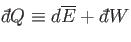 |
(4.40) |
is an inexact differential. However, by analogy with the mathematical example
discussed previously, there must exist some integrating factor,  (say), that
converts the inexact differential
(say), that
converts the inexact differential
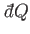 into an exact differential.
So,
into an exact differential.
So,
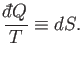 |
(4.41) |
It will be interesting to discover the physical quantities that correspond to
the functions  and
and  . (See Section 5.5.)
. (See Section 5.5.)
Suppose that the system is thermally insulated, so that
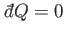 . In this case, the
first law of thermodynamics implies that
. In this case, the
first law of thermodynamics implies that
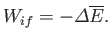 |
(4.42) |
Thus, in this special case, the work done depends only on the energy
difference between the initial and final states, and is independent of the process.
In fact, when Clausius first formulated the first law of thermodynamics, in 1850,
he wrote:
If a thermally isolated system is brought from some initial to some final state
then the work done by the system is independent of the process used.
If the external parameters of the system are kept fixed, so that no work is done,
then
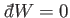 , Equation (4.11) reduces
to
, Equation (4.11) reduces
to
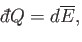 |
(4.43) |
and
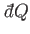 becomes an exact differential. The amount of heat,
becomes an exact differential. The amount of heat, 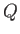 , absorbed
in going from one macrostate to another
depends only on the mean energy difference between them, and
is independent of the process used to effect the change. In this situation,
heat is a conserved quantity, and acts very much like the invisible indestructible
fluid of Lavoisier's calorific theory.
, absorbed
in going from one macrostate to another
depends only on the mean energy difference between them, and
is independent of the process used to effect the change. In this situation,
heat is a conserved quantity, and acts very much like the invisible indestructible
fluid of Lavoisier's calorific theory.



Next: Exercises
Up: Heat and Work
Previous: Quasi-Static Processes
Richard Fitzpatrick
2016-01-25
![]() is some general
function of two
independent variables,
is some general
function of two
independent variables, ![]() and
and ![]() . Consider the change in
. Consider the change in ![]() in
going from the point
in
going from the point ![]() ,
, ![]() in the
in the ![]() -
-![]() plane to the neighboring point
(
plane to the neighboring point
(![]() ,
, ![]() ). This is given by
). This is given by
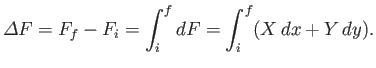
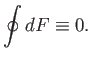
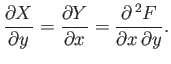
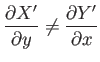
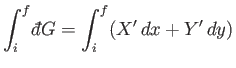
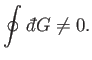
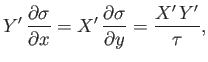
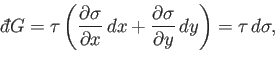
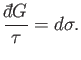
![]() . This, in
turn, fixes other parameters, such as the mean pressure,
. This, in
turn, fixes other parameters, such as the mean pressure, ![]() . Alternatively,
we can specify the external parameters and the mean pressure, which fixes the
mean energy. Quantities such as
. Alternatively,
we can specify the external parameters and the mean pressure, which fixes the
mean energy. Quantities such as ![]() and
and
![]() are infinitesimal
differences between well-defined quantities: that is,
they are exact differentials.
For example,
are infinitesimal
differences between well-defined quantities: that is,
they are exact differentials.
For example,
![]() is just the difference between the
mean energy of the system in the final macrostate
is just the difference between the
mean energy of the system in the final macrostate ![]() and the
initial macrostate
and the
initial macrostate ![]() , in the limit where these two states are nearly the same.
It follows that if the system is taken from an initial macrostate
, in the limit where these two states are nearly the same.
It follows that if the system is taken from an initial macrostate ![]() to any
final macrostate
to any
final macrostate ![]() then the mean energy change is given by
then the mean energy change is given by
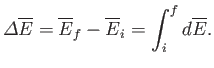
![]() to some neighbouring final macrostate
to some neighbouring final macrostate ![]() . In general,
. In general,
![]() is not the difference between two
numbers referring to the properties of two neighboring macrostates. Instead,
it is merely an infinitesimal quantity characteristic of the process of going
from state
is not the difference between two
numbers referring to the properties of two neighboring macrostates. Instead,
it is merely an infinitesimal quantity characteristic of the process of going
from state ![]() to state
to state ![]() . In other words, the work
. In other words, the work
![]() is in general
an inexact differential. The total work done by the system in going from any
macrostate
is in general
an inexact differential. The total work done by the system in going from any
macrostate ![]() to some other macrostate
to some other macrostate ![]() can be written as
can be written as
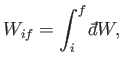
![]() to macrostate
to macrostate ![]() , the change in energy,
, the change in energy,
![]() ,
does not
depend on the process used, whereas the work done,
,
does not
depend on the process used, whereas the work done, ![]() , in general, does.
Thus, it follows from
the first law of thermodynamics, Equation (4.10),
that the heat absorbed,
, in general, does.
Thus, it follows from
the first law of thermodynamics, Equation (4.10),
that the heat absorbed, ![]() , in general, also depends on the
process used. It follows that
, in general, also depends on the
process used. It follows that
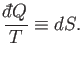
![]() . In this case, the
first law of thermodynamics implies that
. In this case, the
first law of thermodynamics implies that
![]() , Equation (4.11) reduces
to
, Equation (4.11) reduces
to