Next: Partial Pressure Up: Kinetic Theory Previous: Pressure
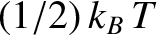 , where
, where  is the temperature of the system. (See Section 5.5.5.) It turns out, however, that this law only applies if the contribution in
question is governed by classical (as opposed to quantum mechanical) physics. (See Section 5.5.6.)
is the temperature of the system. (See Section 5.5.5.) It turns out, however, that this law only applies if the contribution in
question is governed by classical (as opposed to quantum mechanical) physics. (See Section 5.5.6.)
Consider a particular constituent molecule of an ideal gas whose mass is  , and whose velocity is
, and whose velocity is  . The contribution of molecule's
translational kinetic energy to the total energy of the whole gas is
. The contribution of molecule's
translational kinetic energy to the total energy of the whole gas is
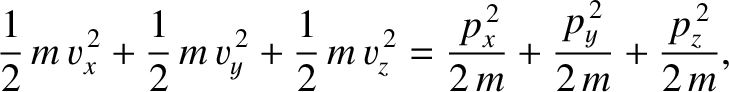 |
(5.181) |
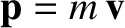 is the molecular momentum. It can be seen that the contribution of the molecules's translational
kinetic energy to the total energy consists of three terms that are quadratic in a momentum component. Hence,
according to the law of equipartition of energy, the mean thermal energy associated with the
molecules translational kinetic energy is
is the molecular momentum. It can be seen that the contribution of the molecules's translational
kinetic energy to the total energy consists of three terms that are quadratic in a momentum component. Hence,
according to the law of equipartition of energy, the mean thermal energy associated with the
molecules translational kinetic energy is
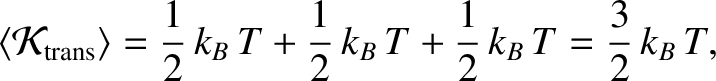 |
(5.182) |