Next: Particle in Finite Square Up: One-Dimensional Wave Mechanics Previous: One-Dimensional Wave Mechanics
![\begin{displaymath}U(x) = \left\{
\begin{array}{lll}
0&\mbox{\hspace{0.5cm}}&0\l...
... \leq a\\ [0.5ex]
\infty &&\mbox{otherwise}
\end{array}\right..\end{displaymath}](img3021.png) |
(4.72) |
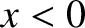 or
or 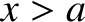 , so
, so 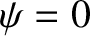 in this region (i.e., there
is zero probability of finding the particle outside the well). Within the well, a particle
of definite energy
in this region (i.e., there
is zero probability of finding the particle outside the well). Within the well, a particle
of definite energy  has a stationary wavefunction,
has a stationary wavefunction, 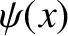 , that satisfies
[See Equation (4.71).]
The boundary conditions are
This follows because
, that satisfies
[See Equation (4.71).]
The boundary conditions are
This follows because 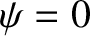 in the region
in the region 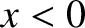 or
or 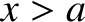 , and
, and 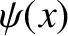 must be continuous [because a discontinuous
wavefunction would generate a singular term (i.e., the term involving
must be continuous [because a discontinuous
wavefunction would generate a singular term (i.e., the term involving
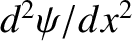 ) in the time-independent Schrödinger equation, (4.71),
that could not be balanced, even by an infinite potential].
) in the time-independent Schrödinger equation, (4.71),
that could not be balanced, even by an infinite potential].
Let us search for solutions to Equation (4.73) of the form
where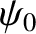 is a constant. It follows that
The solution (4.75) automatically satisfies the boundary condition
is a constant. It follows that
The solution (4.75) automatically satisfies the boundary condition 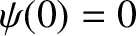 . The second boundary
condition,
. The second boundary
condition, 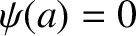 , leads to a quantization of the wavenumber; that is,
where
, leads to a quantization of the wavenumber; that is,
where
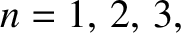 et cetera. (A “quantized” quantity is one that can only take certain discrete values.) According to Equation (4.76), the energy is also quantized.
In fact,
et cetera. (A “quantized” quantity is one that can only take certain discrete values.) According to Equation (4.76), the energy is also quantized.
In fact, 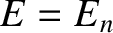 , where
Thus, the allowed wavefunctions for a particle trapped in a one-dimensional square potential well of infinite depth are
where
, where
Thus, the allowed wavefunctions for a particle trapped in a one-dimensional square potential well of infinite depth are
where  is a positive integer, and
is a positive integer, and 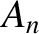 a constant. We cannot have
a constant. We cannot have 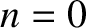 , because, in this case, we obtain
a null wavefunction—that is,
, because, in this case, we obtain
a null wavefunction—that is, 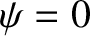 , everywhere—which corresponds to a non-existent state. Furthermore, if
, everywhere—which corresponds to a non-existent state. Furthermore, if  takes a negative integer value
then it generates exactly the same wavefunction as the corresponding positive integer value (assuming
takes a negative integer value
then it generates exactly the same wavefunction as the corresponding positive integer value (assuming
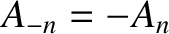 ).
).
The constant 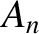 , appearing in the previous wavefunction, can be determined from the constraint that the
wavefunction be properly normalized. For the case under consideration, the normalization condition (4.27)
reduces to
, appearing in the previous wavefunction, can be determined from the constraint that the
wavefunction be properly normalized. For the case under consideration, the normalization condition (4.27)
reduces to
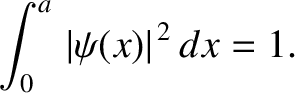 |
(4.80) |
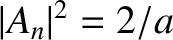 . Hence, the properly normalized version of the wavefunction (4.79)
is
Figure 4.4 shows the first four properly normalized stationary wavefunctions for a particle trapped in a one-dimensional
square potential well of infinite depth; that is,
. Hence, the properly normalized version of the wavefunction (4.79)
is
Figure 4.4 shows the first four properly normalized stationary wavefunctions for a particle trapped in a one-dimensional
square potential well of infinite depth; that is,
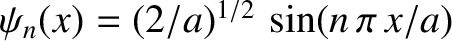 ,
for
,
for 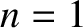 to
to 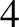 .
.
![\includegraphics[width=0.9\textwidth]{Chapter05/fig11_05.eps}](img3046.png) |
At first sight, it seems rather strange that the lowest possible energy for a particle trapped in a one-dimensional
potential well is not zero, as would be the case in classical mechanics, but rather
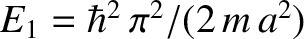 . In fact,
as explained in the following, this residual energy is a direct consequence of Heisenberg's uncertainty principle. A
particle trapped in a one-dimensional well of width
. In fact,
as explained in the following, this residual energy is a direct consequence of Heisenberg's uncertainty principle. A
particle trapped in a one-dimensional well of width  is likely to be found
anywhere inside the well. Thus, the uncertainty in the particle's position is
is likely to be found
anywhere inside the well. Thus, the uncertainty in the particle's position is
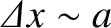 . It
follows from the uncertainty principle, (4.65), that
. It
follows from the uncertainty principle, (4.65), that
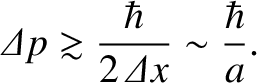 |
(4.82) |
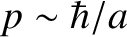 .
However, for a free particle,
.
However, for a free particle,
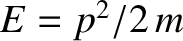 . Hence, the residual energy associated with the
particle's residual momentum is
. Hence, the residual energy associated with the
particle's residual momentum is
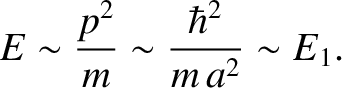 |
(4.83) |
The most general wavefunction for a particle trapped in a one-dimensional square potential well, of infinite depth, is a superposition of all of the possible stationary states. That is,
where the are complex numbers, and the
are complex numbers, and the
 are specified in Equation (4.81).
Consider
are specified in Equation (4.81).
Consider
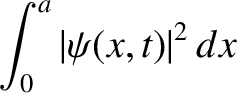 |
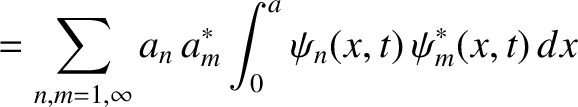 |
|
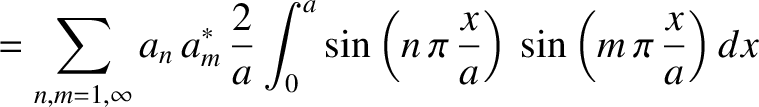 |
||
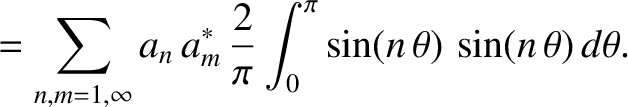 |
(4.85) |
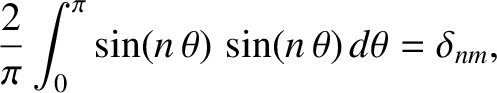 |
(4.86) |
 , which is known as a Kronecker delta, takes the value 1 if
, which is known as a Kronecker delta, takes the value 1 if 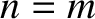 , and 0 otherwise.
Hence, we deduce that
, and 0 otherwise.
Hence, we deduce that
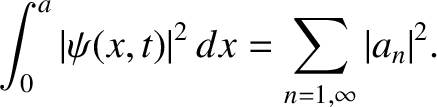 |
(4.87) |
Suppose that we make a measurement of the energy of a particle whose wavefunction is specified by Equation (4.84). Given that the wavefunction is a superposition of stationary states associated
with the quantized energies 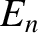 [see Equation (4.78)], it seems reasonable to assume that the
measurement will result in one of these energies. In fact, according to quantum mechanics, the
probability that a measurement of the particle's energy will give the result
[see Equation (4.78)], it seems reasonable to assume that the
measurement will result in one of these energies. In fact, according to quantum mechanics, the
probability that a measurement of the particle's energy will give the result 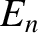 is
is 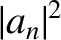 . Thus, we
can see that the normalization condition (4.88) ensures that the sum of all of these probabilities
is unity. This must be the case, because a measurement of the particle's energy is certain to give one of the
allowed energies. Suppose that we make a measurement of the particle's energy, and obtain the result
. Thus, we
can see that the normalization condition (4.88) ensures that the sum of all of these probabilities
is unity. This must be the case, because a measurement of the particle's energy is certain to give one of the
allowed energies. Suppose that we make a measurement of the particle's energy, and obtain the result 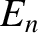 .
A second measurement, made immediately after the first, must yield the same result. In other words, immediately
after the first measurement, the particle's wavefunction must be such that a measurement of its energy
is certain to give the result
.
A second measurement, made immediately after the first, must yield the same result. In other words, immediately
after the first measurement, the particle's wavefunction must be such that a measurement of its energy
is certain to give the result 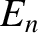 , and has no chance of giving the result
, and has no chance of giving the result 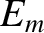 , where
, where 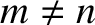 . This
implies that
. This
implies that
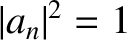 and
and 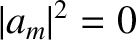 , where
, where 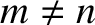 . We conclude that, after the first
measurement, the particle's wavefunction is
. We conclude that, after the first
measurement, the particle's wavefunction is
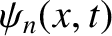 . This is another example of the collapse of a wavefunction consequent on a measurement. (See Section 4.2.8.)
. This is another example of the collapse of a wavefunction consequent on a measurement. (See Section 4.2.8.)