Next: Aberration of Starlight and Up: Experimental Basis of Special Previous: Sound Waves in a
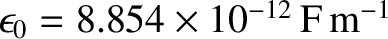 is the electrical permittivity of free space, and
is the electrical permittivity of free space, and
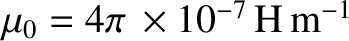 the magnetic permeability of free space. (See Section 2.4.4.) It follows that
the magnetic permeability of free space. (See Section 2.4.4.) It follows that
![$\displaystyle c = \frac{1}{[(8.854\times 10^{-12})\,(4\pi\,\times 10^{-7})]^{1/2}}= 2.998\times 10^8\,{\rm m\,s^{-1}}.$](img2250.png) |
(3.11) |
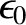 and
and 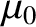 can be determined from simple experiments involving measurements of the
forces exerted by electric charges and current loops on one another.
can be determined from simple experiments involving measurements of the
forces exerted by electric charges and current loops on one another.
The classical theory of electromagnetism (i.e., Maxwell's equations) does not explicitly mention a medium through which
electromagnetic disturbances propagate. (See Section 2.4.2.) Nevertheless, prior to the 20th century, most physicists assumed that
such a medium existed, because they could not conceive of a wave that propagated in the absence of a medium.
The medium in question was known as the aether (from the ancient Greek
![]()
, which is the fifth element
of Aristotelian philosophy), and was thought to permeate all space, including vacuums. Thus, by analogy with a sound wave, the phase velocity of a light wave in a frame of reference moving at fixed velocity  with respect to the
rest frame of the aether was assumed to be
with respect to the
rest frame of the aether was assumed to be
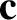 is the phase velocity of the light wave in the rest frame of the aether, and
is the phase velocity of the light wave in the rest frame of the aether, and
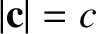 is the speed of light, (3.10).
is the speed of light, (3.10).