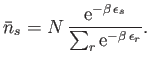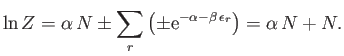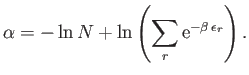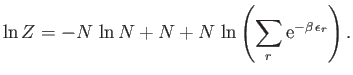Next: Quantum-Mechanical Treatment of Ideal
Up: Quantum Statistics
Previous: Maxwell-Boltzmann Statistics
The preceding analysis regarding the quantum statistics of ideal
gases is summarized in the following statements. The mean number of
particles occupying quantum state 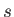 is given by
is given by
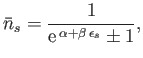 |
(8.56) |
where the upper sign corresponds to Fermi-Dirac statistics and the
lower sign corresponds to Bose-Einstein statistics. The parameter
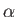 is determined via
is determined via
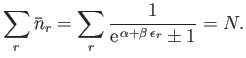 |
(8.57) |
Finally, the partition function of the gas is given by
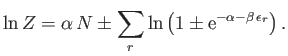 |
(8.58) |
Let us investigate the magnitude of 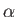 in some important limiting
cases. Consider, first of all, the case of a gas at a given temperature
when its concentration is made sufficiently low: that is, when
in some important limiting
cases. Consider, first of all, the case of a gas at a given temperature
when its concentration is made sufficiently low: that is, when
 is made sufficiently small. The relation (8.57) can only
be satisfied if each term in the sum over states is made
sufficiently small; that is, if
is made sufficiently small. The relation (8.57) can only
be satisfied if each term in the sum over states is made
sufficiently small; that is, if
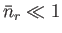 or
or
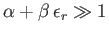 for all states
for all states  . (See Figure 8.1.)
. (See Figure 8.1.)
Consider, next, the case of a gas made up of a fixed number of particles
when its temperature is made sufficiently large. That is, when 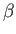 is
made sufficiently small. In the sum in Equation (8.57), the terms of
appreciable magnitude are those for which
is
made sufficiently small. In the sum in Equation (8.57), the terms of
appreciable magnitude are those for which
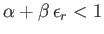 . (See Figure 8.1.)
Thus, it follows that as
. (See Figure 8.1.)
Thus, it follows that as
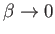 an increasing number of
terms with large values of
an increasing number of
terms with large values of
 contribute substantially to this
sum. In order to prevent the sum from exceeding
contribute substantially to this
sum. In order to prevent the sum from exceeding  , the parameter
, the parameter 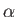 must become large enough that each term is made sufficiently small: that is,
it is again necessary that
must become large enough that each term is made sufficiently small: that is,
it is again necessary that
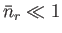 or
or
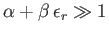 for all states
for all states  .
.
The previous discussion suggests that if the concentration of an ideal
gas is made sufficiently low, or the temperature is made sufficiently high,
then 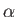 must become so large that
must become so large that
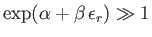 |
(8.59) |
for all  . Equivalently, this means that the number of particles occupying
each quantum state must
become so small that
. Equivalently, this means that the number of particles occupying
each quantum state must
become so small that
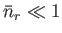 |
(8.60) |
for all  . It is conventional to refer to the limit of sufficiently
low concentration, or sufficiently high temperature, in which Equations (8.59)
and Equations (8.60) are satisfied, as the classical limit.
. It is conventional to refer to the limit of sufficiently
low concentration, or sufficiently high temperature, in which Equations (8.59)
and Equations (8.60) are satisfied, as the classical limit.
According to Equations (8.56) and (8.59), both
the Fermi-Dirac and Bose-Einstein
distributions reduce to
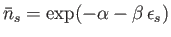 |
(8.61) |
in the classical limit, whereas the constraint (8.57) yields
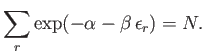 |
(8.62) |
The previous expressions can be combined to give
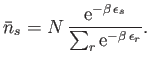 |
(8.63) |
It follows that in the classical limit of sufficiently low density,
or sufficiently high temperature, the quantum distribution functions,
whether Fermi-Dirac or Bose-Einstein, reduce to the Maxwell-Boltzmann
distribution. It is easily demonstrated that the physical criterion for the
validity of the classical approximation is that the mean separation between
particles should be much greater than their mean de Broglie wavelengths. (See Exercise 21.)
Let us now consider the behavior of the partition function (8.58)
in the classical limit. We can expand the logarithm to
give
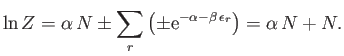 |
(8.64) |
However, according to Equation (8.62),
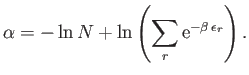 |
(8.65) |
It follows that
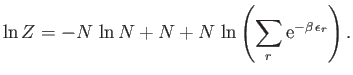 |
(8.66) |
Note that this expression does not match the partition function
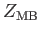 computed in Equation (8.52) from Maxwell-Boltzmann statistics, which gives
computed in Equation (8.52) from Maxwell-Boltzmann statistics, which gives
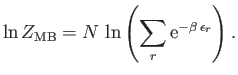 |
(8.67) |
In fact,
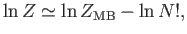 |
(8.68) |
or
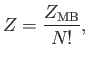 |
(8.69) |
where use has been made of Stirling's approximation (
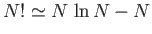 ),
because
),
because  is large. Here, the factor
is large. Here, the factor 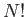 simply corresponds to the number
of different permutations of the
simply corresponds to the number
of different permutations of the  particles: permutations that are
physically meaningless when the particles are identical. Recall, that
we had to introduce precisely this factor, in an ad hoc fashion,
in Section 7.8, in order to avoid the non-physical consequences of
the Gibb's paradox. Clearly, there is no Gibb's paradox when an ideal
gas is treated properly via quantum mechanics.
particles: permutations that are
physically meaningless when the particles are identical. Recall, that
we had to introduce precisely this factor, in an ad hoc fashion,
in Section 7.8, in order to avoid the non-physical consequences of
the Gibb's paradox. Clearly, there is no Gibb's paradox when an ideal
gas is treated properly via quantum mechanics.
A gas in the classical limit, where the typical de Broglie wavelength of the
constituent particles is much smaller than the typical inter-particle
spacing, is said to be non-degenerate. In the opposite limit,
where the concentration and temperature are such that the typical
de Broglie wavelength
becomes comparable with the typical inter-particle spacing, and the actual
Fermi-Dirac or Bose-Einstein distributions must be employed, the
gas is said to be degenerate.



Next: Quantum-Mechanical Treatment of Ideal
Up: Quantum Statistics
Previous: Maxwell-Boltzmann Statistics
Richard Fitzpatrick
2016-01-25
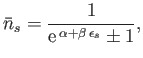
![]() in some important limiting
cases. Consider, first of all, the case of a gas at a given temperature
when its concentration is made sufficiently low: that is, when
in some important limiting
cases. Consider, first of all, the case of a gas at a given temperature
when its concentration is made sufficiently low: that is, when
![]() is made sufficiently small. The relation (8.57) can only
be satisfied if each term in the sum over states is made
sufficiently small; that is, if
is made sufficiently small. The relation (8.57) can only
be satisfied if each term in the sum over states is made
sufficiently small; that is, if
![]() or
or
![]() for all states
for all states ![]() . (See Figure 8.1.)
. (See Figure 8.1.)
![]() is
made sufficiently small. In the sum in Equation (8.57), the terms of
appreciable magnitude are those for which
is
made sufficiently small. In the sum in Equation (8.57), the terms of
appreciable magnitude are those for which
![]() . (See Figure 8.1.)
Thus, it follows that as
. (See Figure 8.1.)
Thus, it follows that as
![]() an increasing number of
terms with large values of
an increasing number of
terms with large values of
![]() contribute substantially to this
sum. In order to prevent the sum from exceeding
contribute substantially to this
sum. In order to prevent the sum from exceeding ![]() , the parameter
, the parameter ![]() must become large enough that each term is made sufficiently small: that is,
it is again necessary that
must become large enough that each term is made sufficiently small: that is,
it is again necessary that
![]() or
or
![]() for all states
for all states ![]() .
.
![]() must become so large that
must become so large that