


Next: Absorption and Stimulated Emission
Up: Time-Dependent Perturbation Theory
Previous: Energy-Shifts and Decay-Widths
Consider a perturbation that oscillates sinusoidally in time.
This is usually called a harmonic perturbation. Thus,
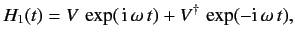 |
(850) |
where 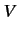 is, in general, a function of position, momentum, and
spin operators.
is, in general, a function of position, momentum, and
spin operators.
Let us initiate the system in the eigenstate 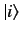 of the unperturbed
Hamiltonian,
of the unperturbed
Hamiltonian, 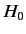 , and switch on the harmonic perturbation at
, and switch on the harmonic perturbation at 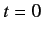 .
It follows from Equation (796) that
.
It follows from Equation (796) that
where
This formula is analogous to Equation (803), provided that
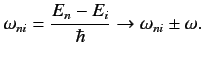 |
(854) |
Thus, it follows from the analysis of Section 8.6 that
the transition probability
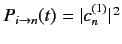 is only appreciable in the limit
is only appreciable in the limit
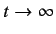 if
if
Clearly, (855) corresponds to the first term on the right-hand side
of Equation (851), and (856) corresponds to the second term. The former
term describes a process by which the system gives up energy
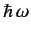 to the perturbing field, while making a transition
to a final state whose energy level is less than that of the initial
state by
to the perturbing field, while making a transition
to a final state whose energy level is less than that of the initial
state by
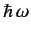 . This process is known as stimulated emission.
The latter term describes a process by which the system gains
energy
. This process is known as stimulated emission.
The latter term describes a process by which the system gains
energy
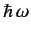 from the perturbing field, while making a transition
to a final state whose energy level exceeds that of the initial
state by
from the perturbing field, while making a transition
to a final state whose energy level exceeds that of the initial
state by
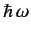 . This process is known as absorption. In
both cases, the total energy (i.e., that of the system plus
the perturbing field) is conserved.
. This process is known as absorption. In
both cases, the total energy (i.e., that of the system plus
the perturbing field) is conserved.
By analogy with Equation (816),
Equation (857) specifies the transition rate for stimulated emission, whereas
Equation (858) gives the transition rate for absorption.
These equations are more usually written
It is clear from Equations (852)-(853) that
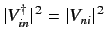 .
It follows from Equations (857)-(858) that
.
It follows from Equations (857)-(858) that
![$\displaystyle \frac{w_{i\rightarrow [n]}}{\rho(E_n)} = \frac{w_{n\rightarrow [i]}}{\rho(E_i)}.$](img2021.png) |
(861) |
In other words, the rate of stimulated emission, divided by the density
of final states for stimulated emission, equals the rate of absorption,
divided
by the density of final states for absorption. This result, which
expresses a fundamental symmetry between absorption and stimulated
emission, is known as detailed balancing, and is very important in
statistical mechanics.



Next: Absorption and Stimulated Emission
Up: Time-Dependent Perturbation Theory
Previous: Energy-Shifts and Decay-Widths
Richard Fitzpatrick
2013-04-08
![]() of the unperturbed
Hamiltonian,
of the unperturbed
Hamiltonian, ![]() , and switch on the harmonic perturbation at
, and switch on the harmonic perturbation at ![]() .
It follows from Equation (796) that
.
It follows from Equation (796) that
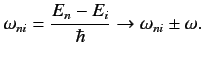
![]() .
It follows from Equations (857)-(858) that
.
It follows from Equations (857)-(858) that
![$\displaystyle \frac{w_{i\rightarrow [n]}}{\rho(E_n)} = \frac{w_{n\rightarrow [i]}}{\rho(E_i)}.$](img2021.png)