


Next: Eigenvalues of Orbital Angular
Up: Orbital Angular Momentum
Previous: Orbital Angular Momentum
Orbital Angular Momentum
Consider a particle described by the Cartesian coordinates
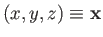 and their conjugate momenta
and their conjugate momenta
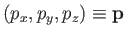 . The classical
definition of the orbital angular momentum of such a particle about the
origin is
. The classical
definition of the orbital angular momentum of such a particle about the
origin is
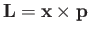 [55], giving
[55], giving
Let us assume that the operators
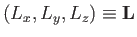 that
represent the components of
orbital angular momentum in quantum mechanics can be defined in
an analogous manner to the corresponding components of
classical angular momentum. In other words, we are
going to assume that the previous equations specify the angular momentum operators
in terms of the position and linear momentum operators. According to Equations (4.1)-(4.3),
that
represent the components of
orbital angular momentum in quantum mechanics can be defined in
an analogous manner to the corresponding components of
classical angular momentum. In other words, we are
going to assume that the previous equations specify the angular momentum operators
in terms of the position and linear momentum operators. According to Equations (4.1)-(4.3), 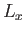 ,
, 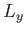 ,
and
,
and 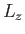 are Hermitian operators, so they represent quantities that can, in principle,
be measured. Note, incidentally, that there is no ambiguity regarding the order
in which operators appear in products on the right-hand sides of Equations (4.1)-(4.3),
because all of the products consist of operators that commute.
are Hermitian operators, so they represent quantities that can, in principle,
be measured. Note, incidentally, that there is no ambiguity regarding the order
in which operators appear in products on the right-hand sides of Equations (4.1)-(4.3),
because all of the products consist of operators that commute.
The fundamental commutation relations satisfied by the
position and linear momentum operators are
where 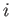 and
and 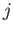 stand for either
stand for either  ,
, 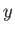 , or
, or  . [See Equations (2.23)-(2.25).]
Consider the commutator of the operators
. [See Equations (2.23)-(2.25).]
Consider the commutator of the operators 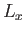 and
and 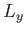 :
:
The cyclic permutations (i.e.,
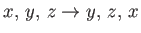 , etc.) of the previous result yield
the fundamental commutation relations satisfied
by the components of an orbital angular momentum:
, etc.) of the previous result yield
the fundamental commutation relations satisfied
by the components of an orbital angular momentum:
These expressions can be summed up more succinctly by writing
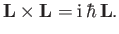 |
(4.11) |
The three commutation relations (4.8)-(4.10) are the foundation for the whole
theory of angular momentum in quantum mechanics. Whenever we encounter
three operators having similar commutation relations, we know the
dynamical variables that they represent have identical properties
to those of the components of an
angular momentum (which we are about to derive). In fact,
we shall assume that any three operators that satisfy the commutation
relations (4.8)-(4.10) represent the components of some sort of angular momentum.
Suppose that there are 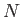 particles in the system, with
angular momentum vectors
particles in the system, with
angular momentum vectors 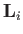 (where
(where 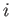 runs from 1 to
runs from 1 to 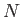 ).
Each of these vectors satisfies Equation (4.11), so that
).
Each of these vectors satisfies Equation (4.11), so that
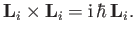 |
(4.12) |
However, we expect the angular momentum operators
belonging to different particles to commute, because they represent different
degrees of freedom of the system. (See Section 2.2.) So,
we can write
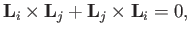 |
(4.13) |
for 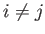 . Consider the total angular momentum of the system,
. Consider the total angular momentum of the system,
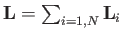 . It is clear from Equations (4.12) and (4.13)
that
. It is clear from Equations (4.12) and (4.13)
that
Thus, the sum of two or more angular momentum vectors satisfies the
same commutation relation as a primitive angular momentum vector.
In particular, the total angular momentum of the system satisfies the
commutation relation (4.11).
The immediate conclusion that can be drawn from the commutation relations
(4.8)-(4.10) is that the three components of an angular momentum vector cannot
be specified (or measured) simultaneously. In fact, once we have specified one
component, the values of other two components become uncertain. It is
conventional to specify the  -component,
-component, 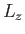 .
.
Consider the magnitude squared of the angular momentum vector,
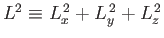 . The commutator of
. The commutator of 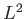 and
and 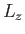 is
written
is
written
![$\displaystyle [L^2, L_z] = [L_x^{\,2}, L_z] + [L_y^{\,2}, L_z] + [L_z^{\,2}, L_z].$](img960.png) |
(4.15) |
It is easily demonstrated that
which implies that
![$\displaystyle [L^2, L_z] = 0.$](img966.png) |
(4.19) |
(See Exercise 1.)
Because there is nothing special about the  -direction, we conclude that
-direction, we conclude that 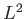 also
commutes with
also
commutes with 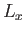 and
and 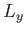 . It is clear from Equations (4.8)-(4.10) and
(4.19) that the best we
can do in quantum mechanics is to specify the
magnitude of an angular momentum vector
along with one of its components (by convention, the
. It is clear from Equations (4.8)-(4.10) and
(4.19) that the best we
can do in quantum mechanics is to specify the
magnitude of an angular momentum vector
along with one of its components (by convention, the  -component).
-component).
It is convenient to define the ladder operators, 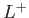 and
and 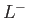 :
:
It can easily be shown that
and also that both ladder operators commute with 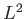 . (See Exercise 1.)
. (See Exercise 1.)



Next: Eigenvalues of Orbital Angular
Up: Orbital Angular Momentum
Previous: Orbital Angular Momentum
Richard Fitzpatrick
2016-01-22
![]() particles in the system, with
angular momentum vectors
particles in the system, with
angular momentum vectors ![]() (where
(where ![]() runs from 1 to
runs from 1 to ![]() ).
Each of these vectors satisfies Equation (4.11), so that
).
Each of these vectors satisfies Equation (4.11), so that
![]() -component,
-component, ![]() .
.
![]() . The commutator of
. The commutator of ![]() and
and ![]() is
written
is
written
![]() and
and ![]() :
: