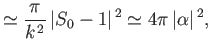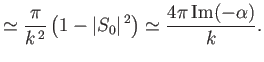Next: Scattering of Identical Particles
Up: Scattering Theory
Previous: Resonant Scattering
According to the analysis of Section 10.5, for the case of a spherically symmetric scattering potential,
the scattered wave is characterized by
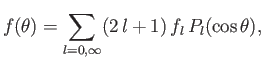 |
(10.138) |
where
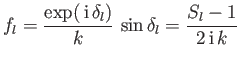 |
(10.139) |
is the amplitude of the  th partial wave, whereas
th partial wave, whereas 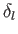 is the associated phase-shift. Here,
is the associated phase-shift. Here,
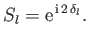 |
(10.140) |
Moreover, the fact that 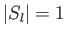 ensures that the scattering
is elastic (i.e., that the number of particles is conserved).
Finally, according to the analysis of Section 10.6, the net elastic scattering cross-section can be written
ensures that the scattering
is elastic (i.e., that the number of particles is conserved).
Finally, according to the analysis of Section 10.6, the net elastic scattering cross-section can be written
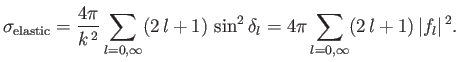 |
(10.141) |
It turns out that many scattering experiments are characterized by the absorption of some of the incident particles. Such
absorption may induce a change in the quantum state of the target, or, perhaps, the emergence of another
particle. Note that scattering that does not conserve particle number is
known as inelastic scattering. We can take inelastic scattering into account in our analysis by writing
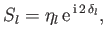 |
(10.142) |
where the real parameter 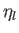 is such that
is such that
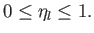 |
(10.143) |
It follows from Equation (10.140) that
![$\displaystyle f_l = \frac{\eta_l\,\sin(2\,\delta_l)}{2\,k} + {\rm i}\left[\frac{1-\eta_l\,\cos(2\,\delta_l)}{2\,k}\right].$](img3688.png) |
(10.144) |
Hence, according to Equation (10.142), the net elastic scattering cross-section becomes
The net inelastic scattering (i.e., absorption) cross-section follows from Equations (10.24) and
(10.88):
Thus, the total cross-section is
Note, from Equations (10.139), (10.140), and (10.143) that
![$\displaystyle {\rm Im}[f(0)] =\frac{1}{2\,k} \sum_{l=0,\infty} (2\,l+1)\left[1-\eta_l\,\cos (2\,\delta_l)\right].$](img3698.png) |
(10.148) |
In other words,
![$\displaystyle \sigma_{\rm total} = \frac{4\pi}{k}\,{\rm Im}[f(0)].$](img3699.png) |
(10.149) |
Hence, we deduce that the optical theorem, described in Section 10.6, still applies in the presence of
inelastic scattering.
If 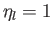 then there is no absorption, and the
then there is no absorption, and the  th partial wave is scattered in a completely elastic manner. On the
other hand, if
th partial wave is scattered in a completely elastic manner. On the
other hand, if 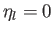 then there is total absorption of the
then there is total absorption of the  th partial wave. However,
such absorption is necessarily accompanied by some degree of elastic scattering. In order to
illustrate this important point, let us investigate the special case of scattering by a black sphere.
Such a sphere has a well-defined edge of radius
th partial wave. However,
such absorption is necessarily accompanied by some degree of elastic scattering. In order to
illustrate this important point, let us investigate the special case of scattering by a black sphere.
Such a sphere has a well-defined edge of radius 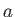 , and is completely absorbing. Consider short-wavelength
scattering characterized by
, and is completely absorbing. Consider short-wavelength
scattering characterized by 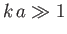 . In this case, we expect all partial waves with
. In this case, we expect all partial waves with
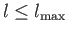 ,
where
,
where
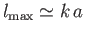 ,
to be completely absorbed (because, by analogy with classical physics, the impact parameters of the associated particles are
less than
,
to be completely absorbed (because, by analogy with classical physics, the impact parameters of the associated particles are
less than 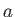 --see Section 10.8), and all other partial waves to suffer neither absorption nor scattering.
In other words,
--see Section 10.8), and all other partial waves to suffer neither absorption nor scattering.
In other words, 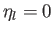 for
for
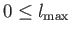 , and
, and 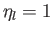 ,
,
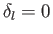 for
for
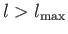 .
It follows from Equations (10.146) and (10.147) that
.
It follows from Equations (10.146) and (10.147) that
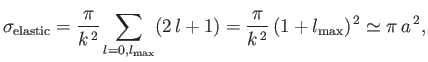 |
(10.150) |
and
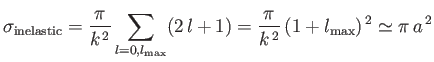 |
(10.151) |
[59].
Thus, the total scattering cross-section is
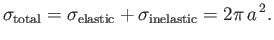 |
(10.152) |
This result seems a little strange, at first, because, by analogy with classical physics, we would not expect the
total cross-section to exceed the cross-section presented by the sphere. Nor would we expect a totally absorbing sphere to give rise to any elastic scattering. In fact,
this reasoning is incorrect. The absorbing sphere removes flux proportional to
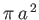 from the incident
wave, which leads to the formation of a shadow behind the sphere. However, a long way from the sphere,
the shadow gets filled in. In other words, the shadow is not visible infinitely far downstream of the sphere. The only way in which this can occur is via the diffraction of some of the
incident wave around the edges of the sphere. Actually, the amount of the incident wave that must
be diffracted is the same amount as was removed from the wave by absorption. Thus, the scattered flux is
also proportional to
from the incident
wave, which leads to the formation of a shadow behind the sphere. However, a long way from the sphere,
the shadow gets filled in. In other words, the shadow is not visible infinitely far downstream of the sphere. The only way in which this can occur is via the diffraction of some of the
incident wave around the edges of the sphere. Actually, the amount of the incident wave that must
be diffracted is the same amount as was removed from the wave by absorption. Thus, the scattered flux is
also proportional to
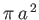 [53].
[53].
Consider low-energy scattering by a hard-sphere potential. As we saw in Section 10.8,
this process is dominated by S-wave (i.e., 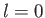 ) scattering. Moreover, the phase-shift of the S-wave takes the form
) scattering. Moreover, the phase-shift of the S-wave takes the form
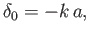 |
(10.153) |
where 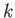 is the wavenumber of the incident particles, and
is the wavenumber of the incident particles, and 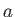 is the radius of the sphere. Note that the low-energy
limit corresponds to
is the radius of the sphere. Note that the low-energy
limit corresponds to 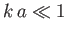 .
It follows that
.
It follows that
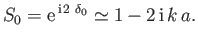 |
(10.154) |
We can generalize the previous analysis to take absorption into account by writing
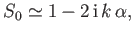 |
(10.155) |
where 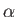 is complex,
is complex,
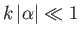 , and
, and
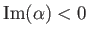 . According to Equations (10.146) and
(10.147),
. According to Equations (10.146) and
(10.147),
We conclude that the low-energy elastic scattering cross-section is again independent of the incident particle velocity (which
is proportional to 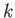 ), whereas the
inelastic cross-section is inversely proportional to the particle velocity. Consequently, as the incident
particle velocity decreases, inelastic scattering becomes more and more important in comparison with
elastic scattering [8].
), whereas the
inelastic cross-section is inversely proportional to the particle velocity. Consequently, as the incident
particle velocity decreases, inelastic scattering becomes more and more important in comparison with
elastic scattering [8].



Next: Scattering of Identical Particles
Up: Scattering Theory
Previous: Resonant Scattering
Richard Fitzpatrick
2016-01-22
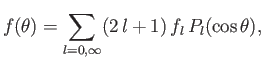
![$\displaystyle f_l = \frac{\eta_l\,\sin(2\,\delta_l)}{2\,k} + {\rm i}\left[\frac{1-\eta_l\,\cos(2\,\delta_l)}{2\,k}\right].$](img3688.png)
![$\displaystyle = \frac{2\pi}{k^{\,2}}\sum_{l=0,\infty} (2\,l+1)\left[1-\eta_l\,\cos (2\,\delta_l)\right].$](img3697.png)
![$\displaystyle {\rm Im}[f(0)] =\frac{1}{2\,k} \sum_{l=0,\infty} (2\,l+1)\left[1-\eta_l\,\cos (2\,\delta_l)\right].$](img3698.png)
![$\displaystyle \sigma_{\rm total} = \frac{4\pi}{k}\,{\rm Im}[f(0)].$](img3699.png)
![]() then there is no absorption, and the
then there is no absorption, and the ![]() th partial wave is scattered in a completely elastic manner. On the
other hand, if
th partial wave is scattered in a completely elastic manner. On the
other hand, if ![]() then there is total absorption of the
then there is total absorption of the ![]() th partial wave. However,
such absorption is necessarily accompanied by some degree of elastic scattering. In order to
illustrate this important point, let us investigate the special case of scattering by a black sphere.
Such a sphere has a well-defined edge of radius
th partial wave. However,
such absorption is necessarily accompanied by some degree of elastic scattering. In order to
illustrate this important point, let us investigate the special case of scattering by a black sphere.
Such a sphere has a well-defined edge of radius ![]() , and is completely absorbing. Consider short-wavelength
scattering characterized by
, and is completely absorbing. Consider short-wavelength
scattering characterized by ![]() . In this case, we expect all partial waves with
. In this case, we expect all partial waves with
![]() ,
where
,
where
![]() ,
to be completely absorbed (because, by analogy with classical physics, the impact parameters of the associated particles are
less than
,
to be completely absorbed (because, by analogy with classical physics, the impact parameters of the associated particles are
less than ![]() --see Section 10.8), and all other partial waves to suffer neither absorption nor scattering.
In other words,
--see Section 10.8), and all other partial waves to suffer neither absorption nor scattering.
In other words, ![]() for
for
![]() , and
, and ![]() ,
,
![]() for
for
![]() .
It follows from Equations (10.146) and (10.147) that
.
It follows from Equations (10.146) and (10.147) that
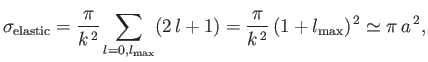
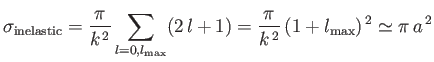
![]() ) scattering. Moreover, the phase-shift of the S-wave takes the form
) scattering. Moreover, the phase-shift of the S-wave takes the form