Next: Collisionality Parameters Up: Neoclassical Theory Previous: Introduction Contents
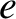 ), majority ions (
), majority ions (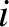 ), and impurity ions
(
), and impurity ions
(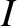 ). The charges of the three species are
). The charges of the three species are 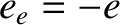 ,
,  , and
, and
 , respectively, where
, respectively, where
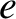 is the magnitude of the electron charge.
Quasi-neutrality [1] demands that
where
is the magnitude of the electron charge.
Quasi-neutrality [1] demands that
where 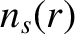 is the species-
is the species- number density. Here,
number density. Here,  is the flux-surface label introduced in Section 14.2.
Let
is the flux-surface label introduced in Section 14.2.
Let
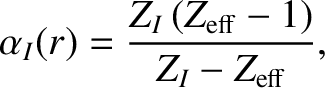 |
(A.2) |
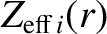 |
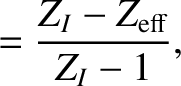 |
(A.6) |
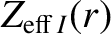 |
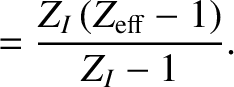 |
(A.7) |
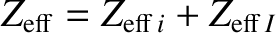 .
.