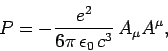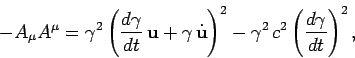Next: Radiation losses
Up: Relativity and electromagnetism
Previous: Accelerated charges
Let us transform to the inertial frame in which the charge is instantaneously
at rest at the origin at time
 . In this frame,
. In this frame,  ,
so that
,
so that
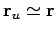 and
and 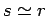 for
events which are sufficiently close to the origin at
for
events which are sufficiently close to the origin at 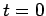 that the retarded
charge still appears to travel with a velocity which is small
compared to that of light. It follows from the previous section that
that the retarded
charge still appears to travel with a velocity which is small
compared to that of light. It follows from the previous section that
Let us define spherical polar coordinates whose axis points along the
direction of instantaneous
acceleration of the charge.
It is easily demonstrated that
These fields are identical to those of a radiating dipole whose axis is
aligned along the direction of instantaneous acceleration (see Sect. 9.2). The Poynting flux
is given by
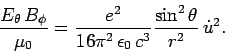 |
(1630) |
We can integrate this expression to obtain the instantaneous
power radiated by the charge
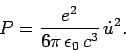 |
(1631) |
This is known as Larmor's formula. Note that zero net momentum
is carried off by the fields (1628) and (1629).
In order to proceed further, it is necessary to prove two useful theorems.
The first theorem states that if a 4-vector field 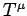 satisfies
satisfies
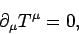 |
(1632) |
and if the components of 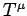 are non-zero only in a finite
spatial region, then the integral over 3-space,
are non-zero only in a finite
spatial region, then the integral over 3-space,
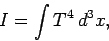 |
(1633) |
is an invariant. In order to prove this theorem, we need to
use the 4-dimensional analog of Gauss's theorem, which states that
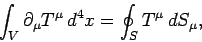 |
(1634) |
where 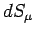 is an element of the 3-dimensional surface
is an element of the 3-dimensional surface 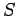 bounding the 4-dimensional volume
bounding the 4-dimensional volume 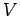 . The
particular volume over which the
integration is performed is indicated in Fig. 58. The surfaces
. The
particular volume over which the
integration is performed is indicated in Fig. 58. The surfaces
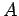 and
and 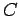 are chosen so that the spatial components of
are chosen so that the spatial components of 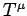 vanish on
vanish on 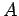 and
and 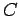 . This is always possible because it is assumed that
the region over which the components of
. This is always possible because it is assumed that
the region over which the components of 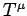 are non-zero
is of finite extent. The surface
are non-zero
is of finite extent. The surface 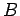 is chosen normal to the
is chosen normal to the 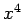 -axis,
whereas the surface
-axis,
whereas the surface 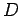 is chosen normal to the
is chosen normal to the 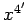 -axis. Here,
the
-axis. Here,
the 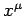 and the
and the 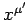 are coordinates in two arbitrarily
chosen inertial frames. It follows from Eq. (1634) that
are coordinates in two arbitrarily
chosen inertial frames. It follows from Eq. (1634) that
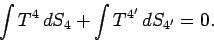 |
(1635) |
Here, we have made use of the fact that 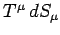 is a scalar
and, therefore, has the same value in all inertial frames. Since
is a scalar
and, therefore, has the same value in all inertial frames. Since
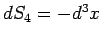 and
and
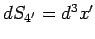 it follows that
it follows that
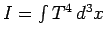 is an invariant under a Lorentz transformation.
Incidentally, the above argument also demonstrates that
is an invariant under a Lorentz transformation.
Incidentally, the above argument also demonstrates that 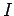 is constant
in time (just take the limit in which the two inertial frames
are identical).
is constant
in time (just take the limit in which the two inertial frames
are identical).
Figure 58:
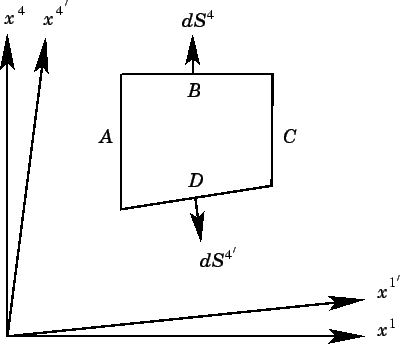 |
The second theorem is an extension of the first. Suppose that a 4-tensor
field 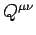 satisfies
satisfies
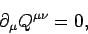 |
(1636) |
and has components which are only non-zero in a finite spatial
region. Let 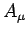 be a 4-vector whose coefficients do not vary with
position in space-time.
It follows that
be a 4-vector whose coefficients do not vary with
position in space-time.
It follows that
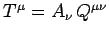 satisfies Eq. (1632). Therefore,
satisfies Eq. (1632). Therefore,
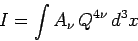 |
(1637) |
is an invariant. However, we can write
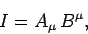 |
(1638) |
where
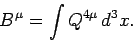 |
(1639) |
It follows from the quotient rule that if 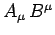 is an invariant
for arbitrary
is an invariant
for arbitrary 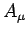 then
then 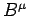 must transform as a
constant (in time) 4-vector.
must transform as a
constant (in time) 4-vector.
These two theorems enable us to convert differential conservation laws
into integral conservation laws. For instance, in differential form,
the conservation of electrical charge is written
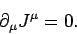 |
(1640) |
However, from Eq. (1635) this immediately implies that
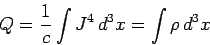 |
(1641) |
is an invariant. In other words, the total electrical charge contained in
space is both constant in time, and the same in all inertial frames.
Suppose that 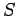 is the instantaneous rest frame of the charge. Let us
consider the electromagnetic energy tensor
is the instantaneous rest frame of the charge. Let us
consider the electromagnetic energy tensor 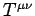 associated with
all of the radiation emitted by the charge between times
associated with
all of the radiation emitted by the charge between times 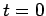 and
and 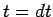 .
According to Eq. (1583), this tensor field satisfies
.
According to Eq. (1583), this tensor field satisfies
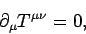 |
(1642) |
apart from a region of space of measure zero in the vicinity of the charge.
Furthermore, the region of space over which 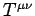 is non-zero is
clearly finite, since we are only considering the fields emitted by the
charge in a small time interval, and these fields propagate at a
finite velocity. Thus, according to the second theorem
is non-zero is
clearly finite, since we are only considering the fields emitted by the
charge in a small time interval, and these fields propagate at a
finite velocity. Thus, according to the second theorem
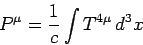 |
(1643) |
is a 4-vector. It follows from Sect. 10.22 that we can write
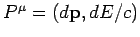 , where
, where 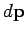 and
and 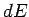 are the total
momentum and energy carried off by the radiation emitted between times
are the total
momentum and energy carried off by the radiation emitted between times
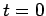 and
and 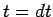 , respectively. As we have already mentioned,
, respectively. As we have already mentioned,
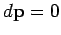 in the instantaneous rest frame
in the instantaneous rest frame 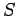 . Transforming to an
arbitrary inertial frame
. Transforming to an
arbitrary inertial frame
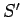 , in which the instantaneous velocity of the charge is
, in which the instantaneous velocity of the charge is 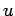 ,
we obtain
,
we obtain
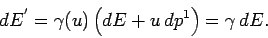 |
(1644) |
However, the time interval over which the radiation is emitted in 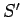 is
is
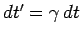 . Thus, the instantaneous power radiated by the charge,
. Thus, the instantaneous power radiated by the charge,
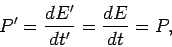 |
(1645) |
is the same in all inertial frames.
We can make use of the fact that the power radiated by an accelerating charge
is Lorentz invariant to find a relativistic generalization of the
Larmor formula, (1631), which is valid in all inertial frames. We expect the
power emitted by the charge to depend only on its 4-velocity and
4-acceleration.
It follows that the Larmor formula can be written in Lorentz invariant form as
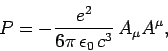 |
(1646) |
since the 4-acceleration takes the form
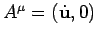 in the instantaneous rest frame. In a general inertial
frame,
in the instantaneous rest frame. In a general inertial
frame,
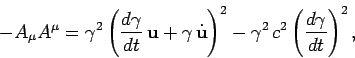 |
(1647) |
where use has been made of Eq. (1429).
Furthermore, it is easily demonstrated that
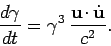 |
(1648) |
It follows, after a little algebra, that the relativistic generalization
of Larmor's formula takes the form
![\begin{displaymath}
P= \frac{e^2}{6\pi \epsilon_0 c^3} \gamma^6 \left[ \dot{\bf u}^2 -
\frac{({\bf u}\times\dot{\bf u})^2}{c^2}\right].
\end{displaymath}](img3320.png) |
(1649) |



Next: Radiation losses
Up: Relativity and electromagnetism
Previous: Accelerated charges
Richard Fitzpatrick
2006-02-02
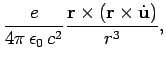
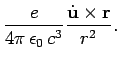
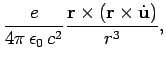
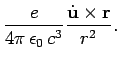
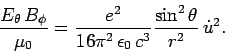
![]() satisfies
satisfies
![]() satisfies
satisfies
![]() is the instantaneous rest frame of the charge. Let us
consider the electromagnetic energy tensor
is the instantaneous rest frame of the charge. Let us
consider the electromagnetic energy tensor ![]() associated with
all of the radiation emitted by the charge between times
associated with
all of the radiation emitted by the charge between times ![]() and
and ![]() .
According to Eq. (1583), this tensor field satisfies
.
According to Eq. (1583), this tensor field satisfies