Next, let us consider terms in the solution of the lunar equations of motions that depend on the lunar eccentricity,  , but are independent of the
lunar inclination,
, but are independent of the
lunar inclination, 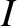 .
.
According to Equations (11.133) and (11.145),
It follows from Equations (11.192), (11.193), (11.199), (11.217), and (11.218) that
According to Equations (11.134) and (11.146),
It follows from Equations (11.192), (11.193), (11.200), (11.217), and (11.218) that
According to Equations (11.128) and (11.140),
It follows from Equation (11.217) and (11.218), as well as the previous expressions for
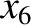 and
and 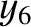 , that
where
Thus, Equations (11.190) and (11.191) reduce to
, that
where
Thus, Equations (11.190) and (11.191) reduce to
![\begin{displaymath}\left(
\begin{array}{ll}
A_{xx}, & A_{xy}\\ [0.5ex]
A_{yx},&A...
...ht)
=
\left(
\begin{array}{c}
0\\ [0.5ex]
0
\end{array}\right),\end{displaymath}](img3550.png) |
(11.277) |
where
The non-trivial solution of the homogenous matrix equation (11.277) is obtained by setting the determinant of the matrix to zero (Riley 1974d).
In other words,
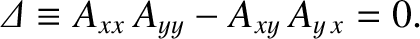 |
(11.282) |
It follows from Equations (11.194), (11.273)–(11.276), and (11.278)–(11.281) that
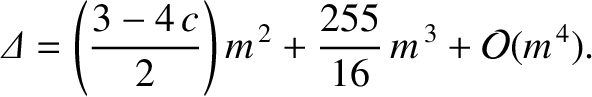 |
(11.283) |
Thus, setting
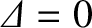 , we get
, we get
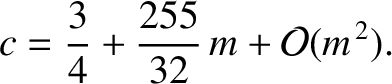 |
(11.284) |
Moreover, Equations (11.278)–(11.281) reduce to
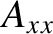 |
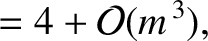 |
(11.285) |
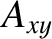 |
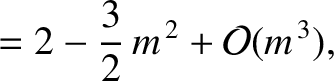 |
(11.286) |
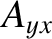 |
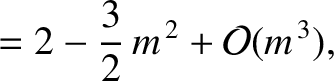 |
(11.287) |
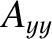 |
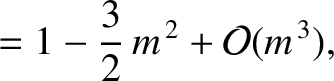 |
(11.288) |
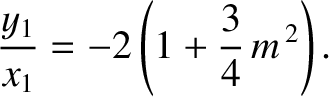 |
(11.289) |
The arbitrary parameter 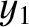 is chosen such that the parameter
is chosen such that the parameter 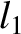 , appearing in Equation (11.123), is the same as in the
undisturbed motion. Thus, making use of Equations (11.172),
Hence, Equations (11.263), (11.264), (11.267), and (11.268) reduce to
, appearing in Equation (11.123), is the same as in the
undisturbed motion. Thus, making use of Equations (11.172),
Hence, Equations (11.263), (11.264), (11.267), and (11.268) reduce to
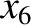 |
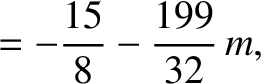 |
(11.292) |
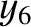 |
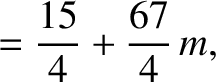 |
(11.293) |
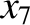 |
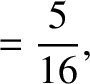 |
(11.294) |
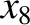 |
 |
(11.295) |
According to Equation (11.126),
 |
(11.296) |
where use has been made of the previous expressions for 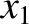 and
and 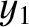 . Equation (11.189) yields
. Equation (11.189) yields
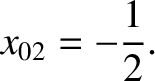 |
(11.297) |
According to Equations (11.129) and (11.141),
where use has been made of the previous expressions for 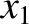 and
and 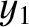 .
Hence, Equations (11.192), (11.193), and (11.195) yield
.
Hence, Equations (11.192), (11.193), and (11.195) yield
It follows from Equations (11.122)–(11.124), (11.158), (11.160) (11.161), (11.165), (11.166), (11.172), (11.173),
(11.177), and (11.178), as well as the previous expressions for 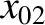 ,
, 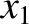 ,
, 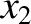 ,
, 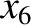 ,
, 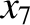 ,
, 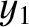 ,
, 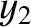 ,
, 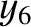 , and
, and 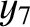 , that the net perturbation of the lunar orbit
due to terms in the solution of the lunar equations of motion that depend on
, that the net perturbation of the lunar orbit
due to terms in the solution of the lunar equations of motion that depend on  , but are independent of
, but are independent of 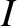 , is
, is
The previous expressions are accurate to
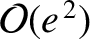 and
and
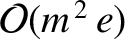 .
.
The first two terms on the
right-hand side of expression (11.303) are Keplerian in origin (i.e., they are independent of the perturbing
action of the Sun). In fact, the first is due to the eccentricity of the lunar orbit (i.e., the fact that the
geometric center of the orbit is slightly shifted from the center of the Earth), and the second is due to
the ellipticity of the orbit (i.e., the fact that the orbit is slightly noncircular). These terms are usually called the major
inequality
The third term on the right-hand side of expression (11.303) corresponds to
evection, and is due to the combined action of the Sun and the eccentricity of the lunar orbit.
In fact, evection can be thought of as causing a slight reduction in the eccentricity of the lunar
orbit around the times of the new moon and the full moon (i.e., 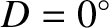 and
and
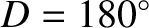 ), and
a corresponding slight increase in the eccentricity around the times of the first and last quarter moons
(i.e.,
), and
a corresponding slight increase in the eccentricity around the times of the first and last quarter moons
(i.e.,
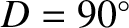 and
and
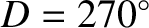 ). (See Section 11.18, Exercise 4.) This follows because the evection term in Equation (11.303) augments the eccentricity
term,
). (See Section 11.18, Exercise 4.) This follows because the evection term in Equation (11.303) augments the eccentricity
term,
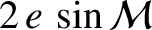 , when
, when
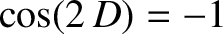 , and reduces the term when
, and reduces the term when
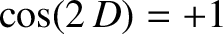 .
Evection generates a perturbation in the lunar ecliptic longitude that oscillates sinusoidally with a period of
.
Evection generates a perturbation in the lunar ecliptic longitude that oscillates sinusoidally with a period of 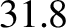 days. This
oscillation period is in good agreement with observations.
However, the amplitude of the oscillation (calculated using
days. This
oscillation period is in good agreement with observations.
However, the amplitude of the oscillation (calculated using 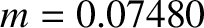 and
and  )
is
)
is 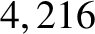 arc seconds, which is somewhat less than the observed amplitude of
arc seconds, which is somewhat less than the observed amplitude of 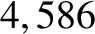 arc seconds (Chapront-Touzé and Chapront 1988). Again, this discrepancy between
theory and observation is due to the fact that we have only calculated the lowest-order (in
arc seconds (Chapront-Touzé and Chapront 1988). Again, this discrepancy between
theory and observation is due to the fact that we have only calculated the lowest-order (in 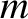 and
and  ) contributions to evection.
) contributions to evection.
 , but are independent of the
lunar inclination,
, but are independent of the
lunar inclination, 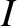 .
.
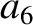
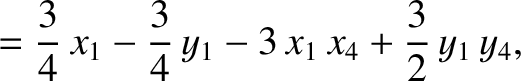
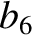
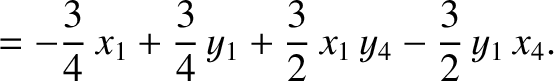
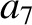
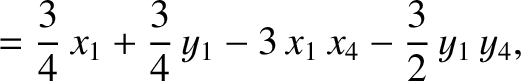
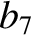
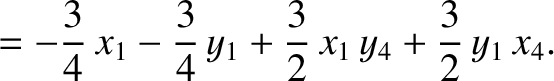
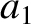
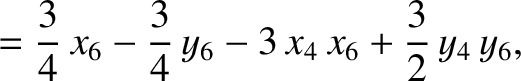
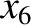 and
and 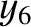 , that
, that
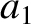
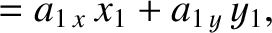
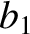
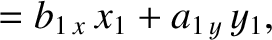
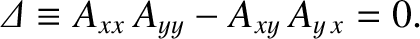
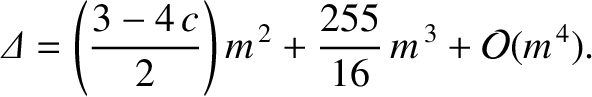
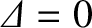 , we get
Moreover, Equations (11.278)–(11.281) reduce to
, we get
Moreover, Equations (11.278)–(11.281) reduce to
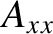
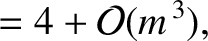
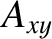
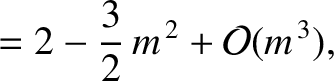
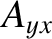
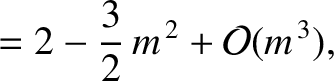
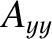
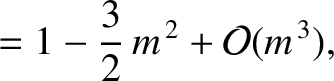
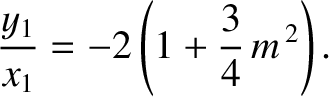
 is chosen such that the parameter
is chosen such that the parameter  , appearing in Equation (11.123), is the same as in the
undisturbed motion. Thus, making use of Equations (11.172),
, appearing in Equation (11.123), is the same as in the
undisturbed motion. Thus, making use of Equations (11.172),
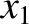
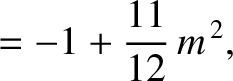
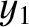
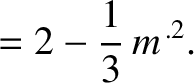
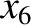
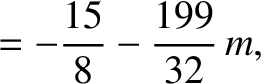
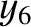
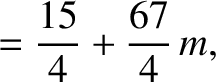
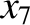
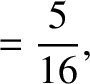
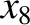
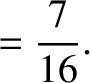

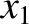 and
and 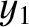 . Equation (11.189) yields
. Equation (11.189) yields
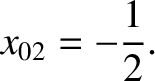
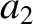
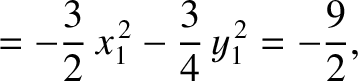
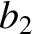
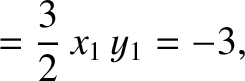
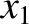 and
and 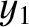 .
Hence, Equations (11.192), (11.193), and (11.195) yield
.
Hence, Equations (11.192), (11.193), and (11.195) yield
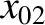 ,
, 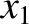 ,
, 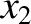 ,
, 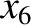 ,
, 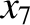 ,
, 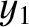 ,
, 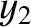 ,
, 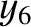 , and
, and 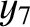 , that the net perturbation of the lunar orbit
due to terms in the solution of the lunar equations of motion that depend on
, that the net perturbation of the lunar orbit
due to terms in the solution of the lunar equations of motion that depend on  , but are independent of
, but are independent of 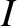 , is
, is
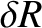
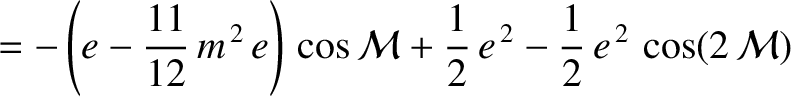
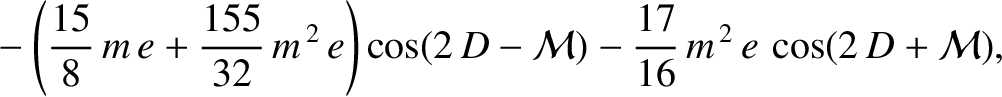
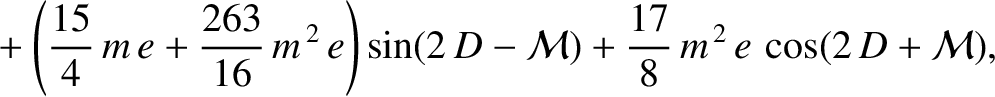
 and
and
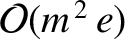 .
.
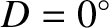 and
and
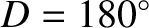 ), and
a corresponding slight increase in the eccentricity around the times of the first and last quarter moons
(i.e.,
), and
a corresponding slight increase in the eccentricity around the times of the first and last quarter moons
(i.e.,
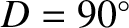 and
and
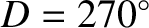 ). (See Section 11.18, Exercise 4.) This follows because the evection term in Equation (11.303) augments the eccentricity
term,
). (See Section 11.18, Exercise 4.) This follows because the evection term in Equation (11.303) augments the eccentricity
term,
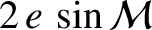 , when
, when
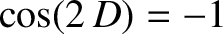 , and reduces the term when
, and reduces the term when
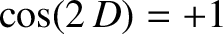 .
Evection generates a perturbation in the lunar ecliptic longitude that oscillates sinusoidally with a period of
.
Evection generates a perturbation in the lunar ecliptic longitude that oscillates sinusoidally with a period of 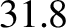 days. This
oscillation period is in good agreement with observations.
However, the amplitude of the oscillation (calculated using
days. This
oscillation period is in good agreement with observations.
However, the amplitude of the oscillation (calculated using 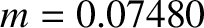 and
and  )
is
)
is 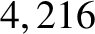 arc seconds, which is somewhat less than the observed amplitude of
arc seconds, which is somewhat less than the observed amplitude of 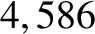 arc seconds (Chapront-Touzé and Chapront 1988). Again, this discrepancy between
theory and observation is due to the fact that we have only calculated the lowest-order (in
arc seconds (Chapront-Touzé and Chapront 1988). Again, this discrepancy between
theory and observation is due to the fact that we have only calculated the lowest-order (in 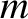 and
and  ) contributions to evection.
) contributions to evection.