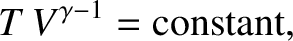Suppose that the temperature of an ideal
gas is held constant by keeping the gas in thermal
contact with a heat reservoir. If the gas is allowed to expand quasi-statically
under these so-called isothermal conditions then the ideal gas law, (5.97),
tells us that
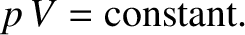 |
(5.114) |
This result is known as the isothermal gas law.
Suppose, now, that the gas is thermally isolated from its surroundings. If
the gas is allowed to expand quasi-statically under these so-called
adiabatic
conditions then
it does work on its environment, and, hence, its internal energy is reduced,
and its temperature decreases. Let us calculate the relationship between the
pressure and volume of the gas during adiabatic expansion.
According to Equation (5.109),
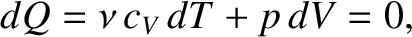 |
(5.115) |
in an adiabatic process (in which no heat is absorbed). The ideal gas
law, (5.97), can be differentiated, yielding
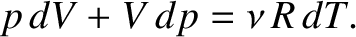 |
(5.116) |
The temperature increment, 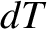 , can be eliminated between the previous two expressions
to give
, can be eliminated between the previous two expressions
to give
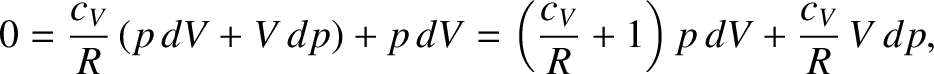 |
(5.117) |
which reduces to
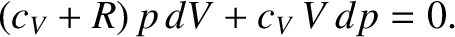 |
(5.118) |
Dividing through by
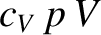 yields
yields
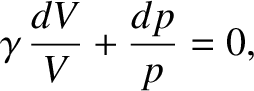 |
(5.119) |
where
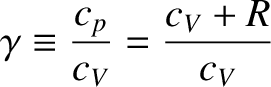 |
(5.120) |
is termed the ratio of specific heats.
[See Equation (5.113).]
Given that 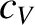 is a constant in an ideal gas, the ratio
of specific heats,
is a constant in an ideal gas, the ratio
of specific heats,  , is also a constant. In fact, Equations (5.106), (5.107), and the previous
equation,
imply that
, is also a constant. In fact, Equations (5.106), (5.107), and the previous
equation,
imply that
 |
(5.121) |
for a monatomic gas, and
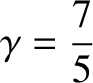 |
(5.122) |
for a diatomic gas.
Because  is a constant for an ideal gas, we can integrate Equation (5.119) to give
is a constant for an ideal gas, we can integrate Equation (5.119) to give
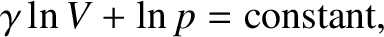 |
(5.123) |
or
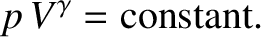 |
(5.124) |
This result is known as the adiabatic gas law.
It is straightforward to obtain analogous relationships between  and
and  , and between
, and between 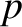 and
and  ,
during adiabatic expansion or contraction. In fact, because
,
during adiabatic expansion or contraction. In fact, because
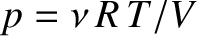 , the previous formula
also implies that
, the previous formula
also implies that
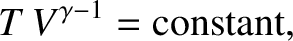 |
(5.125) |
and
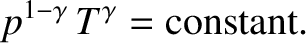 |
(5.126) |
Equations (5.124)–(5.126) are all completely equivalent.
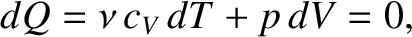
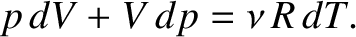
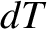 , can be eliminated between the previous two expressions
to give
, can be eliminated between the previous two expressions
to give
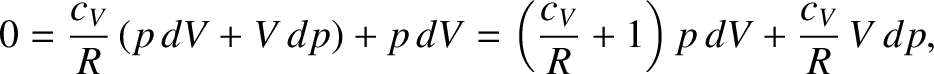
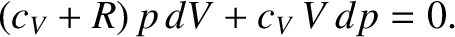
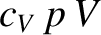 yields
where
yields
where
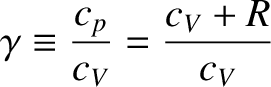
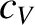 is a constant in an ideal gas, the ratio
of specific heats,
is a constant in an ideal gas, the ratio
of specific heats,  , is also a constant. In fact, Equations (5.106), (5.107), and the previous
equation,
imply that
, is also a constant. In fact, Equations (5.106), (5.107), and the previous
equation,
imply that

 is a constant for an ideal gas, we can integrate Equation (5.119) to give
is a constant for an ideal gas, we can integrate Equation (5.119) to give
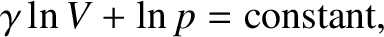
 and
and  , and between
, and between 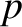 and
and  ,
during adiabatic expansion or contraction. In fact, because
,
during adiabatic expansion or contraction. In fact, because
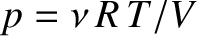 , the previous formula
also implies that
, the previous formula
also implies that