Next: Compton Scattering Up: Experimental Basis of Quantum Previous: Wave-Particle Duality
In 1905, Albert Einstein proposed a radical new theory of light in order to
account for the photoelectric effect. According to this theory, light
of fixed angular frequency  consists of a collection of indivisible discrete packages, called
quanta,4.1 whose energy is
consists of a collection of indivisible discrete packages, called
quanta,4.1 whose energy is
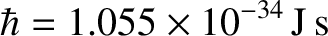 |
(4.2) |
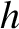 , divided by
, divided by 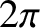 .) Incidentally,
.) Incidentally, 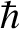 is called Planck's constant, rather than Einstein's constant, because Max Planck first introduced the concept of the quantization of light, in 1900, while trying
to account for the electromagnetic spectrum of a black body (i.e.,
a perfect emitter and absorber of electromagnetic radiation). (See Section 5.6.2.)
is called Planck's constant, rather than Einstein's constant, because Max Planck first introduced the concept of the quantization of light, in 1900, while trying
to account for the electromagnetic spectrum of a black body (i.e.,
a perfect emitter and absorber of electromagnetic radiation). (See Section 5.6.2.)
Suppose that the electrons at the surface of a piece of metal lie in a potential well
of depth 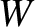 . In other words, the electrons have to acquire an energy
. In other words, the electrons have to acquire an energy 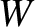 in order to be emitted from the surface. Here,
in order to be emitted from the surface. Here, 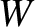 is generally called
the workfunction of the surface, and is a property of the
metal. Suppose that an electron absorbs a single quantum of light, otherwise known as a photon. Its energy
therefore increases by
is generally called
the workfunction of the surface, and is a property of the
metal. Suppose that an electron absorbs a single quantum of light, otherwise known as a photon. Its energy
therefore increases by
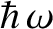 . If
. If
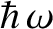 is greater than
is greater than 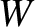 then the
electron is emitted from the surface with the residual kinetic energy
then the
electron is emitted from the surface with the residual kinetic energy
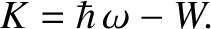 |
(4.3) |
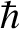 ,
and whose intercept with the
,
and whose intercept with the  axis is
axis is 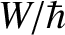 . Finally, the number
of emitted electrons increases with the intensity of the light because, the
more intense the light, the larger the flux of photons onto the surface.
Thus, Einstein's quantum theory of light is capable of accounting for all
three of the previously mentioned observational facts regarding the photoelectric
effect.
. Finally, the number
of emitted electrons increases with the intensity of the light because, the
more intense the light, the larger the flux of photons onto the surface.
Thus, Einstein's quantum theory of light is capable of accounting for all
three of the previously mentioned observational facts regarding the photoelectric
effect.
Of course, an electromagnetic wave of angular frequency  propagates though a vacuum at the speed of light in vacuum,
propagates though a vacuum at the speed of light in vacuum,  . However,
if such a wave actually consists of a swarm of photons then it seems reasonable to
suppose that these photons also move through a vacuum at the speed
. However,
if such a wave actually consists of a swarm of photons then it seems reasonable to
suppose that these photons also move through a vacuum at the speed  . As discussed in Section 3.3.8, if photons move at the speed
. As discussed in Section 3.3.8, if photons move at the speed  then Einstein's special theory of relativity demands that they be massless particles with momenta
then Einstein's special theory of relativity demands that they be massless particles with momenta
 is the wavenumber of the associated electromagnetic wave.
is the wavenumber of the associated electromagnetic wave.