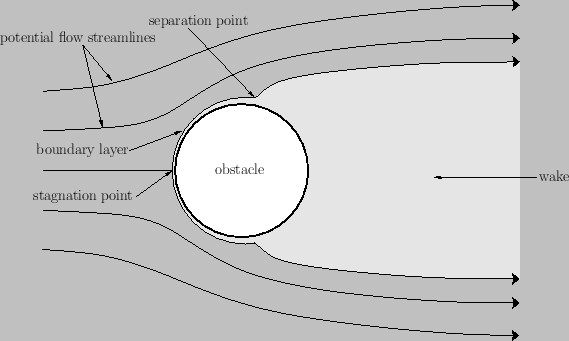
 |
Outside the boundary layer, and the wake, the flow pattern is irrotational and essentially inviscid. So, from Section 5.8, the tangential flow speed just outside the boundary layer (neglecting any circulation of the external flow around the cylinder) is
| (8.118) |
Boundary layer separation is an important physical phenomenon because it gives rise to a greatly
enhanced drag force acting on a non-streamlined obstacle placed in a high Reynolds number flow. This is the case because the pressure in the comparatively wide
wake that forms behind a non-streamlined obstacle, as a consequence of separation, is relatively low. To be more exact, in the
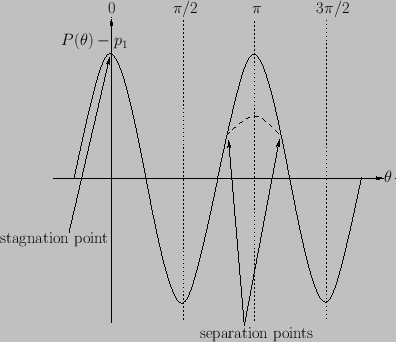
case of a cylindrical obstacle, Equation (8.119) specifies the expected pressure variation over the obstacle's surface in the
absence of separation. It can be seen that the variation on the front side of the obstacle mirrors
that on the back side: that is,
![]() . (See Figure 8.12.) In other words, the resultant pressure force
on the front side of the obstacle is equal and opposite to that on the back side, so that the pressure distribution
gives rise to zero net drag acting on the obstacle. Figure 8.12 illustrates how the pressure distribution is modified
as a consequence of boundary layer separation. In this case, the pressure between the separation points is significantly less than that on the
front side of the obstacle. Consequently, the resultant pressure force on the front side is
greater in magnitude than the oppositely directed force on the back side, giving rise to a significant drag
acting on the obstacle. Let
. (See Figure 8.12.) In other words, the resultant pressure force
on the front side of the obstacle is equal and opposite to that on the back side, so that the pressure distribution
gives rise to zero net drag acting on the obstacle. Figure 8.12 illustrates how the pressure distribution is modified
as a consequence of boundary layer separation. In this case, the pressure between the separation points is significantly less than that on the
front side of the obstacle. Consequently, the resultant pressure force on the front side is
greater in magnitude than the oppositely directed force on the back side, giving rise to a significant drag
acting on the obstacle. Let ![]() be the drag force per unit width (parallel to the axis
of the cylinder) exerted on the obstacle. It is convenient to parameterize this force in terms of a
dimensionless drag coefficient,
be the drag force per unit width (parallel to the axis
of the cylinder) exerted on the obstacle. It is convenient to parameterize this force in terms of a
dimensionless drag coefficient,
 |
Boundary layer separation is associated with strong adverse pressure gradients, or, equivalently, strong flow deceleration, on the
back side of an obstacle placed in a high Reynolds number flow. Such gradients can be significantly reduced by streamlining the obstacle: that is,
by closely aligning its back surface with the unperturbed streamlines of the external flow. Indeed, boundary
layer separation can be delayed, or even completely prevented, on the surface of a sufficiently streamlined
obstacle, thereby significantly decreasing, or even eliminating, the associated form drag (essentially, by reducing the cross-sectional area of the wake). However, even in the limit that the form drag
is reduced to a negligible level, there is still a residual drag acting on the obstacle due to boundary layer viscosity.
This type of drag is called friction drag. As is clear from a comparison of Equations (8.79) and
(8.120), the drag coefficient associated with friction drag is
![]() , where
, where ![]() is the
Reynolds number of the flow. Friction drag thus tends
to zero as the Reynolds number tends to infinity.
is the
Reynolds number of the flow. Friction drag thus tends
to zero as the Reynolds number tends to infinity.
The phenomenon of boundary layer separation allows us to resolve d'Alembert's paradox. Recall, from
Section 5.8, that an idealized fluid that is modeled as inviscid and irrotational is incapable of exerting a drag force on a stationary
obstacle, despite the fact that very high Reynolds number, ostensibly irrotational, fluids are observed to exert significant drag forces
on stationary obstacles. The resolution of the paradox lies in the realization that, in such fluids, viscosity can only be neglected (and the
flow is consequently only irrotational)
in the absence of boundary layer separation. In this case, the region of the fluid in which viscosity
plays a significant role is localized to a thin boundary layer on the surface of the obstacle, and the resultant
friction drag scales as
![]() , and, therefore, disappears in the inviscid limit (essentially,
because the boundary layer shrinks to zero thickness in this limit). On the other hand,
if the boundary layer separates then viscosity is important both in a thin boundary layer on the front
of the obstacle, and in a wide, low-pressure, vortex-filled, wake that forms behind the obstacle. Moreover, the
wake does not disappear in the inviscid limit.
The presence of significant fluid vorticity within the wake invalidates irrotational fluid dynamics.
Consequently, the pressure on the back side of the obstacle is significantly smaller than that
predicted by irrotational fluid dynamics. Hence, the resultant pressure force
on the front side is larger than that on the back side, and a significant drag is exerted on the obstacle.
The drag coefficient associated with this type of drag is generally of order unity, and does not tend to zero
as the Reynolds number tends to infinity.
, and, therefore, disappears in the inviscid limit (essentially,
because the boundary layer shrinks to zero thickness in this limit). On the other hand,
if the boundary layer separates then viscosity is important both in a thin boundary layer on the front
of the obstacle, and in a wide, low-pressure, vortex-filled, wake that forms behind the obstacle. Moreover, the
wake does not disappear in the inviscid limit.
The presence of significant fluid vorticity within the wake invalidates irrotational fluid dynamics.
Consequently, the pressure on the back side of the obstacle is significantly smaller than that
predicted by irrotational fluid dynamics. Hence, the resultant pressure force
on the front side is larger than that on the back side, and a significant drag is exerted on the obstacle.
The drag coefficient associated with this type of drag is generally of order unity, and does not tend to zero
as the Reynolds number tends to infinity.