Next: Cylindrical Waves Up: Multi-Dimensional Waves Previous: Plane Waves Contents
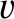 is the characteristic wave speed of the medium through which the wave propagates. (See Section 6.3.)
Likewise, the three-dimensional plane wave solution, (7.5), satisfies the three-dimensional
wave equation (see Exercise 1),
is the characteristic wave speed of the medium through which the wave propagates. (See Section 6.3.)
Likewise, the three-dimensional plane wave solution, (7.5), satisfies the three-dimensional
wave equation (see Exercise 1),