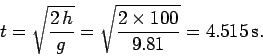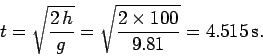Next: Worked example 3.3: Cannon
Up: Motion in 3 dimensions
Previous: Worked example 3.1: Broken
Question: Legend has it that Gallileo tested out his newly developed
theory of projectile motion by throwing weights from the top of the
Leaning Tower of
Pisa. (No wonder he eventually got into trouble with the authorities!)
Suppose that, one day, Gallileo simultaneously threw two equal weights off the tower from
a height of 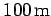 above the ground. Suppose, further, that he
dropped the first weight straight down,
whereas he threw the second weight horizontally with a velocity of
above the ground. Suppose, further, that he
dropped the first weight straight down,
whereas he threw the second weight horizontally with a velocity of 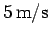 .
Which weight struck the ground first? How long, after it was thrown, did it take
to do this? Finally, what horizontal distance was traveled by the
second weight before it hit the ground? Neglect the effect of air
resistance.
.
Which weight struck the ground first? How long, after it was thrown, did it take
to do this? Finally, what horizontal distance was traveled by the
second weight before it hit the ground? Neglect the effect of air
resistance.
Answer: Since both weights start off traveling with the same initial velocities in the
vertical direction (i.e., zero), and both accelerate vertically downwards
at the same rate, it follows that both weights strike the ground simultaneously.
The time of flight of each weight is simply the time taken to fall
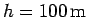 , starting from rest, under the influence of gravity. From
Eq. (27), this time is given by
, starting from rest, under the influence of gravity. From
Eq. (27), this time is given by
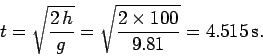
The horizontal distance  traveled by the second weight is simply the
distance traveled by a body moving at a constant velocity
traveled by the second weight is simply the
distance traveled by a body moving at a constant velocity
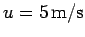 (recall that gravitational acceleration does not affect horizontal motion)
during the time taken by the weight to drop 100m. Thus,
(recall that gravitational acceleration does not affect horizontal motion)
during the time taken by the weight to drop 100m. Thus,



Next: Worked example 3.3: Cannon
Up: Motion in 3 dimensions
Previous: Worked example 3.1: Broken
Richard Fitzpatrick
2006-02-02