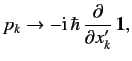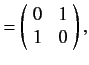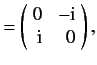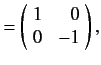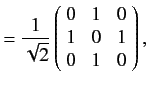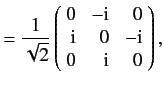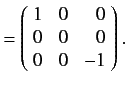Next: Exercises
Up: Spin Angular Momentum
Previous: Pauli Two-Component Formalism
In the absence of spin, the Hamiltonian can be written as some function
of the position and momentum operators. Using the Schrödinger representation,
in which
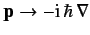 , the energy eigenvalue
problem,
, the energy eigenvalue
problem,
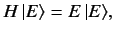 |
(520) |
can be transformed into a partial differential equation for the wavefunction
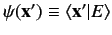 . This function specifies the
probability density for observing the particle at a given position,
. This function specifies the
probability density for observing the particle at a given position, 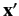 .
In general, we find
.
In general, we find
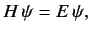 |
(521) |
where 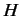 is now a partial differential operator.
The boundary conditions (for a bound state) are obtained
from the normalization constraint
is now a partial differential operator.
The boundary conditions (for a bound state) are obtained
from the normalization constraint
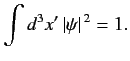 |
(522) |
This is all very familiar. However, we now know how to generalize this scheme
to deal with a spin one-half particle. Instead of representing the
state of the particle by a single wavefunction, we use two wavefunctions.
The first,
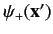 , specifies the probability density of
observing the particle at position
, specifies the probability density of
observing the particle at position  with spin angular momentum
with spin angular momentum 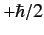 in the
in the 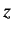 -direction. The second,
-direction. The second,
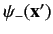 , specifies the
probability density of
observing the particle at position
, specifies the
probability density of
observing the particle at position 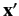 with spin angular momentum
with spin angular momentum 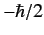 in the
in the 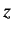 -direction. In the Pauli scheme, these wavefunctions
are combined into a spinor,
-direction. In the Pauli scheme, these wavefunctions
are combined into a spinor, 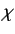 , which is simply the column vector of
, which is simply the column vector of 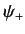 and
and 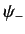 .
In general, the Hamiltonian is a function of the position, momentum, and spin
operators. Adopting the Schrödinger representation, and the Pauli scheme,
the energy eigenvalue problem reduces to
.
In general, the Hamiltonian is a function of the position, momentum, and spin
operators. Adopting the Schrödinger representation, and the Pauli scheme,
the energy eigenvalue problem reduces to
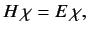 |
(523) |
where 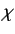 is a spinor (i.e., a
is a spinor (i.e., a 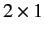 matrix of wavefunctions)
and
matrix of wavefunctions)
and 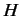 is a
is a 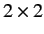 matrix partial differential operator [see Equation (507)].
The above spinor equation can always be written out explicitly as two
coupled partial differential equations for
matrix partial differential operator [see Equation (507)].
The above spinor equation can always be written out explicitly as two
coupled partial differential equations for 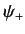 and
and 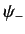 .
.
Suppose that the Hamiltonian has no dependence on the spin operators. In this
case, the Hamiltonian is represented as diagonal 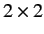 matrix partial
differential operator in the Schrödinger/Pauli scheme [see Equation (506)].
In other words, the partial differential equation for
matrix partial
differential operator in the Schrödinger/Pauli scheme [see Equation (506)].
In other words, the partial differential equation for 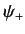 decouples
from that for
decouples
from that for 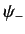 . In fact, both equations have the same form, so there
is only really one differential equation. In this
situation, the most general solution to Equation (523) can be written
. In fact, both equations have the same form, so there
is only really one differential equation. In this
situation, the most general solution to Equation (523) can be written
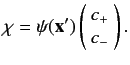 |
(524) |
Here,
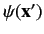 is determined by the solution of the differential equation,
and the
is determined by the solution of the differential equation,
and the 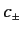 are arbitrary complex numbers. The physical significance of
the above expression is clear. The Hamiltonian determines the relative probabilities
of finding the particle at various different positions, but the direction
of its spin angular momentum remains undetermined.
are arbitrary complex numbers. The physical significance of
the above expression is clear. The Hamiltonian determines the relative probabilities
of finding the particle at various different positions, but the direction
of its spin angular momentum remains undetermined.
Suppose that the Hamiltonian depends only on the spin operators. In this
case, the Hamiltonian is represented as a 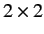 matrix of complex numbers
in the Schrödinger/Pauli scheme [see Equation (489)], and the spinor eigenvalue
equation (523) reduces to a straightforward matrix eigenvalue problem.
The most general solution can again be written
matrix of complex numbers
in the Schrödinger/Pauli scheme [see Equation (489)], and the spinor eigenvalue
equation (523) reduces to a straightforward matrix eigenvalue problem.
The most general solution can again be written
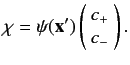 |
(525) |
Here, the ratio 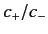 is determined by the matrix eigenvalue problem,
and the wavefunction
is determined by the matrix eigenvalue problem,
and the wavefunction
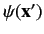 is arbitrary. Clearly, the Hamiltonian
determines the direction of the particle's spin angular momentum, but leaves
its position undetermined.
is arbitrary. Clearly, the Hamiltonian
determines the direction of the particle's spin angular momentum, but leaves
its position undetermined.
In general, of course, the Hamiltonian is a function of both position and
spin operators. In this case, it is not possible to decompose the
spinor as in Equations (524) and (525).
In other words, a general Hamiltonian causes the
direction of the particle's spin angular momentum to vary with position in
some specified manner. This can only be represented as a spinor involving
different wavefunctions, 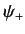 and
and 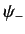 .
.
But, what happens if we have a spin one or a spin three-halves particle?
It turns out that we can generalize the Pauli two-component scheme in a fairly
straightforward manner. Consider a spin-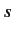 particle: i.e., a particle for which
the eigenvalue of
particle: i.e., a particle for which
the eigenvalue of 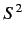 is
is
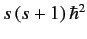 . Here,
. Here, 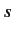 is either an integer, or a half-integer. The eigenvalues of
is either an integer, or a half-integer. The eigenvalues of 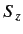 are written
are written
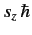 , where
, where
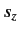 is allowed to take the values
is allowed to take the values
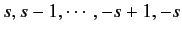 . In fact,
there are
. In fact,
there are  distinct allowed values of
distinct allowed values of 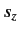 . Not surprisingly, we can represent
the state of the particle by
. Not surprisingly, we can represent
the state of the particle by  different wavefunctions, denoted
different wavefunctions, denoted
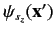 . Here,
. Here,
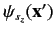 specifies the probability density
for observing the particle at position
specifies the probability density
for observing the particle at position 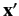 with spin angular
momentum
with spin angular
momentum
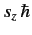 in the
in the 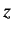 -direction. More exactly,
-direction. More exactly,
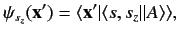 |
(526) |
where
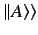 denotes a state ket in the product space of the position
and spin operators. The state of the particle can be represented more
succinctly by a spinor,
denotes a state ket in the product space of the position
and spin operators. The state of the particle can be represented more
succinctly by a spinor, 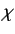 , which is simply the
, which is simply the  component column
vector of the
component column
vector of the
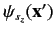 .
Thus, a spin one-half particle is represented by a two-component spinor,
a spin one particle by a three-component spinor, a spin three-halves particle
by a four-component spinor, and so on.
.
Thus, a spin one-half particle is represented by a two-component spinor,
a spin one particle by a three-component spinor, a spin three-halves particle
by a four-component spinor, and so on.
In this extended Schrödinger/Pauli
scheme, position space operators take the form of diagonal
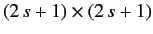 matrix differential operators. Thus, we can represent the momentum operators
as [see
Equation (506)]
matrix differential operators. Thus, we can represent the momentum operators
as [see
Equation (506)]
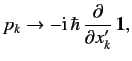 |
(527) |
where 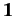 is the
is the
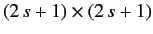 unit matrix.
We represent the spin
operators as
unit matrix.
We represent the spin
operators as
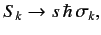 |
(528) |
where the
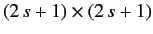 extended Pauli matrix
extended Pauli matrix 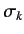 has elements
has elements
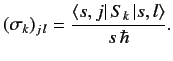 |
(529) |
Here, 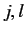 are integers, or half-integers, lying in the range
are integers, or half-integers, lying in the range 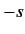 to
to  .
But, how can we evaluate the brackets
.
But, how can we evaluate the brackets
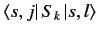 and, thereby, construct the extended Pauli matrices? In fact, it is trivial
to construct the
and, thereby, construct the extended Pauli matrices? In fact, it is trivial
to construct the 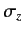 matrix. By definition,
matrix. By definition,
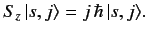 |
(530) |
Hence,
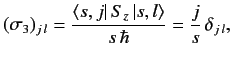 |
(531) |
where use has been made of the orthonormality property of the
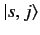 .
Thus,
.
Thus, 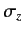 is the suitably normalized diagonal matrix of the eigenvalues
of
is the suitably normalized diagonal matrix of the eigenvalues
of 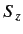 . The matrix elements of
. The matrix elements of 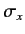 and
and 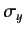 are most easily
obtained by considering the shift operators,
are most easily
obtained by considering the shift operators,
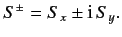 |
(532) |
We know, from Equations (344)-(345), that
It follows from Equations (529), and (532)-(534), that
According to Equations (531) and (535)-(536), the Pauli matrices for a spin one-half
(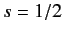 )
particle are
)
particle are
as we have seen previously. For a spin one (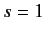 ) particle, we find that
) particle, we find that
In fact, we can now construct the Pauli matrices for a spin anything particle.
This means that we can convert the general energy eigenvalue problem for a spin-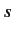 particle, where the Hamiltonian is some function of position and spin operators,
into
particle, where the Hamiltonian is some function of position and spin operators,
into  coupled partial differential equations involving the
coupled partial differential equations involving the
 wavefunctions
wavefunctions
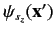 . Unfortunately, such a system
of equations is generally too complicated
to solve exactly.
. Unfortunately, such a system
of equations is generally too complicated
to solve exactly.



Next: Exercises
Up: Spin Angular Momentum
Previous: Pauli Two-Component Formalism
Richard Fitzpatrick
2013-04-08
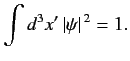
![]() , specifies the probability density of
observing the particle at position
, specifies the probability density of
observing the particle at position ![]() with spin angular momentum
with spin angular momentum ![]() in the
in the ![]() -direction. The second,
-direction. The second,
![]() , specifies the
probability density of
observing the particle at position
, specifies the
probability density of
observing the particle at position ![]() with spin angular momentum
with spin angular momentum ![]() in the
in the ![]() -direction. In the Pauli scheme, these wavefunctions
are combined into a spinor,
-direction. In the Pauli scheme, these wavefunctions
are combined into a spinor, ![]() , which is simply the column vector of
, which is simply the column vector of ![]() and
and ![]() .
In general, the Hamiltonian is a function of the position, momentum, and spin
operators. Adopting the Schrödinger representation, and the Pauli scheme,
the energy eigenvalue problem reduces to
.
In general, the Hamiltonian is a function of the position, momentum, and spin
operators. Adopting the Schrödinger representation, and the Pauli scheme,
the energy eigenvalue problem reduces to
![]() matrix partial
differential operator in the Schrödinger/Pauli scheme [see Equation (506)].
In other words, the partial differential equation for
matrix partial
differential operator in the Schrödinger/Pauli scheme [see Equation (506)].
In other words, the partial differential equation for ![]() decouples
from that for
decouples
from that for ![]() . In fact, both equations have the same form, so there
is only really one differential equation. In this
situation, the most general solution to Equation (523) can be written
. In fact, both equations have the same form, so there
is only really one differential equation. In this
situation, the most general solution to Equation (523) can be written
![]() matrix of complex numbers
in the Schrödinger/Pauli scheme [see Equation (489)], and the spinor eigenvalue
equation (523) reduces to a straightforward matrix eigenvalue problem.
The most general solution can again be written
matrix of complex numbers
in the Schrödinger/Pauli scheme [see Equation (489)], and the spinor eigenvalue
equation (523) reduces to a straightforward matrix eigenvalue problem.
The most general solution can again be written
![]() and
and ![]() .
.
![]() particle: i.e., a particle for which
the eigenvalue of
particle: i.e., a particle for which
the eigenvalue of ![]() is
is
![]() . Here,
. Here, ![]() is either an integer, or a half-integer. The eigenvalues of
is either an integer, or a half-integer. The eigenvalues of ![]() are written
are written
![]() , where
, where
![]() is allowed to take the values
is allowed to take the values
![]() . In fact,
there are
. In fact,
there are ![]() distinct allowed values of
distinct allowed values of ![]() . Not surprisingly, we can represent
the state of the particle by
. Not surprisingly, we can represent
the state of the particle by ![]() different wavefunctions, denoted
different wavefunctions, denoted
![]() . Here,
. Here,
![]() specifies the probability density
for observing the particle at position
specifies the probability density
for observing the particle at position ![]() with spin angular
momentum
with spin angular
momentum
![]() in the
in the ![]() -direction. More exactly,
-direction. More exactly,
![]() matrix differential operators. Thus, we can represent the momentum operators
as [see
Equation (506)]
matrix differential operators. Thus, we can represent the momentum operators
as [see
Equation (506)]