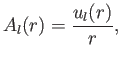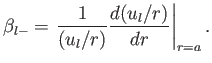Next: Hard-Sphere Scattering
Up: Scattering Theory
Previous: Optical Theorem
Let us now consider how the partial wave phase-shifts, 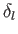 , can be
evaluated. Consider a spherically symmetric potential,
, can be
evaluated. Consider a spherically symmetric potential, 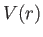 , that
vanishes for
, that
vanishes for 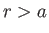 , where
, where 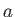 is termed the range of the potential.
In the region
is termed the range of the potential.
In the region 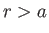 , the wavefunction
, the wavefunction
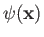 satisfies the free-space Schrödinger equation, (10.56). According to Equations (10.70),
(10.73), (10.74), and (10.78), the
most general solution of this equation that is consistent with no incoming spherical waves, other than those contained in the incident wave, is
satisfies the free-space Schrödinger equation, (10.56). According to Equations (10.70),
(10.73), (10.74), and (10.78), the
most general solution of this equation that is consistent with no incoming spherical waves, other than those contained in the incident wave, is
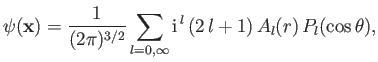 |
(10.93) |
where
![$\displaystyle A_l(r) = \exp(\,{\rm i} \,\delta_l)\, \left[ \cos\delta_l \,j_l(k\,r) -\sin\delta_l\, \eta_l(k\,r)\right].$](img3586.png) |
(10.94) |
Note that Neumann functions are allowed to appear in the previous
expression, because its region of validity does not include the origin
(where 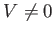 ). The logarithmic derivative of the
). The logarithmic derivative of the  th
radial wavefunction,
th
radial wavefunction,
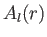 , just outside the range of the potential is given by
, just outside the range of the potential is given by
![$\displaystyle \beta_{l+} = k\,a \left[\frac{ \cos\delta_l\,j_l'(k\,a) - \sin\de...
... \eta_l'(k\,a)}{\cos\delta_l \, j_l(k\,a) - \sin\delta_l\,\eta_l(k\,a)}\right],$](img3589.png) |
(10.95) |
where 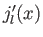 denotes
denotes
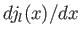 , et cetera. The previous equation
can be inverted to give
, et cetera. The previous equation
can be inverted to give
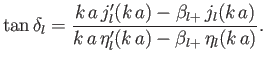 |
(10.96) |
Thus, the problem of determining the phase-shift, 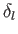 , is equivalent
to that of determining
, is equivalent
to that of determining
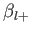 .
.
The most general solution to Schrödinger's equation inside
the range of the potential (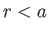 ) that does not depend on the
azimuthal angle,
) that does not depend on the
azimuthal angle, 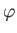 , is
, is
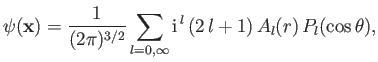 |
(10.97) |
where
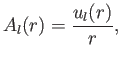 |
(10.98) |
and
![$\displaystyle \frac{d^{\,2} u_l}{d r^{\,2}} +\left[k^{\,2} - \frac{2\,m}{\hbar^{\,2}} \,V - \frac{l\,(l+1)} {r^{\,2}}\right] u_l = 0.$](img3596.png) |
(10.99) |
(See Exercise 12.)
The boundary condition
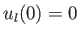 |
(10.100) |
ensures that the radial wavefunction is well behaved at the
origin.
We can launch a well-behaved solution of the previous equation from
 , integrate out to
, integrate out to  , and form the logarithmic derivative [of
, and form the logarithmic derivative [of 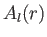 ]
]
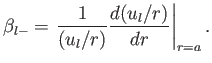 |
(10.101) |
Because
 and its first derivatives are necessarily continuous for
physically acceptable wavefunctions, it follows that
and its first derivatives are necessarily continuous for
physically acceptable wavefunctions, it follows that
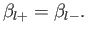 |
(10.102) |
The phase-shift, 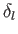 , is then obtained from Equation (10.97).
, is then obtained from Equation (10.97).



Next: Hard-Sphere Scattering
Up: Scattering Theory
Previous: Optical Theorem
Richard Fitzpatrick
2016-01-22
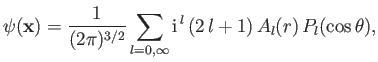
![$\displaystyle \beta_{l+} = k\,a \left[\frac{ \cos\delta_l\,j_l'(k\,a) - \sin\de...
... \eta_l'(k\,a)}{\cos\delta_l \, j_l(k\,a) - \sin\delta_l\,\eta_l(k\,a)}\right],$](img3589.png)
![]() ) that does not depend on the
azimuthal angle,
) that does not depend on the
azimuthal angle, ![]() , is
, is