Next: Magnetized Limit Up: Plasma Fluid Theory Previous: Braginskii Equations Contents
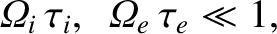 |
(4.80) |
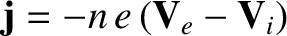 is the net plasma current density,
and the electrical conductivity,
is the net plasma current density,
and the electrical conductivity,
 , is given by
Moreover, use has been made of the conservation law,
Equation (4.32).
, is given by
Moreover, use has been made of the conservation law,
Equation (4.32).
Let us examine each of the previous collisional terms, one by one. The first
term on the right-hand side of Equation (4.81) is a friction force (per unit volume) caused by the
relative motion of electrons and ions, and obviously controls the electrical
conductivity of the plasma. The form of this term is fairly easy to understand.
The electrons lose their ordered velocity
with respect to the ions,
 ,
in an electron-ion collision time,
,
in an electron-ion collision time, 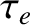 , and consequently lose momentum
, and consequently lose momentum
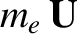 per electron (which is given to the ions) in this time.
This means that a frictional force
per electron (which is given to the ions) in this time.
This means that a frictional force
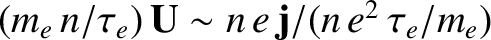 is exerted on the electrons.
An equal and opposite force is exerted on the ions. Because the Coulomb
cross-section diminishes with increasing electron energy (i.e.,
is exerted on the electrons.
An equal and opposite force is exerted on the ions. Because the Coulomb
cross-section diminishes with increasing electron energy (i.e.,
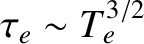 ),
the conductivity of the fast electrons in the distribution function
is higher than that of the slow electrons (because
),
the conductivity of the fast electrons in the distribution function
is higher than that of the slow electrons (because
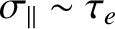 ).
Hence, electrical current in plasmas is carried predominately by the
fast electrons. This effect has some important and interesting
consequences.
).
Hence, electrical current in plasmas is carried predominately by the
fast electrons. This effect has some important and interesting
consequences.
One immediate consequence is the second term on the right-hand side of Equation (4.81),
which is called the thermal force. To understand the origin of
a frictional force (per unit volume) proportional to minus the gradient of the electron temperature,
let us assume that the electron and ion fluids are at rest (i.e.,
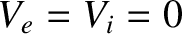 ). It follows that the number of electrons moving from left to right
(along the
). It follows that the number of electrons moving from left to right
(along the 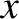 -axis, say) and from right to left per unit time is exactly the
same at a given point (coordinate
-axis, say) and from right to left per unit time is exactly the
same at a given point (coordinate 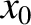 , say) in the plasma. As a result
of electron-ion collisions, these fluxes experience frictional forces,
, say) in the plasma. As a result
of electron-ion collisions, these fluxes experience frictional forces,
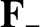 and
and 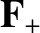 , respectively, of approximate magnitude
, respectively, of approximate magnitude
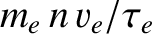 ,
where
,
where 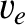 is the electron thermal velocity. In a completely homogeneous
plasma, these forces balance exactly, and so there is zero net frictional force.
Suppose, however, that the electrons coming from the right are, on average, hotter
than those coming from the left. It follows that the frictional force
is the electron thermal velocity. In a completely homogeneous
plasma, these forces balance exactly, and so there is zero net frictional force.
Suppose, however, that the electrons coming from the right are, on average, hotter
than those coming from the left. It follows that the frictional force
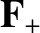 acting on the fast electrons coming from the right is less than
the force
acting on the fast electrons coming from the right is less than
the force 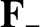 acting on the slow electrons coming from the left, because
acting on the slow electrons coming from the left, because
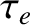 increases with electron temperature. As a result, there is a net
frictional force acting to the left: that is, in the direction of
increases with electron temperature. As a result, there is a net
frictional force acting to the left: that is, in the direction of
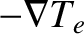 .
.
Let us estimate the magnitude of the frictional force. At point 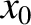 , collisions
are experienced by electrons that have traversed distances of similar magnitude to a
mean-free-path,
, collisions
are experienced by electrons that have traversed distances of similar magnitude to a
mean-free-path,
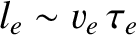 . Thus, the electrons coming from the
right originate from regions in which the temperature is approximately
. Thus, the electrons coming from the
right originate from regions in which the temperature is approximately
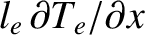 greater than the regions from which the electrons
coming from the left originate. Because the friction force is proportional to
greater than the regions from which the electrons
coming from the left originate. Because the friction force is proportional to
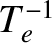 , the net force
, the net force
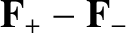 is approximately
is approximately
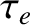 explicitly.
explicitly.
The term 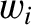 , specified in Equation (4.82), represents the rate (per unit volume) at which
energy is acquired by the ions due to collisions
with the electrons.
The most striking aspect of this term is
its smallness
(note that it is proportional to an inverse mass ratio,
, specified in Equation (4.82), represents the rate (per unit volume) at which
energy is acquired by the ions due to collisions
with the electrons.
The most striking aspect of this term is
its smallness
(note that it is proportional to an inverse mass ratio,
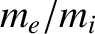 ). The smallness of
). The smallness of 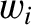 is a direct consequence of the
fact that electrons are considerably lighter than ions. Consider the
limit in which the ion mass is infinite, and the ions are at rest on average:
that is,
is a direct consequence of the
fact that electrons are considerably lighter than ions. Consider the
limit in which the ion mass is infinite, and the ions are at rest on average:
that is, 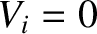 . In this case, collisions of electrons with ions
take place without any exchange of energy. The electron velocities
are randomized by the collisions, so that the energy associated
with their ordered velocity,
. In this case, collisions of electrons with ions
take place without any exchange of energy. The electron velocities
are randomized by the collisions, so that the energy associated
with their ordered velocity,
 , is converted
into heat energy in the electron fluid [this is represented by the second term
on the extreme right-hand side of Equation (4.83)]. However, the ion energy remains
unchanged. Let us now assume that the ratio
, is converted
into heat energy in the electron fluid [this is represented by the second term
on the extreme right-hand side of Equation (4.83)]. However, the ion energy remains
unchanged. Let us now assume that the ratio 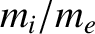 is large, but finite, and
that
is large, but finite, and
that 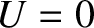 . If
. If 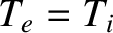 then the ions and electrons are in thermal equilibrium, so
no heat is exchanged between them. However, if
then the ions and electrons are in thermal equilibrium, so
no heat is exchanged between them. However, if 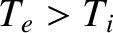 then heat
is transferred from the electrons to the ions. As is well known, when
a light particle collides with a heavy particle, the order of magnitude of the
transferred energy is given by the mass ratio
then heat
is transferred from the electrons to the ions. As is well known, when
a light particle collides with a heavy particle, the order of magnitude of the
transferred energy is given by the mass ratio 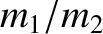 , where
, where 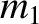 is the
mass of the lighter particle. For example, the mean fractional energy transferred
in isotropic scattering is
is the
mass of the lighter particle. For example, the mean fractional energy transferred
in isotropic scattering is
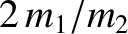 . Thus, we would expect the
energy per unit time transferred from the electrons to the ions to be roughly
. Thus, we would expect the
energy per unit time transferred from the electrons to the ions to be roughly
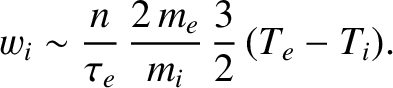 |
(4.86) |
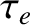 is defined so as to make the previous estimate exact.
is defined so as to make the previous estimate exact.
The term  , specified in Equation (4.83), represents the rate (per unit volume) at
which energy is acquired by the electrons because of
collisions with the ions, and consists of three terms. Not surprisingly,
the first term is simply minus the rate at which energy is
acquired by the ions due to collisions with the
electrons. The second term represents the conversion
of the ordered motion of the electrons, relative to the ions, into random
motion (i.e., heat) via collisions with the ions. This
term is positive definite, indicating that the randomization of the electron
ordered motion gives rise to irreversible heat generation.
Incidentally, this
term is usually called the ohmic heating term. Finally, the third
term represents the work done against the thermal force. This
term can be either positive or negative, depending on the direction of
the current flow relative to the electron temperature gradient, which
indicates that work done against the thermal force gives rise to reversible
heat generation. There is an analogous effect in metals called the Thomson effect (Doolittle 1959).
, specified in Equation (4.83), represents the rate (per unit volume) at
which energy is acquired by the electrons because of
collisions with the ions, and consists of three terms. Not surprisingly,
the first term is simply minus the rate at which energy is
acquired by the ions due to collisions with the
electrons. The second term represents the conversion
of the ordered motion of the electrons, relative to the ions, into random
motion (i.e., heat) via collisions with the ions. This
term is positive definite, indicating that the randomization of the electron
ordered motion gives rise to irreversible heat generation.
Incidentally, this
term is usually called the ohmic heating term. Finally, the third
term represents the work done against the thermal force. This
term can be either positive or negative, depending on the direction of
the current flow relative to the electron temperature gradient, which
indicates that work done against the thermal force gives rise to reversible
heat generation. There is an analogous effect in metals called the Thomson effect (Doolittle 1959).
The electron and ion heat flux densities are given by
respectively. The electron and ion thermal conductivities are written respectively.
It follows, by comparison with Equations (4.63)–(4.68), that
the first term on the right-hand side of Equation (4.87), as well as the expression
on the right-hand side of Equation (4.88), represent straightforward
random-walk heat diffusion, with frequency 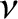 , and step-length
, and step-length 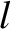 .
Recall, that
.
Recall, that
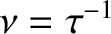 is the collision frequency, and
is the collision frequency, and
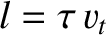 is the mean-free-path. The
electron heat diffusivity is generally much greater than that of the ions,
because
is the mean-free-path. The
electron heat diffusivity is generally much greater than that of the ions,
because
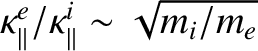 ,
assuming that
,
assuming that
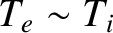 .
.
The second term on the right-hand side of Equation (4.87) describes a convective
heat flux due to the motion of the electrons relative to the ions.
To understand the origin of this flux, we need to recall that
electric current in plasmas is carried predominately by the fast electrons
in the distribution function. Suppose that 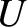 is non-zero. In the
coordinate system in which
is non-zero. In the
coordinate system in which 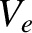 is zero, more fast electrons move in the
direction of
is zero, more fast electrons move in the
direction of 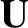 , and more slow electrons move in the opposite
direction. Although the electron fluxes are balanced in this frame of reference,
the energy fluxes are not (because a fast electron possesses more energy than a slow
electron), and heat flows in the direction of
, and more slow electrons move in the opposite
direction. Although the electron fluxes are balanced in this frame of reference,
the energy fluxes are not (because a fast electron possesses more energy than a slow
electron), and heat flows in the direction of 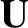 : that is, in
the opposite direction to the electric current. The net heat flux density is of
approximate magnitude
: that is, in
the opposite direction to the electric current. The net heat flux density is of
approximate magnitude 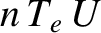 , because there is no near cancellation of the fluxes
due to the fast and slow electrons. Like the thermal force, this effect
depends on collisions, despite the fact that the expression for the convective
heat flux does not contain
, because there is no near cancellation of the fluxes
due to the fast and slow electrons. Like the thermal force, this effect
depends on collisions, despite the fact that the expression for the convective
heat flux does not contain 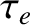 explicitly.
explicitly.
Finally, the electron and ion viscosity tensors take the form
respectively. Obviously,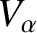 refers to a Cartesian component of the
electron fluid velocity in Equation (4.91) and the ion fluid velocity in Equation (4.92).
Here, the electron and ion viscosities are given
by
respectively.
It follows, by comparison with Equations (4.62)–(4.68), that the previous expressions
correspond to straightforward random-walk diffusion of momentum, with
frequency
refers to a Cartesian component of the
electron fluid velocity in Equation (4.91) and the ion fluid velocity in Equation (4.92).
Here, the electron and ion viscosities are given
by
respectively.
It follows, by comparison with Equations (4.62)–(4.68), that the previous expressions
correspond to straightforward random-walk diffusion of momentum, with
frequency 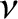 , and step-length
, and step-length 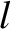 . Again, the electron diffusivity
exceeds the ion diffusivity by the square root of a mass ratio (assuming
. Again, the electron diffusivity
exceeds the ion diffusivity by the square root of a mass ratio (assuming
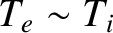 ). However,
the ion viscosity exceeds the electron viscosity by the same factor (recall
that
). However,
the ion viscosity exceeds the electron viscosity by the same factor (recall
that
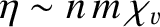 ): that is,
): that is,
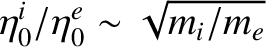 .
For this reason, the viscosity of a plasma is determined essentially by the
ions. This is not surprising, because viscosity is the diffusion of momentum,
and the ions possess nearly all of the momentum in a plasma by virtue of
their large masses.
.
For this reason, the viscosity of a plasma is determined essentially by the
ions. This is not surprising, because viscosity is the diffusion of momentum,
and the ions possess nearly all of the momentum in a plasma by virtue of
their large masses.