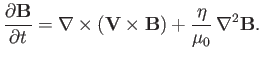 |
(7.169) |
The evolution of the magnetic field in a resistive-MHD plasma is governed by the following well-known equation [see Equation (7.102)]:
The relative magnitude of the two terms on the right-hand side of Equation (7.169) is conventionally measured in terms of magnetic Reynolds number, or Lundquist number:
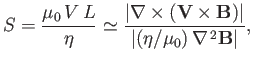 |
(7.170) |
It turns out that very large ![]() -values are virtually guaranteed to occur in the solar system because of
the extremely large lengthscales of solar system plasmas. For instance,
-values are virtually guaranteed to occur in the solar system because of
the extremely large lengthscales of solar system plasmas. For instance,
![]() for solar flares, whereas
for solar flares, whereas
![]() is appropriate for
the solar wind and the Earth's magnetosphere (Priest 1984). Of course, in calculating
these values, we have identified the lengthscale
is appropriate for
the solar wind and the Earth's magnetosphere (Priest 1984). Of course, in calculating
these values, we have identified the lengthscale ![]() with the characteristic
size of the plasma under investigation.
with the characteristic
size of the plasma under investigation.
On the basis of the previous discussion, it seems reasonable to neglect diffusive processes altogether in solar system plasmas. Of course, this leads to very strong constraints on the behavior of such plasmas, because, in this limit, all cross-field mixing of plasma elements is suppressed. Particles may freely mix along field-lines (within limitations imposed by magnetic mirroring, etc.), but are completely ordered perpendicular to the field, because they always remain tied to the same field-lines as they convect in the plasma flow.
Let us consider what happens when two initially separate plasma regions come into contact with one another, as occurs, for example, in the interaction between the solar wind and the Earth's magnetic field. Assuming that each plasma is frozen to its own magnetic field, and that cross-field diffusion is absent, we conclude that the two plasmas will not mix, but, instead, that a thin boundary layer will form between them, separating the two plasmas and their respective magnetic fields. In equilibrium, the location of the boundary layer will be determined by pressure balance. Because, in general, the frozen fields on either side of the boundary will have differing strengths, and differing orientations tangential to the boundary, the layer must also constitute a current sheet. Thus, flux freezing leads inevitably to the prediction that in plasma systems space becomes divided into separate cells, wholly containing the plasma and magnetic field from individual sources, and separated from each other by thin current sheets.
The ``separate cell'' picture constitutes an excellent zeroth-order approximation to
the interaction of solar system plasmas, as witnessed, for example, by
the well-defined planetary magnetospheres (Russell 1991). It must be noted, however, that
the large ![]() -values upon which the applicability of the frozen flux constraint
was justified were derived using the large overall spatial scales of the
systems involved. However, strict application of this constraint to the
problem of the interaction of separate plasma systems leads to the
inevitable conclusion
that structures will form having small spatial scales, at least in
one dimension: that is, the thin current sheets constituting the cell
boundaries. It is certainly not guaranteed, from the previous discussion, that
the effects of diffusion can be neglected in
these boundary layers. In fact, we shall demonstrate that
the localized breakdown of the flux freezing constraint in the boundary
regions, due to diffusion, not only has an impact on the
properties of the boundary regions themselves, but can also have a decisive
impact on the large lengthscale plasma regions in which the flux freezing
constraint remains valid. This observation illustrates both the subtlety and the
significance of the magnetic reconnection process.
-values upon which the applicability of the frozen flux constraint
was justified were derived using the large overall spatial scales of the
systems involved. However, strict application of this constraint to the
problem of the interaction of separate plasma systems leads to the
inevitable conclusion
that structures will form having small spatial scales, at least in
one dimension: that is, the thin current sheets constituting the cell
boundaries. It is certainly not guaranteed, from the previous discussion, that
the effects of diffusion can be neglected in
these boundary layers. In fact, we shall demonstrate that
the localized breakdown of the flux freezing constraint in the boundary
regions, due to diffusion, not only has an impact on the
properties of the boundary regions themselves, but can also have a decisive
impact on the large lengthscale plasma regions in which the flux freezing
constraint remains valid. This observation illustrates both the subtlety and the
significance of the magnetic reconnection process.