


Next: Poisson's Equation in Spherical
Up: Potential Theory
Previous: Spherical Harmonics
Laplace's Equation in Spherical Coordinates
Consider the general solution to Laplace's equation,
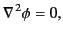 |
(322) |
in spherical coordinates. Let us write
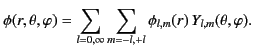 |
(323) |
It follows from Equation (308) that
![$\displaystyle \sum_{l=0,\infty}\sum_{m=-l,+l}\left[\frac{d}{dr}\!\left(r^{\,2}\...
...c{d\phi_{l,m}}{dr}\right)-l\,(l+1)\,\phi_{l,m}\right]Y_{l,m}(\theta,\varphi)=0.$](img735.png) |
(324) |
However, given that the spherical harmonics are mutually orthogonal [in the sense that they satisfy Equation (311)], we can separately equate the coefficients of each
in the above equation, to give
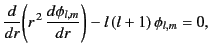 |
(325) |
for all  and
and 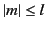 . It follows that
. It follows that
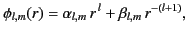 |
(326) |
where the
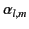 and
and
 are arbitrary constants. Hence, the general solution to Laplace's equation in spherical
coordinates is written
are arbitrary constants. Hence, the general solution to Laplace's equation in spherical
coordinates is written
![$\displaystyle \phi(r,\theta,\varphi)= \sum_{l=0,\infty}\sum_{m=-l,+l}\left[\alpha_{l,m}\,r^{\,l}+\beta_{l,m}\,r^{-(l+1)}\right]Y_{l,m}(\theta,\varphi).$](img742.png) |
(327) |
If the domain of solution includes the origin then all of the
 must be zero, in order to ensure that the potential
remains finite at
must be zero, in order to ensure that the potential
remains finite at 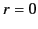 . On the other hand, if the domain of solution extends to infinity then all of the
. On the other hand, if the domain of solution extends to infinity then all of the
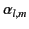 (except
(except
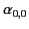 )
must be zero, otherwise the potential would be infinite at
)
must be zero, otherwise the potential would be infinite at 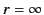 .
.



Next: Poisson's Equation in Spherical
Up: Potential Theory
Previous: Spherical Harmonics
Richard Fitzpatrick
2014-06-27
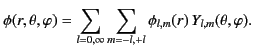
![$\displaystyle \sum_{l=0,\infty}\sum_{m=-l,+l}\left[\frac{d}{dr}\!\left(r^{\,2}\...
...c{d\phi_{l,m}}{dr}\right)-l\,(l+1)\,\phi_{l,m}\right]Y_{l,m}(\theta,\varphi)=0.$](img735.png)
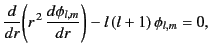
![$\displaystyle \phi(r,\theta,\varphi)= \sum_{l=0,\infty}\sum_{m=-l,+l}\left[\alpha_{l,m}\,r^{\,l}+\beta_{l,m}\,r^{-(l+1)}\right]Y_{l,m}(\theta,\varphi).$](img742.png)