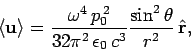Next: Thompson scattering
Up: Electromagnetic radiation
Previous: The Hertzian dipole
In the previous section, we examined the radiation emitted by a short electric dipole of oscillating dipole moment
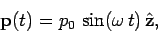 |
(1116) |
where
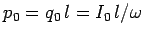 . We found that, in the far field, the mean
electromagnetic energy flux takes the form [see Eq. (1094)]
. We found that, in the far field, the mean
electromagnetic energy flux takes the form [see Eq. (1094)]
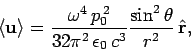 |
(1117) |
assuming that the dipole is centered on the origin of our spherical polar coordinate system.
The mean power radiated into the element of solid angle
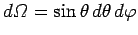 , centered on the angular coordinates (
, centered on the angular coordinates (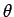 ,
, 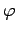 ),
is
),
is
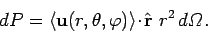 |
(1118) |
Hence, the differential power radiated into this element of solid angle is
simply
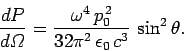 |
(1119) |
This formula completely specifies the radiation pattern of an oscillating electric dipole (provided that the dipole is much shorter in length than the
wave-length of the emitted radiation). Of course, the power radiated into
a given element of solid angle is independent of 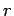 , otherwise energy would not be conserved. Finally, the total radiated power is the integral of
, otherwise energy would not be conserved. Finally, the total radiated power is the integral of
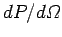 over all solid angles.
over all solid angles.



Next: Thompson scattering
Up: Electromagnetic radiation
Previous: The Hertzian dipole
Richard Fitzpatrick
2006-02-02