Euler's equations
The fundamental equation of motion of a rotating body [see Equation (8.3)],
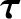 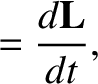 |
(8.23) |
is only valid in an inertial frame. However, we have seen that 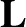 is most simply expressed in a frame of reference whose axes are aligned
along the principal axes of rotation of the body. Such a frame of reference
rotates with the body, and is, therefore, non-inertial. Thus, it is helpful to define two Cartesian coordinate systems with the same origins. The first,
with coordinates
is most simply expressed in a frame of reference whose axes are aligned
along the principal axes of rotation of the body. Such a frame of reference
rotates with the body, and is, therefore, non-inertial. Thus, it is helpful to define two Cartesian coordinate systems with the same origins. The first,
with coordinates  ,
,  ,
,  , is a fixed inertial frame; let us denote
this the fixed frame. The second, with coordinates
, is a fixed inertial frame; let us denote
this the fixed frame. The second, with coordinates 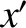 ,
, 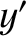 ,
, 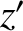 ,
co-rotates with the body in such a manner that the
,
co-rotates with the body in such a manner that the 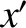 -,
-, 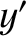 -, and
-, and 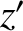 -axes are always pointing along its principal axes of rotation; we shall
refer to this as the body frame. Because the body frame co-rotates with the body, its instantaneous angular velocity is the same as that of the
body. Hence, it follows from the analysis in Section 6.2 that
Here,
-axes are always pointing along its principal axes of rotation; we shall
refer to this as the body frame. Because the body frame co-rotates with the body, its instantaneous angular velocity is the same as that of the
body. Hence, it follows from the analysis in Section 6.2 that
Here, 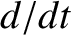 is the time derivative in the fixed frame, and
is the time derivative in the fixed frame, and 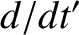 the
time derivative in the body frame.
Combining Equations (8.23) and (8.24), we obtain
In the body frame, let
the
time derivative in the body frame.
Combining Equations (8.23) and (8.24), we obtain
In the body frame, let
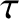
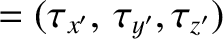 and
and

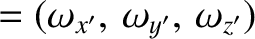 .
It follows that
.
It follows that
 |
(8.26) |
where
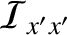 ,
,
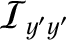 and
and
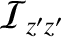 are the principal
moments of inertia. Hence, in the body frame, the components of Equation (8.25) yield
where
are the principal
moments of inertia. Hence, in the body frame, the components of Equation (8.25) yield
where
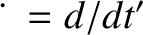 .
Here, we have made use of the fact that the moments of inertia
of a rigid body are constant in time in the co-rotating body frame.
The preceding three equations are known as Euler's equations.
.
Here, we have made use of the fact that the moments of inertia
of a rigid body are constant in time in the co-rotating body frame.
The preceding three equations are known as Euler's equations.
Consider a body that is freely rotating; that is, in the absence of external
torques. Furthermore, let the body be rotationally symmetric
about the 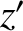 -axis. It follows that
-axis. It follows that
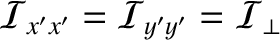 .
Likewise, we can write
.
Likewise, we can write
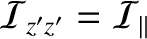 . In general, however,
. In general, however,
 . Thus, Euler's equations yield
. Thus, Euler's equations yield
Clearly,
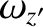 is a constant of the motion.
Equation (8.30) and (8.31) can be written
where
is a constant of the motion.
Equation (8.30) and (8.31) can be written
where
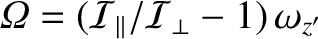 . As is easily
demonstrated, the solution to these equations is
where
. As is easily
demonstrated, the solution to these equations is
where
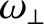 is a constant. Thus, the projection of the
angular velocity vector onto the
is a constant. Thus, the projection of the
angular velocity vector onto the 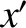 -
-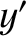 plane has the fixed length
plane has the fixed length
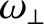 , and rotates steadily about the
, and rotates steadily about the 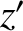 -axis with angular
velocity
-axis with angular
velocity
 .
It follows that the length of the angular
velocity vector,
.
It follows that the length of the angular
velocity vector,
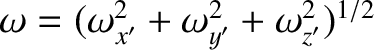 , is a constant of the motion.
Clearly, the angular
velocity vector subtends some constant angle,
, is a constant of the motion.
Clearly, the angular
velocity vector subtends some constant angle, 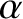 , with the
, with the 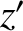 -axis, which implies that
-axis, which implies that
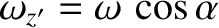 and
and
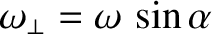 .
Hence, the components of the angular velocity vector are
where
.
Hence, the components of the angular velocity vector are
where
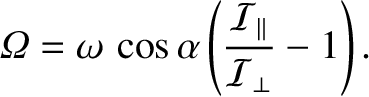 |
(8.40) |
We conclude that, in the body frame, the angular velocity vector precesses about the
symmetry axis (i.e., the 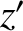 -axis) with the angular
frequency
-axis) with the angular
frequency
 . Now, the components of the angular momentum vector are
Thus, in the body frame, the angular momentum vector is also of constant length, and
precesses about the symmetry axis with the angular frequency
. Now, the components of the angular momentum vector are
Thus, in the body frame, the angular momentum vector is also of constant length, and
precesses about the symmetry axis with the angular frequency
 . Furthermore, the angular momentum vector subtends a constant angle
. Furthermore, the angular momentum vector subtends a constant angle  with the symmetry
axis, where
with the symmetry
axis, where
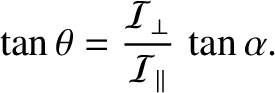 |
(8.44) |
The angular momentum vector, the angular velocity vector, and
the symmetry axis all lie in the same plane;
that is,
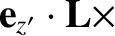

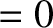 ,
as can easily be verified. Moreover, the
angular momentum vector lies between the angular velocity vector and
the symmetry axis (i.e.,
,
as can easily be verified. Moreover, the
angular momentum vector lies between the angular velocity vector and
the symmetry axis (i.e.,
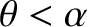 ) for a flattened (or oblate) body
(i.e.,
) for a flattened (or oblate) body
(i.e.,
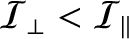 ), whereas the angular velocity vector lies
between the angular momentum vector and the symmetry axis (i.e.,
), whereas the angular velocity vector lies
between the angular momentum vector and the symmetry axis (i.e.,
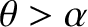 ) for an elongated (or prolate) body (i.e.,
) for an elongated (or prolate) body (i.e.,
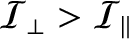 ). See Figure 8.2.
). See Figure 8.2.
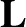 is most simply expressed in a frame of reference whose axes are aligned
along the principal axes of rotation of the body. Such a frame of reference
rotates with the body, and is, therefore, non-inertial. Thus, it is helpful to define two Cartesian coordinate systems with the same origins. The first,
with coordinates
is most simply expressed in a frame of reference whose axes are aligned
along the principal axes of rotation of the body. Such a frame of reference
rotates with the body, and is, therefore, non-inertial. Thus, it is helpful to define two Cartesian coordinate systems with the same origins. The first,
with coordinates  ,
,  ,
,  , is a fixed inertial frame; let us denote
this the fixed frame. The second, with coordinates
, is a fixed inertial frame; let us denote
this the fixed frame. The second, with coordinates 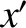 ,
, 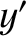 ,
, 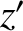 ,
co-rotates with the body in such a manner that the
,
co-rotates with the body in such a manner that the 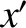 -,
-, 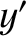 -, and
-, and 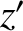 -axes are always pointing along its principal axes of rotation; we shall
refer to this as the body frame. Because the body frame co-rotates with the body, its instantaneous angular velocity is the same as that of the
body. Hence, it follows from the analysis in Section 6.2 that
Here,
-axes are always pointing along its principal axes of rotation; we shall
refer to this as the body frame. Because the body frame co-rotates with the body, its instantaneous angular velocity is the same as that of the
body. Hence, it follows from the analysis in Section 6.2 that
Here, 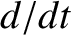 is the time derivative in the fixed frame, and
is the time derivative in the fixed frame, and 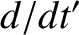 the
time derivative in the body frame.
Combining Equations (8.23) and (8.24), we obtain
In the body frame, let
the
time derivative in the body frame.
Combining Equations (8.23) and (8.24), we obtain
In the body frame, let
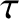
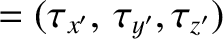 and
and

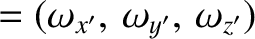 .
It follows that
.
It follows that

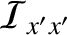 ,
,
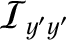 and
and
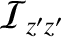 are the principal
moments of inertia. Hence, in the body frame, the components of Equation (8.25) yield
where
are the principal
moments of inertia. Hence, in the body frame, the components of Equation (8.25) yield
where
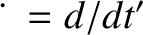 .
Here, we have made use of the fact that the moments of inertia
of a rigid body are constant in time in the co-rotating body frame.
The preceding three equations are known as Euler's equations.
.
Here, we have made use of the fact that the moments of inertia
of a rigid body are constant in time in the co-rotating body frame.
The preceding three equations are known as Euler's equations.
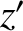 -axis. It follows that
-axis. It follows that
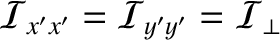 .
Likewise, we can write
.
Likewise, we can write
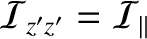 . In general, however,
. In general, however,
 . Thus, Euler's equations yield
. Thus, Euler's equations yield
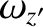 is a constant of the motion.
Equation (8.30) and (8.31) can be written
is a constant of the motion.
Equation (8.30) and (8.31) can be written
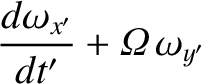
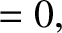
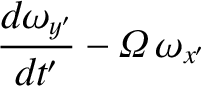
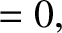
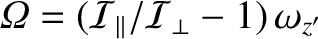 . As is easily
demonstrated, the solution to these equations is
. As is easily
demonstrated, the solution to these equations is
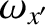
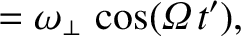
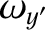
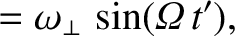
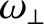 is a constant. Thus, the projection of the
angular velocity vector onto the
is a constant. Thus, the projection of the
angular velocity vector onto the 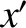 -
-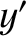 plane has the fixed length
plane has the fixed length
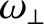 , and rotates steadily about the
, and rotates steadily about the 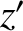 -axis with angular
velocity
-axis with angular
velocity
 .
It follows that the length of the angular
velocity vector,
.
It follows that the length of the angular
velocity vector,
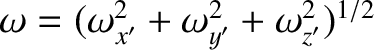 , is a constant of the motion.
Clearly, the angular
velocity vector subtends some constant angle,
, is a constant of the motion.
Clearly, the angular
velocity vector subtends some constant angle, 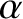 , with the
, with the 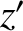 -axis, which implies that
-axis, which implies that
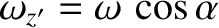 and
and
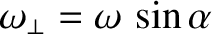 .
Hence, the components of the angular velocity vector are
.
Hence, the components of the angular velocity vector are
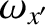
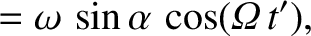
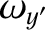
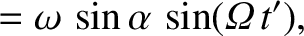
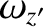

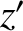 -axis) with the angular
frequency
-axis) with the angular
frequency
 . Now, the components of the angular momentum vector are
. Now, the components of the angular momentum vector are
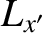
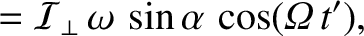

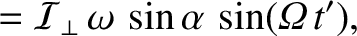
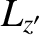
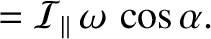
 . Furthermore, the angular momentum vector subtends a constant angle
. Furthermore, the angular momentum vector subtends a constant angle  with the symmetry
axis, where
The angular momentum vector, the angular velocity vector, and
the symmetry axis all lie in the same plane;
that is,
with the symmetry
axis, where
The angular momentum vector, the angular velocity vector, and
the symmetry axis all lie in the same plane;
that is,
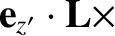

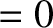 ,
as can easily be verified. Moreover, the
angular momentum vector lies between the angular velocity vector and
the symmetry axis (i.e.,
,
as can easily be verified. Moreover, the
angular momentum vector lies between the angular velocity vector and
the symmetry axis (i.e.,
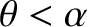 ) for a flattened (or oblate) body
(i.e.,
) for a flattened (or oblate) body
(i.e.,
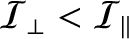 ), whereas the angular velocity vector lies
between the angular momentum vector and the symmetry axis (i.e.,
), whereas the angular velocity vector lies
between the angular momentum vector and the symmetry axis (i.e.,
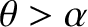 ) for an elongated (or prolate) body (i.e.,
) for an elongated (or prolate) body (i.e.,
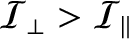 ). See Figure 8.2.
). See Figure 8.2.