Next: Kepler's third law Up: Keplerian orbits Previous: Kepler's second law
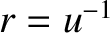 , where
, where
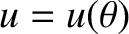 and
and
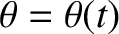 . It follows that
Likewise,
. It follows that
Likewise,
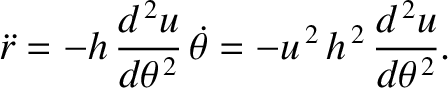 |
(4.27) |
 and
and 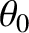 are arbitrary constants. Without loss of generality, we can
set
are arbitrary constants. Without loss of generality, we can
set
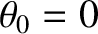 by rotating our coordinate system about the
by rotating our coordinate system about the  -axis. We can also assume that
-axis. We can also assume that 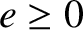 . Thus,
we obtain
where
Equation (4.30) is the equation of a conic
section that is confocal with the origin (i.e., with the Sun). (See Section A.9.)
Specifically, for
. Thus,
we obtain
where
Equation (4.30) is the equation of a conic
section that is confocal with the origin (i.e., with the Sun). (See Section A.9.)
Specifically, for 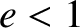 , Equation (4.30) is the equation of an ellipse; for
, Equation (4.30) is the equation of an ellipse; for 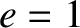 , Equation (4.30) is the equation of a parabola; finally, for
, Equation (4.30) is the equation of a parabola; finally, for 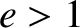 , Equation (4.30) is the equation of a hyperbola. However, a planet cannot have a parabolic
or a hyperbolic orbit, because such orbits are only appropriate to objects that are ultimately able to escape from the Sun's gravitational field.
Thus, the orbit of our planet
is an ellipse that is confocal with the Sun; this is Kepler's first law
of planetary motion
, Equation (4.30) is the equation of a hyperbola. However, a planet cannot have a parabolic
or a hyperbolic orbit, because such orbits are only appropriate to objects that are ultimately able to escape from the Sun's gravitational field.
Thus, the orbit of our planet
is an ellipse that is confocal with the Sun; this is Kepler's first law
of planetary motion