Next: Rotational Kinetic Energy Up: Rigid Body Rotation Previous: Fundamental Equations
 about
an axis that passes through the origin. See Figure 1.8.
Let
about
an axis that passes through the origin. See Figure 1.8.
Let  be the displacement of the
be the displacement of the  th mass element, whose mass
is
th mass element, whose mass
is 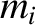 .
We expect this displacement to precess about the axis of rotation
(which is parallel to
.
We expect this displacement to precess about the axis of rotation
(which is parallel to
 )
with angular velocity
)
with angular velocity  . It, therefore, follows from Equation (A.52)
that
. It, therefore, follows from Equation (A.52)
that
The total angular momentum of the body (about the origin) is written
where use has been made of Equation (1.181), and some standard vector identities. (See Section A.11.) The previous formula can be written as a matrix equation of the form where Here,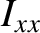 is called the moment of inertia about the
is called the moment of inertia about the  -axis,
-axis,
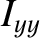 the moment of inertia about the
the moment of inertia about the  -axis,
-axis,  the
the  -
- product of inertia,
product of inertia, 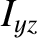 the
the  -
- product of
inertia, et cetera. The matrix of the
product of
inertia, et cetera. The matrix of the 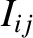 values is
known as the moment of inertia tensor.
values is
known as the moment of inertia tensor.
Suppose that our body is rotationally symmetric about the  -axis. In this case, it is easily seen that
the products of inertia are all zero. Moreover,
-axis. In this case, it is easily seen that
the products of inertia are all zero. Moreover,
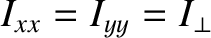 . Let us write
. Let us write
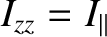 . Note that,
in general,
. Note that,
in general,
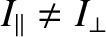 (unless the body is spherically symmetric). Thus, Equation (1.183)
simplifies to give
(unless the body is spherically symmetric). Thus, Equation (1.183)
simplifies to give
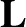 , obtained from the previous equation,
does not necessarily point in the same direction as the angular velocity vector,
, obtained from the previous equation,
does not necessarily point in the same direction as the angular velocity vector,
 (because
(because
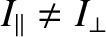 ). In other words,
). In other words, 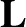 is generally not parallel to
is generally not parallel to
 . However, if the body rotates about
. However, if the body rotates about 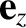 or any axis in the
or any axis in the  -
- plane then
plane then 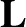 is parallel to
is parallel to
 . These special axes of rotation are called principal
axes of rotation, and the associated moments of inertia,
. These special axes of rotation are called principal
axes of rotation, and the associated moments of inertia,
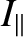 and
and 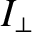 , respectively, are called principal moments of inertia.
, respectively, are called principal moments of inertia.
It can be demonstrated that any rigid body (not just an axisymmetric one) has three mutually perpendicular principal axes of rotation. Furthermore, if a body is rotating about one of its principal axes of rotation then
where is the associated principal moment of inertia. More generally, assuming that the
Cartesian axis are parallel to the principal moments of inertia, we can write
is the associated principal moment of inertia. More generally, assuming that the
Cartesian axis are parallel to the principal moments of inertia, we can write
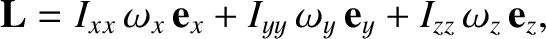 |
(1.192) |
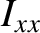 ,
, 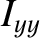 , and
, and 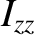 are the three principal moments of inertia.
are the three principal moments of inertia.