Next: Stefan-Boltzmann Law Up: Standing-Wave States Previous: Planck Radiation Law
The power density inside the enclosure can be written
 |
(5.472) |
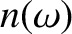 is the mean
number of photons per unit volume whose frequencies lie
in the range
is the mean
number of photons per unit volume whose frequencies lie
in the range  to
to
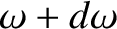 . The radiation field inside the
enclosure is isotropic (we are assuming that the hole is sufficiently small that
it does not distort the field). It follows that the mean number of photons
per unit volume
whose frequencies lie in the specified range, and
whose directions of propagation subtend an angle in the range
. The radiation field inside the
enclosure is isotropic (we are assuming that the hole is sufficiently small that
it does not distort the field). It follows that the mean number of photons
per unit volume
whose frequencies lie in the specified range, and
whose directions of propagation subtend an angle in the range
 to
to
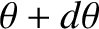 with the normal to the hole, is
with the normal to the hole, is
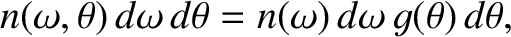 |
(5.473) |
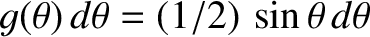 is the fractional range of solid angle in the specified
range of directions. (See Section 5.3.2.)
The previous two equations give
is the fractional range of solid angle in the specified
range of directions. (See Section 5.3.2.)
The previous two equations give
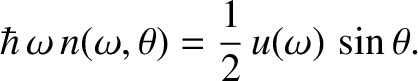 |
(5.474) |
Photons travel at the speed of light, so the power per unit area escaping from
the hole in the frequency range  to
to
 is
is
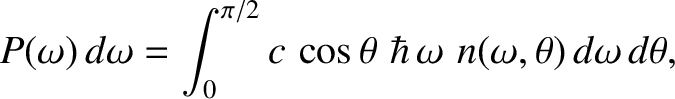 |
(5.475) |
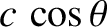 is the component of the photon velocity in the direction
of the hole.
This gives
is the component of the photon velocity in the direction
of the hole.
This gives
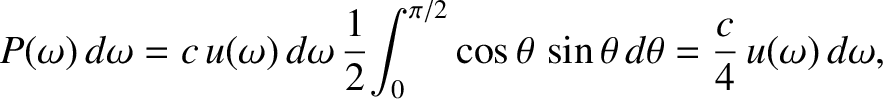 |
(5.476) |
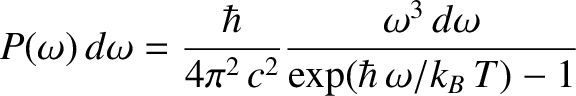 |
(5.477) |
 to
to
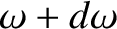 .
.
A black-body is very much an idealization. The power
spectra of real radiating bodies
can deviate quite substantially from black-body spectra. Nevertheless, we
can make some useful predictions using this model. The black-body power spectrum
peaks when
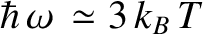 , implying that the peak radiation
frequency scales linearly with the temperature of the body. In other words,
hot bodies tend to radiate at higher frequencies than cold bodies. This
result (in particular, the linear scaling) is known as Wien's displacement
law, after Wilhelm Wein who derived it in 1893, and allows us to estimate the surface temperatures of stars from their
colors (surprisingly enough, stars are fairly good black-bodies). Table 5.2 shows
some stellar temperatures determined by this method (in fact,
the whole emission spectrum is fitted to a black-body spectrum).
It can be seen that the
apparent colors (which correspond quite well to the colors of the peak radiation)
scan the whole visible spectrum, from red to blue, as the stellar surface temperatures
gradually rise.
, implying that the peak radiation
frequency scales linearly with the temperature of the body. In other words,
hot bodies tend to radiate at higher frequencies than cold bodies. This
result (in particular, the linear scaling) is known as Wien's displacement
law, after Wilhelm Wein who derived it in 1893, and allows us to estimate the surface temperatures of stars from their
colors (surprisingly enough, stars are fairly good black-bodies). Table 5.2 shows
some stellar temperatures determined by this method (in fact,
the whole emission spectrum is fitted to a black-body spectrum).
It can be seen that the
apparent colors (which correspond quite well to the colors of the peak radiation)
scan the whole visible spectrum, from red to blue, as the stellar surface temperatures
gradually rise.
Probably the most famous black-body spectrum is cosmological in origin. Just after
the “big bang,” the universe was essentially a “fireball,” with the energy associated with
radiation completely dominating that associated with
matter. The early universe was also fairly
well described by equilibrium statistical thermodynamics,
which means that the radiation had a black-body spectrum. As the universe expanded,
the radiation was gradually Doppler shifted to ever larger wavelengths (in other
words, the radiation did work against the expansion of the universe, and, thereby,
lost energy, but its spectrum remained invariant). Nowadays, this primordial
radiation is detectable as a faint microwave background that pervades the
whole universe. The cosmic microwave background was discovered accidentally by Arno Penzias
and Robert Wilson in 1964. For many years, it was difficult to measure the full
spectrum of the microwave background with any degree of
precision, because of strong absorption and scattering of microwaves by
the Earth's atmosphere. However, all of this changed when the COBE satellite
was launched in 1989. It took precisely nine minutes to measure the perfect
black-body spectrum reproduced in Figure 5.8.
The data shown in the figure can be fitted to a black-body
curve of characteristic
temperature 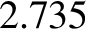 K. In a very real sense, this can be regarded
as the “temperature of the universe.”
K. In a very real sense, this can be regarded
as the “temperature of the universe.”
![\includegraphics[width=0.85\textwidth]{Chapter06/cobe1.eps}](img4357.png) |