Next: Specific Heats of Gases Up: Applications of Statistical Mechanics Previous: Harmonic Oscillator
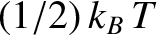 in thermal equilibrium at temperature
in thermal equilibrium at temperature
 . Consider a substance made up of
. Consider a substance made up of  molecules. Every molecular
degree of freedom contributes
molecules. Every molecular
degree of freedom contributes
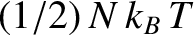 ,
or
,
or
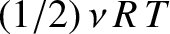 , to the mean internal energy of the substance (with the tacit proviso
that each degree of freedom is associated with a quadratic term in the energy).
Thus, the contribution to the molar heat capacity at constant volume is
, to the mean internal energy of the substance (with the tacit proviso
that each degree of freedom is associated with a quadratic term in the energy).
Thus, the contribution to the molar heat capacity at constant volume is
![$\displaystyle \frac{1}{\nu}\left(
\frac{\partial U}{\partial T}\right)_V =
\frac{1}{\nu} \frac{\partial[ (1/2)\, \nu\, R\,T] }{\partial T}= \frac{1}{2}\, R,$](img4166.png) |
(5.398) |
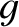 is the number of molecular degrees of freedom.
is the number of molecular degrees of freedom.
As we have seen, the equipartition theorem (and the whole classical approximation) is only valid
when the typical thermal energy, 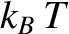 , greatly exceeds the spacing between quantum
energy levels. Suppose that the temperature is sufficiently low that this
condition is not satisfied for one particular molecular degree of freedom.
In fact, suppose that
, greatly exceeds the spacing between quantum
energy levels. Suppose that the temperature is sufficiently low that this
condition is not satisfied for one particular molecular degree of freedom.
In fact, suppose that 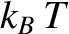 is much less than the spacing between
the energy levels.
In this situation, the degree of freedom only contributes
the ground-state energy,
is much less than the spacing between
the energy levels.
In this situation, the degree of freedom only contributes
the ground-state energy, 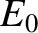 (say) to the mean energy of the molecule. Now, the
ground-state energy can be a quite complicated
function of the internal properties of the
molecule, but is certainly not a function of the temperature, because this is
a collective property of all molecules. It follows that the contribution to
the molar heat capacity is zero.
Thus, if
(say) to the mean energy of the molecule. Now, the
ground-state energy can be a quite complicated
function of the internal properties of the
molecule, but is certainly not a function of the temperature, because this is
a collective property of all molecules. It follows that the contribution to
the molar heat capacity is zero.
Thus, if 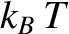 is much less than the spacing between the energy levels then
the degree of
freedom contributes nothing at all
to the molar heat capacity. We say that this particular
degree of freedom is “frozen out.” Clearly, at very low temperatures, just about
all degrees of freedom are frozen out. As the temperature is gradually increased,
degrees of freedom successively
kick in, and eventually contribute their full
is much less than the spacing between the energy levels then
the degree of
freedom contributes nothing at all
to the molar heat capacity. We say that this particular
degree of freedom is “frozen out.” Clearly, at very low temperatures, just about
all degrees of freedom are frozen out. As the temperature is gradually increased,
degrees of freedom successively
kick in, and eventually contribute their full 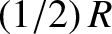 to
the molar heat capacity, as
to
the molar heat capacity, as 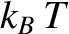 approaches, and then greatly exceeds, the spacing
between their
quantum energy levels. We can use these simple ideas to explain the behaviors
of most
experimental heat capacities.
approaches, and then greatly exceeds, the spacing
between their
quantum energy levels. We can use these simple ideas to explain the behaviors
of most
experimental heat capacities.
To make further progress, we need to
estimate the typical spacing between the quantum energy levels
associated with various degrees of freedom.
We can do this by observing the
frequency
of the electromagnetic radiation emitted and absorbed during transitions between
these energy levels. If the typical spacing between energy levels is
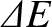 then
transitions between the various levels are associated with photons of
frequency
then
transitions between the various levels are associated with photons of
frequency 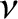 , where
, where
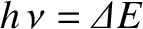 . (Here,
. (Here, 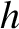 is Planck's constant.) We can define an effective
temperature of the radiation via
is Planck's constant.) We can define an effective
temperature of the radiation via
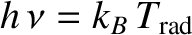 . If
. If
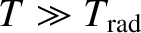 then
then
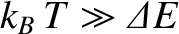 , and the degree of freedom makes its
full contribution to the heat capacity. On the other hand, if
, and the degree of freedom makes its
full contribution to the heat capacity. On the other hand, if
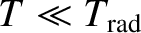 then
then
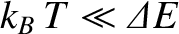 , and the degree of freedom is frozen out.
Table 5.1 lists the “temperatures” of various different types of radiation.
It is clear that degrees of freedom that give rise to emission or absorption
of radio or microwave radiation contribute their full
, and the degree of freedom is frozen out.
Table 5.1 lists the “temperatures” of various different types of radiation.
It is clear that degrees of freedom that give rise to emission or absorption
of radio or microwave radiation contribute their full 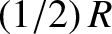 to the molar heat capacity at room temperature. On the other hand, degrees of freedom that give rise to
emission or absorption in the visible, ultraviolet, X-ray, or
to the molar heat capacity at room temperature. On the other hand, degrees of freedom that give rise to
emission or absorption in the visible, ultraviolet, X-ray, or  -ray
regions of the electromagnetic spectrum are frozen out at room temperature.
Degrees of freedom that emit or absorb infrared radiation are on the border line.
-ray
regions of the electromagnetic spectrum are frozen out at room temperature.
Degrees of freedom that emit or absorb infrared radiation are on the border line.