Next: Schrödinger's Equation Up: Wave Mechanics Previous: Wave Mechanics
 and linear momentum
and linear momentum 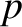 , moving in the
, moving in the  -direction (say),
can be represented by a one-dimensional wavefunction of the form
where the complex amplitude,
-direction (say),
can be represented by a one-dimensional wavefunction of the form
where the complex amplitude, 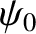 , is arbitrary, while the angular frequency,
, is arbitrary, while the angular frequency,  , and the wavenumber,
, and the wavenumber,  ,
are related to the particle energy,
,
are related to the particle energy,  , and momentum,
, and momentum, 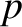 , via the fundamental
relations
(See Section 4.1.9.)
, via the fundamental
relations
(See Section 4.1.9.)
The one-dimensional wavefunction (4.10) is the solution of a one-dimensional wave equation that determines how the wavefunction evolves in time. As described in the next section, we can guess the form of this wave equation by drawing an analogy with classical physics.