
Answer: Let ![]() be the weight of the platform,
be the weight of the platform, ![]() the mass
of the platform, and
the mass
of the platform, and ![]() the tension in the rope. From Newton's third law, it is clear that
the tension in the rope. From Newton's third law, it is clear that ![]() .
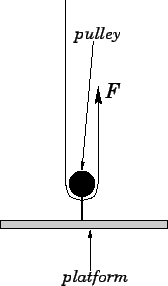
Let us apply Newton's second law to the upward motion
of the platform. The platform is subject to two vertical forces: a downward
force
.
Let us apply Newton's second law to the upward motion
of the platform. The platform is subject to two vertical forces: a downward
force ![]() due to its weight, and an upward force
due to its weight, and an upward force ![]() due to
the tension in the rope (the force is
due to
the tension in the rope (the force is ![]() , rather than
, rather than ![]() , because both
the leftmost and rightmost sections of the rope, emerging from the pulley,
are in tension and exerting an upward force on the pulley). Thus, the
upward acceleration
, because both
the leftmost and rightmost sections of the rope, emerging from the pulley,
are in tension and exerting an upward force on the pulley). Thus, the
upward acceleration ![]() of the platform is
of the platform is