 |
 |
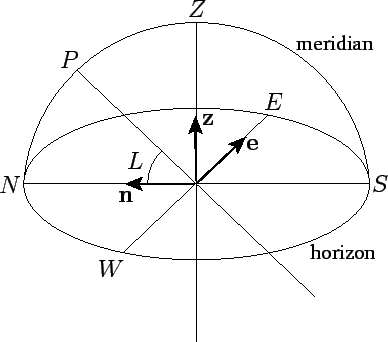 |
Figure 8 shows the visible half of the celestial sphere at observation site ![]() .
Here,
.
Here, ![]() is the local horizon, and
is the local horizon, and ![]() ,
, ![]() ,
, ![]() , and
, and ![]() are
the north, east, south, and west compass points, respectively. The plane
are
the north, east, south, and west compass points, respectively. The plane ![]() ,
which passes through the north and south compass points, as well as the zenith,
is known as the local meridian. The meridian is perpendicular
to the horizon. The north celestial pole lies in the meridian plane, and
is elevated an angular distance
,
which passes through the north and south compass points, as well as the zenith,
is known as the local meridian. The meridian is perpendicular
to the horizon. The north celestial pole lies in the meridian plane, and
is elevated an angular distance ![]() above the north compass point--see Figs. 7 and 8. Here,
above the north compass point--see Figs. 7 and 8. Here,
![]() is the terrestrial latitude of observation site
is the terrestrial latitude of observation site ![]() . It is helpful to
define three, right-handed, mutually perpendicular, local unit vectors:
. It is helpful to
define three, right-handed, mutually perpendicular, local unit vectors: ![]() ,
, ![]() , and
, and ![]() .
Here,
.
Here,
![]() is directed toward the east compass point,
is directed toward the east compass point, ![]() toward the north compass point, and
toward the north compass point, and ![]() toward the zenith--see Fig. 8.
toward the zenith--see Fig. 8.
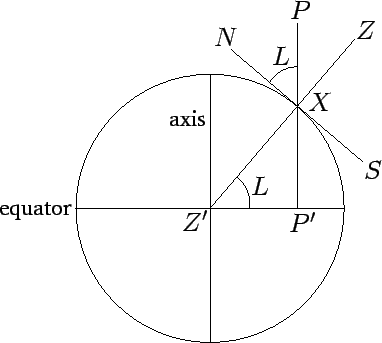
Figure 9 shows the meridian plane at ![]() . Let the line
. Let the line ![]() lie
in this plane such that it is perpendicular to the celestial axis,
lie
in this plane such that it is perpendicular to the celestial axis, ![]() . Moreover, let
. Moreover, let
![]() lie in the visible hemisphere. It is helpful to define the unit vector
lie in the visible hemisphere. It is helpful to define the unit vector ![]() which
is directed toward
which
is directed toward ![]() , as shown in the diagram. It is easily seen that
, as shown in the diagram. It is easily seen that
Figure 10 shows the celestial equator viewed from observation site ![]() . Here,
. Here, ![]() is
the right ascension of the celestial objects culminating (i.e., reaching
their highest altitude in the sky) on the meridian at the time of observation. Incidentally, it is
easily demonstrated that all objects culminating on the meridian at
any instant in time have the same right ascension. Note that the angle
is
the right ascension of the celestial objects culminating (i.e., reaching
their highest altitude in the sky) on the meridian at the time of observation. Incidentally, it is
easily demonstrated that all objects culminating on the meridian at
any instant in time have the same right ascension. Note that the angle ![]() increases uniformly in time, at the rate of
increases uniformly in time, at the rate of ![]() a (sidereal) hour, due to the diurnal motion of the celestial
sphere. It can be seen from the diagram that
a (sidereal) hour, due to the diurnal motion of the celestial
sphere. It can be seen from the diagram that