Collision Times
It is conventional to define the collision time,
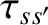 , associated with collisions of species-
, associated with collisions of species- particles with species-
particles with species- particles such that
particles such that
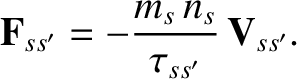 |
(3.184) |
It follows from Equation (3.180) that
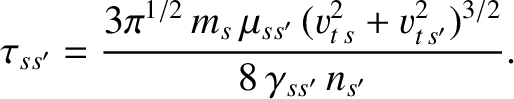 |
(3.185) |
Furthermore, when expressed in terms of the collision time,
expression (3.184) for the collisional heating rate becomes
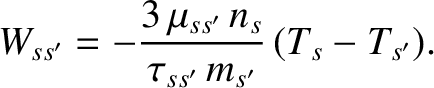 |
(3.186) |
According to the definition (3.185), the collision time,
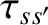 , is the time required for collisions with species-
, is the time required for collisions with species- particles to decelerate species-
particles to decelerate species- particles to such an extent that the mean drift velocity of the latter particles with respect to the former is eliminated. At the individual
particle level, the collision time is the mean time required for the direction of motion of an individual species-
particles to such an extent that the mean drift velocity of the latter particles with respect to the former is eliminated. At the individual
particle level, the collision time is the mean time required for the direction of motion of an individual species- particle to deviate through
approximately
particle to deviate through
approximately 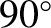 as a consequence of collisions with species-
as a consequence of collisions with species- particles.
particles.
Consider a quasi-neutral plasma consisting of electrons of mass 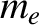 , charge
, charge 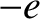 , and number density
, and number density 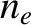 , and ions
of mass
, and ions
of mass 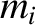 , charge
, charge 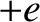 , and number density
, and number density 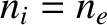 . Let the two species both have Maxwellian distributions characterized by a
common temperature
. Let the two species both have Maxwellian distributions characterized by a
common temperature 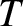 , and a small relative drift velocity. It follows, from the previous analysis, that we can
identify four different collision times. First, the electron-electron collision time,
, and a small relative drift velocity. It follows, from the previous analysis, that we can
identify four different collision times. First, the electron-electron collision time,
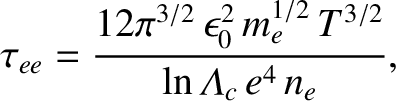 |
(3.187) |
which is the mean time required for the direction of motion of an individual electron to deviate through
approximately 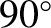 as a consequence of collisions with other electrons.
Second, the electron-ion collision time,
as a consequence of collisions with other electrons.
Second, the electron-ion collision time,
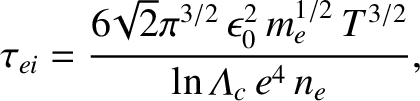 |
(3.188) |
which is the mean time required for the direction of motion of an individual electron to deviate through
approximately 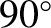 as a consequence of collisions with ions. (Here, we have made use of the fact that
as a consequence of collisions with ions. (Here, we have made use of the fact that
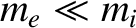 .)
Third, the ion-ion collision time,
.)
Third, the ion-ion collision time,
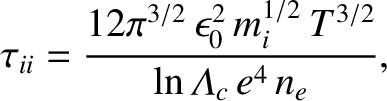 |
(3.189) |
which is the mean time required for the direction of motion of an individual ion to deviate through
approximately 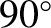 as a consequence of collisions with other ions.
Finally, the ion-electron collision time,
as a consequence of collisions with other ions.
Finally, the ion-electron collision time,
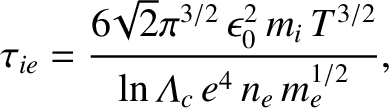 |
(3.190) |
which is the mean time required for the direction of motion of an individual ion to deviate through
approximately 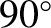 as a consequence of collisions with electrons. Note that these collision times are not all
of the same magnitude, as a consequence of the large difference between the electron and ion masses.
In fact,
as a consequence of collisions with electrons. Note that these collision times are not all
of the same magnitude, as a consequence of the large difference between the electron and ion masses.
In fact,
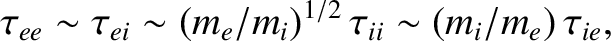 |
(3.191) |
which implies that electrons scatter electrons (through 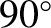 ) at about the same rate that ions scatter electrons, but that ions scatter ions
at a significantly lower rate than ions scatter electrons, and, finally, that electrons scatter ions at a significantly lower rate than ions scatter ions.
) at about the same rate that ions scatter electrons, but that ions scatter ions
at a significantly lower rate than ions scatter electrons, and, finally, that electrons scatter ions at a significantly lower rate than ions scatter ions.
The collision frequency is simply the inverse of the collision time. Thus, the electron-electron collision frequency
is written
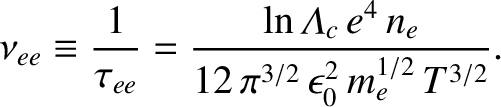 |
(3.192) |
Given that
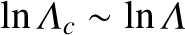 (see Section 3.10), where
(see Section 3.10), where
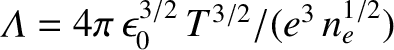 is the
plasma parameter (see Section 1.6),
we obtain the estimate (see Section 1.7)
is the
plasma parameter (see Section 1.6),
we obtain the estimate (see Section 1.7)
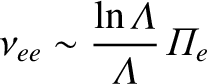 |
(3.193) |
where
 is the electron plasma frequency (see Section 1.4).
Likewise, the ion-ion collision frequency is such that
is the electron plasma frequency (see Section 1.4).
Likewise, the ion-ion collision frequency is such that
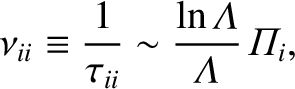 |
(3.194) |
where
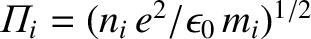 is the ion plasma frequency.
is the ion plasma frequency.
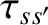 , associated with collisions of species-
, associated with collisions of species- particles with species-
particles with species- particles such that
It follows from Equation (3.180) that
Furthermore, when expressed in terms of the collision time,
expression (3.184) for the collisional heating rate becomes
particles such that
It follows from Equation (3.180) that
Furthermore, when expressed in terms of the collision time,
expression (3.184) for the collisional heating rate becomes
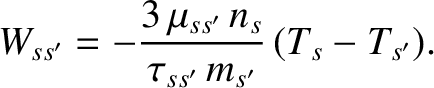
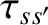 , is the time required for collisions with species-
, is the time required for collisions with species- particles to decelerate species-
particles to decelerate species- particles to such an extent that the mean drift velocity of the latter particles with respect to the former is eliminated. At the individual
particle level, the collision time is the mean time required for the direction of motion of an individual species-
particles to such an extent that the mean drift velocity of the latter particles with respect to the former is eliminated. At the individual
particle level, the collision time is the mean time required for the direction of motion of an individual species- particle to deviate through
approximately
particle to deviate through
approximately 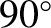 as a consequence of collisions with species-
as a consequence of collisions with species- particles.
particles.
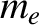 , charge
, charge 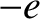 , and number density
, and number density 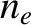 , and ions
of mass
, and ions
of mass 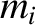 , charge
, charge 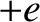 , and number density
, and number density 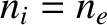 . Let the two species both have Maxwellian distributions characterized by a
common temperature
. Let the two species both have Maxwellian distributions characterized by a
common temperature 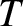 , and a small relative drift velocity. It follows, from the previous analysis, that we can
identify four different collision times. First, the electron-electron collision time,
, and a small relative drift velocity. It follows, from the previous analysis, that we can
identify four different collision times. First, the electron-electron collision time,
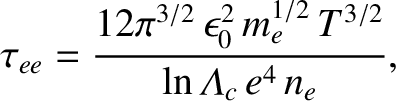
 as a consequence of collisions with other electrons.
Second, the electron-ion collision time,
as a consequence of collisions with other electrons.
Second, the electron-ion collision time,
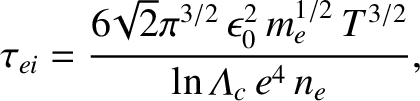
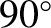 as a consequence of collisions with ions. (Here, we have made use of the fact that
as a consequence of collisions with ions. (Here, we have made use of the fact that
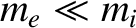 .)
Third, the ion-ion collision time,
.)
Third, the ion-ion collision time,
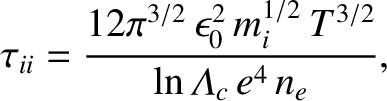
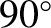 as a consequence of collisions with other ions.
Finally, the ion-electron collision time,
as a consequence of collisions with other ions.
Finally, the ion-electron collision time,
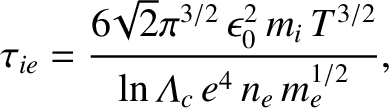
 as a consequence of collisions with electrons. Note that these collision times are not all
of the same magnitude, as a consequence of the large difference between the electron and ion masses.
In fact,
as a consequence of collisions with electrons. Note that these collision times are not all
of the same magnitude, as a consequence of the large difference between the electron and ion masses.
In fact,
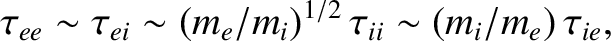
 ) at about the same rate that ions scatter electrons, but that ions scatter ions
at a significantly lower rate than ions scatter electrons, and, finally, that electrons scatter ions at a significantly lower rate than ions scatter ions.
) at about the same rate that ions scatter electrons, but that ions scatter ions
at a significantly lower rate than ions scatter electrons, and, finally, that electrons scatter ions at a significantly lower rate than ions scatter ions.
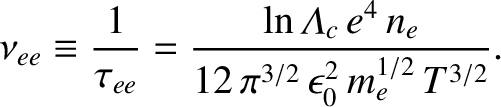
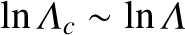 (see Section 3.10), where
(see Section 3.10), where
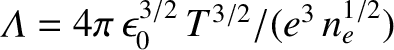 is the
plasma parameter (see Section 1.6),
we obtain the estimate (see Section 1.7)
is the
plasma parameter (see Section 1.6),
we obtain the estimate (see Section 1.7)
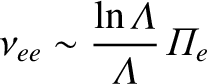
 is the electron plasma frequency (see Section 1.4).
Likewise, the ion-ion collision frequency is such that
is the electron plasma frequency (see Section 1.4).
Likewise, the ion-ion collision frequency is such that
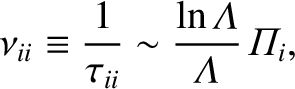
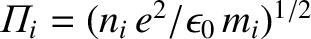 is the ion plasma frequency.
is the ion plasma frequency.