Next: Fresnel Relations Up: Multi-Dimensional Waves Previous: Polarization of Electromagnetic Waves Contents
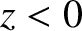 is occupied by a transparent dielectric medium of refractive index
is occupied by a transparent dielectric medium of refractive index 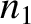 , whereas
the region
, whereas
the region 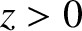 is occupied by a second transparent dielectric medium of refractive index
is occupied by a second transparent dielectric medium of refractive index 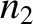 . See Figure 7.7. Let a plane light wave be launched toward positive
. See Figure 7.7. Let a plane light wave be launched toward positive  from a
light source of angular frequency
from a
light source of angular frequency 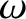 located at large negative
located at large negative  . Furthermore, suppose that this wave, which has the wavevector
. Furthermore, suppose that this wave, which has the wavevector 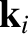 , is obliquely incident on the interface between the two media. We would expect the incident plane wave to be partially reflected and partially
refracted (i.e., transmitted) by the interface. (See Section 6.8.)
Let the reflected and refracted plane waves have the wavevectors
, is obliquely incident on the interface between the two media. We would expect the incident plane wave to be partially reflected and partially
refracted (i.e., transmitted) by the interface. (See Section 6.8.)
Let the reflected and refracted plane waves have the wavevectors
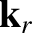 and
and 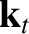 , respectively. See Figure 7.7. Hence, we can write
, respectively. See Figure 7.7. Hence, we can write
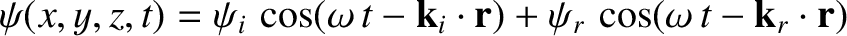 |
(7.40) |
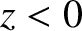 , and
, and
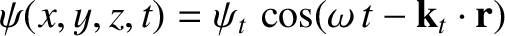 |
(7.41) |
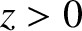 . Here,
. Here,
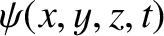 represents the magnetic component of the resultant light wave,
represents the magnetic component of the resultant light wave, 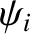 the
amplitude of the incident wave,
the
amplitude of the incident wave, 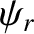 the amplitude of the reflected wave, and
the amplitude of the reflected wave, and 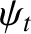 the amplitude of the
refracted wave. All of the component waves have the same angular frequency,
the amplitude of the
refracted wave. All of the component waves have the same angular frequency, 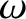 , because this property is
ultimately determined by the wave source. Furthermore, according to standard electromagnetic
theory (Fitzpatrick 2008), if the magnetic component of an electromagnetic wave is specified then the electric
component of the wave is fully determined, and vice versa.
, because this property is
ultimately determined by the wave source. Furthermore, according to standard electromagnetic
theory (Fitzpatrick 2008), if the magnetic component of an electromagnetic wave is specified then the electric
component of the wave is fully determined, and vice versa.
In general, the wavefunction, 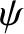 , must be continuous at
, must be continuous at 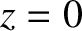 , because, according to standard electromagnetic theory (see Appendix C), there cannot be a discontinuity in either the
normal or the tangential component of a magnetic field across an interface between two (non-magnetic) dielectric media. [The same is not true of an electric field, which can have a normal discontinuity across
an interface between two dielectric media (ibid.). This explains why we have chosen
, because, according to standard electromagnetic theory (see Appendix C), there cannot be a discontinuity in either the
normal or the tangential component of a magnetic field across an interface between two (non-magnetic) dielectric media. [The same is not true of an electric field, which can have a normal discontinuity across
an interface between two dielectric media (ibid.). This explains why we have chosen 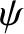 to represent the magnetic, rather than the electric,
component of the wave.] Thus, the matching condition at
to represent the magnetic, rather than the electric,
component of the wave.] Thus, the matching condition at 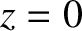 takes the form
takes the form
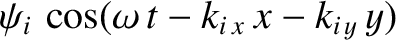 |
||
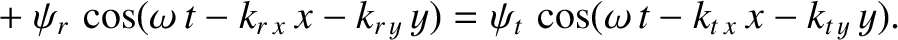 |
(7.42) |
 ,
, 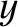 , and
, and 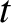 . This is only possible if
and
. This is only possible if
and
Suppose that the direction of propagation of the incident wave lies in the  -
- plane, so that
plane, so that
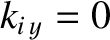 . It immediately
follows, from Equation (7.44), that
. It immediately
follows, from Equation (7.44), that
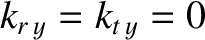 . In other words, the directions of propagation of the reflected
and the refracted waves also lie in the
. In other words, the directions of propagation of the reflected
and the refracted waves also lie in the  -
- plane, which implies that
plane, which implies that 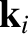 ,
, 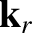 and
and 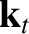 are
co-planar vectors. This constraint is implicit in the well-known laws of
geometric optics (Hecht 1974).
are
co-planar vectors. This constraint is implicit in the well-known laws of
geometric optics (Hecht 1974).
Assuming that the previously mentioned constraint is satisfied, let the incident, reflected, and refracted wave normals subtend
angles 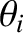 ,
, 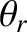 , and
, and 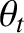 with the
with the  -axis, respectively. See Figure 7.7. It follows that
-axis, respectively. See Figure 7.7. It follows that
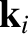 |
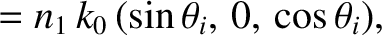 |
(7.45) |
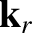 |
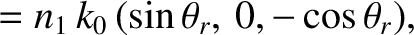 |
(7.46) |
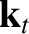 |
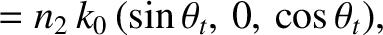 |
(7.47) |
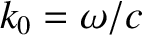 is the vacuum wavenumber, and
is the vacuum wavenumber, and 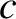 the velocity of light in vacuum.
Here, we have made use of the fact that wavenumber (i.e., the magnitude of the wavevector) of a light wave propagating through a dielectric medium of
refractive index
the velocity of light in vacuum.
Here, we have made use of the fact that wavenumber (i.e., the magnitude of the wavevector) of a light wave propagating through a dielectric medium of
refractive index 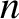 is
is 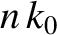 . (See Section 6.8.)
. (See Section 6.8.)
According to Equation (7.43),
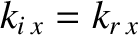 , which yields
, which yields
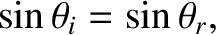 |
(7.48) |
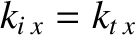 , which reduces to
The first of these relations states that the angle of incidence,
, which reduces to
The first of these relations states that the angle of incidence, 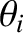 , is equal to the angle of reflection,
, is equal to the angle of reflection, 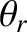 . This is the
familiar law of reflection (Hecht 1974). Furthermore, the second relation corresponds to the equally familiar
law of refraction, otherwise known as Snell's law (ibid.).
. This is the
familiar law of reflection (Hecht 1974). Furthermore, the second relation corresponds to the equally familiar
law of refraction, otherwise known as Snell's law (ibid.).
Incidentally, the fact that a plane wave propagates through a uniform dielectric medium with a constant wavevector, and, therefore, a constant direction of motion, is equivalent to the well-known law of rectilinear propagation, which states that a light ray (i.e., the normal to a constant phase surface) propagates through a uniform medium in a straight line (Hecht 1974).
It follows, from the previous discussion, that the laws of geometric optics (i.e., the law of rectilinear propagation, the law of reflection, and the law of refraction) are fully consistent with the wave properties of light, despite the fact that they do not seem to explicitly depend on these properties.