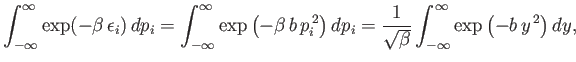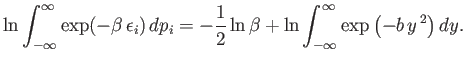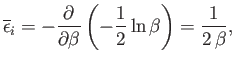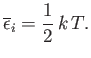Next: Harmonic Oscillators
Up: Applications of Statistical Thermodynamics
Previous: General Paramagnetism
Equipartition Theorem
The internal energy of a monatomic ideal gas containing  particles is
particles is
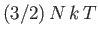 .
This means that each particle possess, on average,
.
This means that each particle possess, on average,
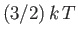 units of energy.
Monatomic particles have only three translational degrees
of freedom, corresponding to
their motion in three dimensions. They possess no internal
rotational or vibrational degrees of freedom. Thus, the mean energy per degree of
freedom in a monatomic ideal gas is
units of energy.
Monatomic particles have only three translational degrees
of freedom, corresponding to
their motion in three dimensions. They possess no internal
rotational or vibrational degrees of freedom. Thus, the mean energy per degree of
freedom in a monatomic ideal gas is
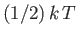 . In fact,
this is a special case of a rather general result. Let us now try to prove this.
. In fact,
this is a special case of a rather general result. Let us now try to prove this.
Suppose that the energy of a
system is determined by 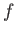 generalized coordinates,
generalized coordinates, 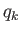 ,
and
,
and 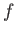 corresponding generalized momenta,
corresponding generalized momenta, 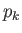 , so that
, so that
 |
(7.124) |
Suppose further that:
- The total energy splits additively into the form
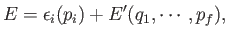 |
(7.125) |
where
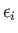 involves only one variable,
involves only one variable, 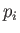 , and the remaining part,
, and the remaining part,
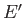 , does not depend on
, does not depend on 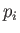 .
.
- The function
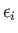 is quadratic in
is quadratic in 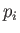 , so that
, so that
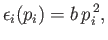 |
(7.126) |
where 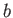 is a constant.
is a constant.
The most common situation in which the previous assumptions are valid is where
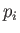 is a momentum. This is because the kinetic energy is usually a quadratic
function of each momentum component, whereas the potential energy does not
involve the momenta at all. However, if a coordinate,
is a momentum. This is because the kinetic energy is usually a quadratic
function of each momentum component, whereas the potential energy does not
involve the momenta at all. However, if a coordinate, 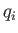 , were to satisfy
assumptions 1 and 2 then the theorem that we are about to establish would hold just
as well.
, were to satisfy
assumptions 1 and 2 then the theorem that we are about to establish would hold just
as well.
What is the mean value of
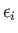 in thermal equilibrium if conditions
1 and 2 are satisfied? If the system is in equilibrium at absolute temperature
in thermal equilibrium if conditions
1 and 2 are satisfied? If the system is in equilibrium at absolute temperature
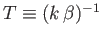 then it is distributed according to the canonical probability
distribution. In the classical approximation,
the mean value of
then it is distributed according to the canonical probability
distribution. In the classical approximation,
the mean value of
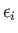 is expressed in terms of
integrals over all phase-space:
is expressed in terms of
integrals over all phase-space:
![$\displaystyle \overline{\epsilon_i} = \frac{ \int_{-\infty}^{\infty} \exp[-\bet...
... {\int_{-\infty}^{\infty} \exp[-\beta E(q_1,\cdots, p_f)] dq_1\cdots dp_f}.$](img1498.png) |
(7.127) |
Condition 1 gives
where use has been made of the multiplicative property of the exponential function,
and where the final integrals in both the numerator and denominator extend over
all variables,
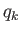 and
and 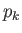 , except for
, except for 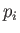 . These integrals are equal and, thus, cancel.
Hence,
. These integrals are equal and, thus, cancel.
Hence,
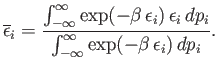 |
(7.129) |
This expression can be simplified further because
![$\displaystyle \int_{-\infty}^\infty \exp(-\beta \epsilon_i) \epsilon_i dp_...
...ial \beta} \left[\int_{-\infty}^\infty \exp(-\beta \epsilon_i) dp_i\right],$](img1503.png) |
(7.130) |
so
![$\displaystyle \overline{\epsilon}_i = - \frac{\partial}{\partial\beta} \ln\left[\int_{-\infty}^\infty \exp(-\beta \epsilon_i) dp_i\right].$](img1504.png) |
(7.131) |
According to condition 2,
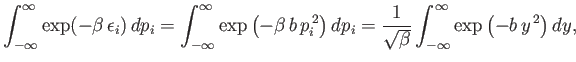 |
(7.132) |
where
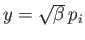 . Thus,
. Thus,
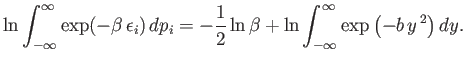 |
(7.133) |
Note that the integral on the right-hand side is independent of 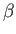 . It follows
from Equation (7.131) that
. It follows
from Equation (7.131) that
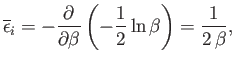 |
(7.134) |
giving
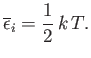 |
(7.135) |
This is the famous equipartition theorem of classical physics.
It states that the mean value of every independent
quadratic term in the energy is equal to
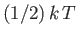 . If all terms in the energy are quadratic
then the mean energy is spread equally over all degrees of freedom. (Hence, the
name ``equipartition.'')
. If all terms in the energy are quadratic
then the mean energy is spread equally over all degrees of freedom. (Hence, the
name ``equipartition.'')



Next: Harmonic Oscillators
Up: Applications of Statistical Thermodynamics
Previous: General Paramagnetism
Richard Fitzpatrick
2016-01-25
![]() generalized coordinates,
generalized coordinates, ![]() ,
and
,
and ![]() corresponding generalized momenta,
corresponding generalized momenta, ![]() , so that
, so that
![]() in thermal equilibrium if conditions
1 and 2 are satisfied? If the system is in equilibrium at absolute temperature
in thermal equilibrium if conditions
1 and 2 are satisfied? If the system is in equilibrium at absolute temperature
![]() then it is distributed according to the canonical probability
distribution. In the classical approximation,
the mean value of
then it is distributed according to the canonical probability
distribution. In the classical approximation,
the mean value of
![]() is expressed in terms of
integrals over all phase-space:
is expressed in terms of
integrals over all phase-space:
![$\displaystyle \overline{\epsilon_i} = \frac{ \int_{-\infty}^{\infty} \exp[-\bet...
... {\int_{-\infty}^{\infty} \exp[-\beta E(q_1,\cdots, p_f)] dq_1\cdots dp_f}.$](img1498.png)
![$\displaystyle =\frac{ \int_{-\infty}^{\infty} \exp[-\beta (\epsilon_i + E')] ...
...p_f} {\int_{-\infty}^{\infty} \exp[-\beta (\epsilon_i + E')] dq_1\cdots dp_f}$](img1500.png)
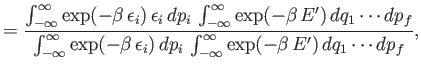
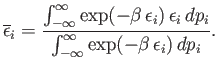
![$\displaystyle \int_{-\infty}^\infty \exp(-\beta \epsilon_i) \epsilon_i dp_...
...ial \beta} \left[\int_{-\infty}^\infty \exp(-\beta \epsilon_i) dp_i\right],$](img1503.png)