Next: Mean, Variance, and Standard
Up: Probability Theory
Previous: Combinatorial Analysis
Binomial Probability Distribution
It follows from Equations (2.16) and (2.20) that the probability of obtaining
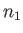 occurrences of the
outcome 1 in
occurrences of the
outcome 1 in  statistically independent observations of a two-state system
is
statistically independent observations of a two-state system
is
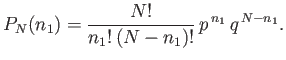 |
(2.21) |
This probability function is called the binomial probability distribution.
The reason for this name becomes obvious if we tabulate the probabilities for the
first few possible values of  , as is done in Table 2.1.
Of course, we immediately recognize the expressions appearing in the first four rows of this table:
they appear in the standard
algebraic expansions of
, as is done in Table 2.1.
Of course, we immediately recognize the expressions appearing in the first four rows of this table:
they appear in the standard
algebraic expansions of 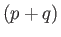 ,
,
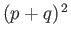 ,
,
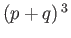 , and
, and
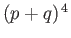 ,
respectively. In algebra, the expansion of
,
respectively. In algebra, the expansion of
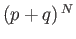 is called the
binomial expansion (hence, the name given to the probability distribution
function),
and is written
is called the
binomial expansion (hence, the name given to the probability distribution
function),
and is written
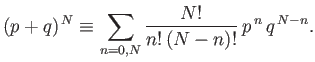 |
(2.22) |
Equations (2.21) and (2.22) can be used to establish the normalization
condition for the binomial distribution function:
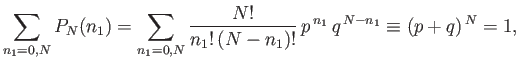 |
(2.23) |
because 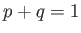 . [See Equation (2.11).]
. [See Equation (2.11).]
Table 2.1:
The binomial probability distribution,  .
.
|
|



Next: Mean, Variance, and Standard
Up: Probability Theory
Previous: Combinatorial Analysis
Richard Fitzpatrick
2016-01-25