


Next: Resonances
Up: Scattering Theory
Previous: Hard Sphere Scattering
In general, at low energies (i.e., when 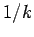 is much larger than the range
of the potential) partial waves with
is much larger than the range
of the potential) partial waves with 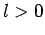 make a
negligible contribution to the scattering cross-section. It follows
that, at these energies, with a finite range potential, only
make a
negligible contribution to the scattering cross-section. It follows
that, at these energies, with a finite range potential, only 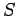 -wave
scattering is important.
-wave
scattering is important.
As a specific example, let us consider scattering by a finite
potential well, characterized by 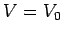 for
for 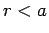 , and
, and
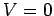 for
for 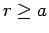 . Here,
. Here, 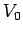 is a constant. The potential
is repulsive for
is a constant. The potential
is repulsive for 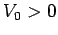 , and attractive for
, and attractive for 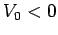 .
The outside wavefunction is given by [see Eq. (1310)]
.
The outside wavefunction is given by [see Eq. (1310)]
where use has been made of Eqs. (1289) and (1290).
The inside wavefunction follows from Eq. (1315). We obtain
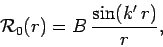 |
(1333) |
where use has been made of the boundary condition (1316).
Here, 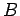 is a constant, and
is a constant, and
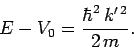 |
(1334) |
Note that Eq. (1333) only applies when 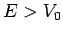 . For
. For 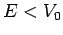 , we have
, we have
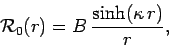 |
(1335) |
where
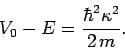 |
(1336) |
Matching 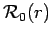 , and its radial derivative, at
, and its radial derivative, at 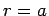 yields
yields
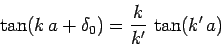 |
(1337) |
for 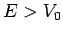 , and
, and
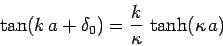 |
(1338) |
for 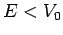 .
.
Consider an attractive potential, for which 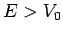 . Suppose that
. Suppose that
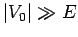 (i.e., the depth of the potential well is much larger than
the energy of the incident particles), so that
(i.e., the depth of the potential well is much larger than
the energy of the incident particles), so that 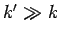 . We can see
from Eq. (1337) that, unless
. We can see
from Eq. (1337) that, unless 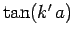 becomes extremely large, the right-hand side is much less that unity, so replacing the tangent of a
small quantity with the quantity itself, we obtain
becomes extremely large, the right-hand side is much less that unity, so replacing the tangent of a
small quantity with the quantity itself, we obtain
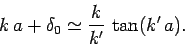 |
(1339) |
This yields
![\begin{displaymath}
\delta_0 \simeq k a \left[ \frac{\tan( k' a)}{k' a} -1\right].
\end{displaymath}](img3006.png) |
(1340) |
According to Eq. (1330), the scattering cross-section is given by
![\begin{displaymath}
\sigma_{\rm total} \simeq \frac{4\pi}{k^2} \sin^2\delta_0
=4\pi a^2\left[\frac{\tan (k 'a)}{k' a} -1\right]^2.
\end{displaymath}](img3007.png) |
(1341) |
Now
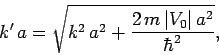 |
(1342) |
so for sufficiently small values of 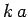 ,
,
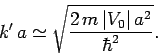 |
(1343) |
It follows that the total (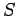 -wave) scattering cross-section is independent
of the energy of the incident particles (provided that this energy is
sufficiently small).
-wave) scattering cross-section is independent
of the energy of the incident particles (provided that this energy is
sufficiently small).
Note that there are values of 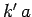 (e.g.,
(e.g.,
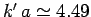 ) at which
) at which
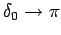 , and
the scattering cross-section (1341) vanishes, despite the very strong
attraction of the potential. In reality, the cross-section is not
exactly zero, because of contributions from
, and
the scattering cross-section (1341) vanishes, despite the very strong
attraction of the potential. In reality, the cross-section is not
exactly zero, because of contributions from 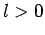 partial waves. But,
at low incident energies, these contributions are small. It follows that
there are certain values of
partial waves. But,
at low incident energies, these contributions are small. It follows that
there are certain values of 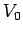 and
and 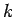 which give rise to almost perfect
transmission of the incident wave. This is called the Ramsauer-Townsend
effect, and has been observed experimentally.
which give rise to almost perfect
transmission of the incident wave. This is called the Ramsauer-Townsend
effect, and has been observed experimentally.



Next: Resonances
Up: Scattering Theory
Previous: Hard Sphere Scattering
Richard Fitzpatrick
2010-07-20
![]() for
for ![]() , and
, and
![]() for
for ![]() . Here,
. Here, ![]() is a constant. The potential
is repulsive for
is a constant. The potential
is repulsive for ![]() , and attractive for
, and attractive for ![]() .
The outside wavefunction is given by [see Eq. (1310)]
.
The outside wavefunction is given by [see Eq. (1310)]
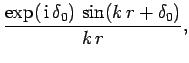
![]() . Suppose that
. Suppose that
![]() (i.e., the depth of the potential well is much larger than
the energy of the incident particles), so that
(i.e., the depth of the potential well is much larger than
the energy of the incident particles), so that ![]() . We can see
from Eq. (1337) that, unless
. We can see
from Eq. (1337) that, unless ![]() becomes extremely large, the right-hand side is much less that unity, so replacing the tangent of a
small quantity with the quantity itself, we obtain
becomes extremely large, the right-hand side is much less that unity, so replacing the tangent of a
small quantity with the quantity itself, we obtain
![\begin{displaymath}
\delta_0 \simeq k a \left[ \frac{\tan( k' a)}{k' a} -1\right].
\end{displaymath}](img3006.png)
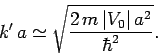
![]() (e.g.,
(e.g.,
![]() ) at which
) at which
![]() , and
the scattering cross-section (1341) vanishes, despite the very strong
attraction of the potential. In reality, the cross-section is not
exactly zero, because of contributions from
, and
the scattering cross-section (1341) vanishes, despite the very strong
attraction of the potential. In reality, the cross-section is not
exactly zero, because of contributions from ![]() partial waves. But,
at low incident energies, these contributions are small. It follows that
there are certain values of
partial waves. But,
at low incident energies, these contributions are small. It follows that
there are certain values of ![]() and
and ![]() which give rise to almost perfect
transmission of the incident wave. This is called the Ramsauer-Townsend
effect, and has been observed experimentally.
which give rise to almost perfect
transmission of the incident wave. This is called the Ramsauer-Townsend
effect, and has been observed experimentally.