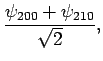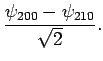Next: Fine Structure of Hydrogen
Up: Time-Independent Perturbation Theory
Previous: Degenerate Perturbation Theory
Returning to the Stark effect, let us examine the effect of an external electric
field on the energy levels of the 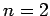 states of a hydrogen atom.
There are four such states: an
states of a hydrogen atom.
There are four such states: an 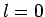 state, usually referred to as
state, usually referred to as 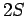 ,
and three
,
and three 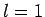 states (with
states (with 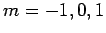 ), usually referred to as 2P. All
of these states possess the same unperturbed energy,
), usually referred to as 2P. All
of these states possess the same unperturbed energy,
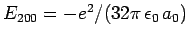 .
As before, the perturbing Hamiltonian is
.
As before, the perturbing Hamiltonian is
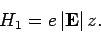 |
(956) |
According to the previously determined selection rules (i.e., 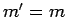 ,
and
,
and 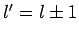 ), this Hamiltonian couples
), this Hamiltonian couples 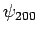 and
and 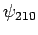 .
Hence, non-degenerate perturbation theory breaks down when applied to
these two states. On the other hand, non-degenerate perturbation
theory works fine for the
.
Hence, non-degenerate perturbation theory breaks down when applied to
these two states. On the other hand, non-degenerate perturbation
theory works fine for the 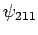 and
and 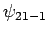 states,
since these are not coupled to any other
states,
since these are not coupled to any other 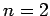 states by the perturbing
Hamiltonian.
states by the perturbing
Hamiltonian.
In order to apply perturbation theory to the 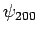 and
and 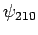 states, we have to solve the
matrix eigenvalue equation
states, we have to solve the
matrix eigenvalue equation
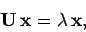 |
(957) |
where 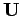 is the matrix of the matrix elements of
is the matrix of the matrix elements of 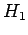 between these states. Thus,
between these states. Thus,
![\begin{displaymath}
{\bf U} = e \vert{\bf E}\vert\left(\begin{array}{cc}
0& \la...
...]
\langle 2,1,0\vert z\vert 2,0,0\rangle&0
\end{array}\right),
\end{displaymath}](img2272.png) |
(958) |
where the rows and columns correspond to 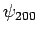 and
and 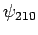 , respectively. Here, we have again
made use of the selection rules, which tell us
that the matrix element of
, respectively. Here, we have again
made use of the selection rules, which tell us
that the matrix element of 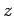 between two hydrogen atom
states is zero unless the states possess
between two hydrogen atom
states is zero unless the states possess 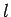 quantum numbers which differ by unity. It is easily demonstrated,
from the exact forms of the 2S and 2P wavefunctions, that
quantum numbers which differ by unity. It is easily demonstrated,
from the exact forms of the 2S and 2P wavefunctions, that
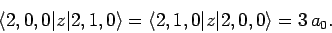 |
(959) |
It can be seen, by inspection, that the eigenvalues of 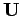 are
are
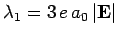 and
and
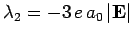 . The corresponding normalized eigenvectors are
. The corresponding normalized eigenvectors are
It follows that the simultaneous eigenstates of 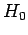 and
and 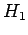 take the form
take the form
In the absence of an external electric field, both of these states possess the
same energy, 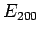 . The first-order energy shifts induced by
an external electric field are given by
. The first-order energy shifts induced by
an external electric field are given by
Thus, in the presence of
an electric field, the energies of states 1 and 2 are shifted upwards and downwards, respectively, by an amount
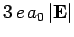 . These states are orthogonal linear combinations of the
original
. These states are orthogonal linear combinations of the
original 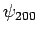 and
and 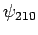 states. Note that the energy shifts
are linear in the electric field-strength, so this effect--which is
known as the linear Stark effect--is much larger than the quadratic effect described in Sect. 12.5.
Note, also, that the energies of the
states. Note that the energy shifts
are linear in the electric field-strength, so this effect--which is
known as the linear Stark effect--is much larger than the quadratic effect described in Sect. 12.5.
Note, also, that the energies of the 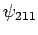 and
and 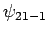 states are not affected by the electric field to first-order. Of course,
to second-order the energies of these states are shifted by an amount
which depends on the square of the electric field-strength (see Sect. 12.5).
states are not affected by the electric field to first-order. Of course,
to second-order the energies of these states are shifted by an amount
which depends on the square of the electric field-strength (see Sect. 12.5).



Next: Fine Structure of Hydrogen
Up: Time-Independent Perturbation Theory
Previous: Degenerate Perturbation Theory
Richard Fitzpatrick
2010-07-20
![]() and
and ![]() states, we have to solve the
matrix eigenvalue equation
states, we have to solve the
matrix eigenvalue equation
![\begin{displaymath}
{\bf U} = e \vert{\bf E}\vert\left(\begin{array}{cc}
0& \la...
...]
\langle 2,1,0\vert z\vert 2,0,0\rangle&0
\end{array}\right),
\end{displaymath}](img2272.png)
![]() are
are
![]() and
and
![]() . The corresponding normalized eigenvectors are
. The corresponding normalized eigenvectors are
![$\displaystyle \left(\begin{array}{c}
1/\sqrt{2}\ [0.5ex]
1/\sqrt{2}\end{array}\right),$](img2277.png)
![$\displaystyle \left(\begin{array}{c}
1/\sqrt{2}\ [0.5ex]
-1/\sqrt{2}\end{array}\right).$](img2279.png)