


Next: Relativistic Electron Theory
Up: Identical Particles
Previous: Helium Atom
- Demonstrate that the particle interchange operator,
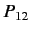 , in a system of two identical particles is Hermitian.
, in a system of two identical particles is Hermitian.
- Consider two identical spin-
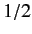 particles of mass
particles of mass  confined in a cubic box of dimension
confined in a cubic box of dimension  . Find the possible
energies and wavefunctions of this system in the case of no interaction between the particles.
. Find the possible
energies and wavefunctions of this system in the case of no interaction between the particles.
- Consider a system of two spin-
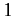 particles with no orbital angular momentum (i.e., both particles are in
particles with no orbital angular momentum (i.e., both particles are in 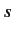 -states). What are
the possible eigenvalues of the total spin angular momentum of the system, as well as its projection along the
-states). What are
the possible eigenvalues of the total spin angular momentum of the system, as well as its projection along the 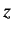 -direction, in the
cases in which the particles are non-identical and identical?
-direction, in the
cases in which the particles are non-identical and identical?
Richard Fitzpatrick
2013-04-08