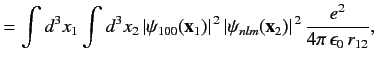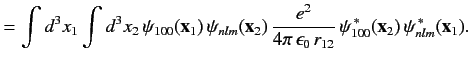Next: Exercises
Up: Identical Particles
Previous: Two-Electron System
Consider the helium atom, which is a good example of a two-electron system. The Hamiltonian is written
 |
(1081) |
where 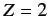 ,
,
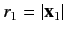 ,
,
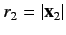 , and
, and
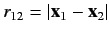 . Suppose that the final term on the right-hand side of the above expression were absent. In this case, the overall spatial wavefunction can be formed from products of hydrogen atom wavefunctions calculated with
. Suppose that the final term on the right-hand side of the above expression were absent. In this case, the overall spatial wavefunction can be formed from products of hydrogen atom wavefunctions calculated with
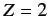 , instead of
, instead of 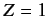 . Each of these wavefunctions is characterized by the usual triplet of quantum numbers,
. Each of these wavefunctions is characterized by the usual triplet of quantum numbers,  ,
,  , and
, and  .
Now, the total spin of the system is a constant of the motion (since
.
Now, the total spin of the system is a constant of the motion (since 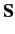 obviously commutes with the Hamiltonian),
so the overall spin state is either the singlet or the triplet state. The corresponding spatial wavefunction is symmetric in the former case, and
antisymmetric in the latter. Suppose that one electron has the quantum numbers
obviously commutes with the Hamiltonian),
so the overall spin state is either the singlet or the triplet state. The corresponding spatial wavefunction is symmetric in the former case, and
antisymmetric in the latter. Suppose that one electron has the quantum numbers  ,
,  ,
,  whereas the other has the quantum numbers
whereas the other has the quantum numbers 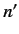 ,
, 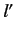 ,
, 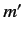 .
The corresponding spatial wavefunction is
.
The corresponding spatial wavefunction is
![$\displaystyle \phi({\bf x}_1,{\bf x}_2)=\frac{1}{\sqrt{2}}\left[\psi_{nlm}({\bf...
..._{n'l'm'}({\bf x}_2)\pm \psi_{nlm}({\bf x}_2)\,\psi_{n'l'm'}({\bf x}_1)\right],$](img2497.png) |
(1082) |
where the plus and minus signs correspond to the singlet and triplet spin states, respectively. Here,
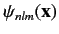 is a standard hydrogen atom wavefunction (calculated with
is a standard hydrogen atom wavefunction (calculated with 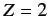 ). For the special case in which the two sets of spatial quantum numbers,
). For the special case in which the two sets of spatial quantum numbers,  ,
,  ,
,  and
and 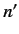 ,
,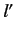 ,
,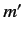 , are the same, the triplet spin state does not exist (because the associated spatial wavefunction is null). Hence, only
singlet spin state is allowed, and the spatial wavefunction reduces to
, are the same, the triplet spin state does not exist (because the associated spatial wavefunction is null). Hence, only
singlet spin state is allowed, and the spatial wavefunction reduces to
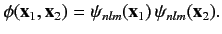 |
(1083) |
In particular, the ground state ( ,
, 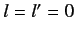 ,
, 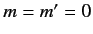 ) can only exist as a singlet spin state (i.e., a state of overall spin 0), and has the
spatial wavefunction
) can only exist as a singlet spin state (i.e., a state of overall spin 0), and has the
spatial wavefunction
![$\displaystyle \phi({\bf x}_1,{\bf x}_2) =\psi_{100}({\bf x}_1)\,\psi_{100}({\bf...
...2)= \frac{Z^{\,3}}{\pi\,a_0^{\,3}}\,\exp\left[\frac{-Z\,(r_1+r_2)}{a_0}\right],$](img2502.png) |
(1084) |
where 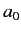 is the Bohr radius. This follows because
is the Bohr radius. This follows because
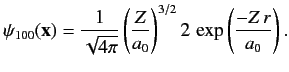 |
(1085) |
The energy of this state
is
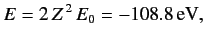 |
(1086) |
where
 is the ground state energy of a hydrogen atom. In the above expression, the factor of
is the ground state energy of a hydrogen atom. In the above expression, the factor of 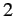 comes from the fact that
there are two electrons in a helium atom.
comes from the fact that
there are two electrons in a helium atom.
The above estimate for the ground state energy of a helium atom completely ignores the final term on the right-hand side of Equation (1081),
which describes the mutual interaction between the two electrons. We can obtain a better estimate for the ground state energy by treating (1084)
as the unperturbed wavefunction, and
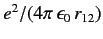 as a perturbation. According to standard first-order perturbation theory, the
correction to the ground state energy is
as a perturbation. According to standard first-order perturbation theory, the
correction to the ground state energy is
![$\displaystyle {\mit\Delta} E = \left\langle \frac{e^2}{4\pi\,\epsilon_0\,r_{12}...
...p\left[\frac{-2\,Z\,(r_1+r_2)}{a_0}\right]\frac{e^2}{4\pi\,\epsilon_0\,r_{12}}.$](img2507.png) |
(1087) |
This can be written
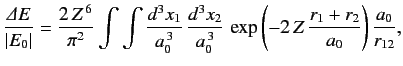 |
(1088) |
since
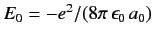 . Now,
. Now,
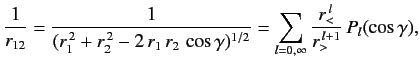 |
(1089) |
where 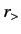 (
(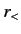 ) is the larger (smaller) of
) is the larger (smaller) of 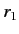 and
and 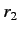 , and
, and 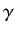 is the angle subtended between
is the angle subtended between 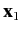 and
and 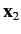 .
Moreover, the so-called addition theorem for spherical harmonics states that
.
Moreover, the so-called addition theorem for spherical harmonics states that
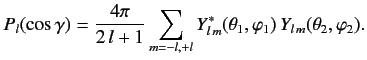 |
(1090) |
However,
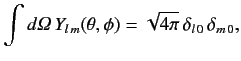 |
(1091) |
so we obtain
Here,
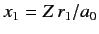 and
and
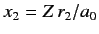 , and
, and 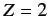 .
Thus, our improved estimate for the ground state energy of the helium atom is
.
Thus, our improved estimate for the ground state energy of the helium atom is
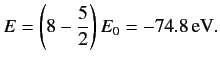 |
(1093) |
This is much closer to the experimental value of
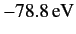 than our previous estimate.
than our previous estimate.
Consider an excited state of the helium atom in which one electron is in the ground state, while the other is in a state characterized by the quantum
numbers  ,
,  ,
,  . We can write the energy of this state as
. We can write the energy of this state as
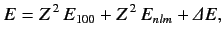 |
(1094) |
where 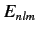 is the energy of a hydrogen atom electron whose quantum numbers are
is the energy of a hydrogen atom electron whose quantum numbers are  ,
,  ,
,  .
According to first-order perturbation theory,
.
According to first-order perturbation theory,
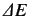 is the expectation value of
is the expectation value of
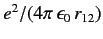 . It follows from
(1082) (with
. It follows from
(1082) (with
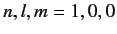 and
and
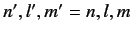 ) that
) that
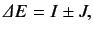 |
(1095) |
where
Here, the plus sign in (1095) corresponds to the spin singlet state, whereas the minus sign corresponds to the spin triplet state. The
integral 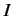 --which is known as the direct integral--is obviously positive. The integral
--which is known as the direct integral--is obviously positive. The integral 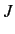 --which is known as the exchange integral--can
be shown to also be positive. Hence, we conclude that in excited states of helium the spin singlet state has a higher energy
than the spin triplet state. Incidentally, helium in the spin singlet state is known as para-helium, whereas helium in the triplet state is
called ortho-helium. As we have seen, for the ground state, only para-helium is possible.
--which is known as the exchange integral--can
be shown to also be positive. Hence, we conclude that in excited states of helium the spin singlet state has a higher energy
than the spin triplet state. Incidentally, helium in the spin singlet state is known as para-helium, whereas helium in the triplet state is
called ortho-helium. As we have seen, for the ground state, only para-helium is possible.
The fact that para-helium energy levels lie slightly above corresponding ortho-helium levels is interesting because our original Hamiltonian does not
depend on spin. Nevertheless, there is a spin dependent effect--i.e., a helium atom has a lower energy when its electrons possess parallel spins--as a consequence of
Fermi-Dirac statistics. To be more exact, the energy is lower in the spin triplet state because the corresponding spatial wavefunction is antisymmetric,
causing the electrons to tend to avoid one another (thereby reducing their electrostatic repulsion).



Next: Exercises
Up: Identical Particles
Previous: Two-Electron System
Richard Fitzpatrick
2013-04-08

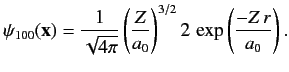
![]() as a perturbation. According to standard first-order perturbation theory, the
correction to the ground state energy is
as a perturbation. According to standard first-order perturbation theory, the
correction to the ground state energy is
![$\displaystyle {\mit\Delta} E = \left\langle \frac{e^2}{4\pi\,\epsilon_0\,r_{12}...
...p\left[\frac{-2\,Z\,(r_1+r_2)}{a_0}\right]\frac{e^2}{4\pi\,\epsilon_0\,r_{12}}.$](img2507.png)
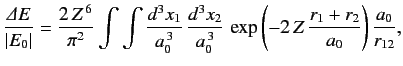
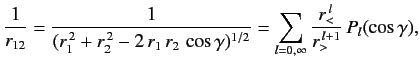
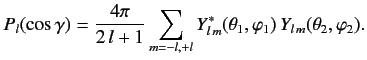
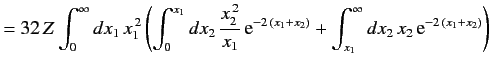
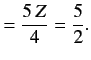
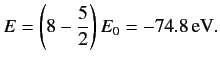
![]() ,
, ![]() ,
, ![]() . We can write the energy of this state as
. We can write the energy of this state as