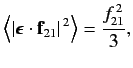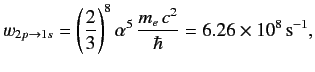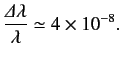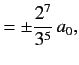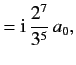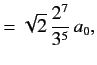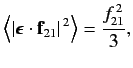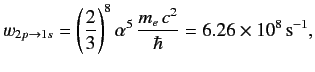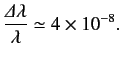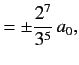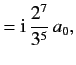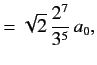Next: Scattering Theory
Up: Time-Dependent Perturbation Theory
Previous: Spontaneous Emission
- Demonstrate that
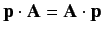 when
when
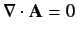 , where
, where
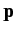 is the momentum operator, and
is the momentum operator, and
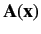 is a real function of the position operator,
is a real function of the position operator, 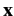 .
Hence, show that the Hamiltonian (870) is Hermitian.
.
Hence, show that the Hamiltonian (870) is Hermitian.
- Find the selection rules for the matrix elements
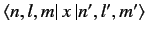 ,
,
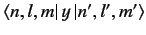 ,
and
,
and
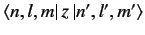 to be non-zero. Here,
to be non-zero. Here,
 denotes an energy eigenket of a hydrogen-like
atom corresponding to the conventional quantum numbers,
denotes an energy eigenket of a hydrogen-like
atom corresponding to the conventional quantum numbers,  ,
,  , and
, and  .
.
- Demonstrate that
where the average is taken over all directions of the incident radiation.
- Demonstrate that the spontaneous decay rate (via an electric dipole transition) from any 2p state to a 1s state
of a hydrogen atom is
where 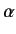 is the fine structure constant.
Hence, deduce that the natural line width of the associated spectral line is
is the fine structure constant.
Hence, deduce that the natural line width of the associated spectral line is
The only non-zero
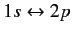 electric dipole matrix elements take the values
electric dipole matrix elements take the values
where 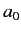 is the Bohr radius.
is the Bohr radius.



Next: Scattering Theory
Up: Time-Dependent Perturbation Theory
Previous: Spontaneous Emission
Richard Fitzpatrick
2013-04-08