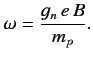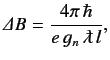Next: Pauli Two-Component Formalism
Up: Spin Angular Momentum
Previous: Magnetic Moments
The Hamiltonian for an electron at rest in a 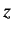 -directed magnetic field,
-directed magnetic field,
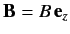 ,
is
,
is
where
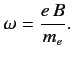 |
(464) |
According to Equation (247), the time evolution operator for this system is
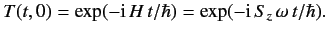 |
(465) |
It can be seen, by comparison with Equation (440), that the time evolution operator
is precisely the same as the rotation operator for spin, with
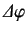 set
equal to
set
equal to
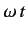 . It is immediately clear that the Hamiltonian (463)
causes the electron
spin to precess about the
. It is immediately clear that the Hamiltonian (463)
causes the electron
spin to precess about the 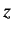 -axis with angular frequency
-axis with angular frequency 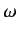 . In fact,
Equations (451)-(453) imply that
. In fact,
Equations (451)-(453) imply that
The time evolution of the state ket is given by analogy with Equation (456):
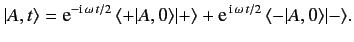 |
(469) |
Note that it takes time
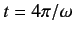 for the state ket to return to its
original state.
By contrast, it only takes times
for the state ket to return to its
original state.
By contrast, it only takes times
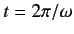 for the spin vector to point
in its original direction.
for the spin vector to point
in its original direction.
We now describe an experiment to detect the minus sign in Equation (457). An almost
monoenergetic beam of neutrons is split in two, sent along two different
paths, 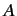 and
and 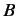 , and then recombined. Path
, and then recombined. Path 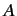 goes through a magnetic field
free region. However, path
goes through a magnetic field
free region. However, path 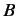 enters a small region where a static magnetic
field is present. As a result, a neutron state ket going along path
enters a small region where a static magnetic
field is present. As a result, a neutron state ket going along path
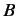 acquires a phase-shift
acquires a phase-shift
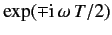 (the
(the 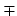 signs correspond to
signs correspond to
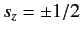 states). Here,
states). Here, 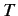 is the
time spent in the magnetic field, and
is the
time spent in the magnetic field, and 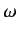 is the spin precession frequency
is the spin precession frequency
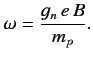 |
(470) |
This frequency is defined in an analogous manner to Equation (464). The gyromagnetic
ratio for a neutron is found experimentally to be
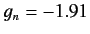 .
(The magnetic moment of a neutron is entirely a quantum field effect).
When neutrons from path
.
(The magnetic moment of a neutron is entirely a quantum field effect).
When neutrons from path 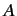 and path
and path 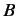 meet they undergo interference. We
expect the observed neutron intensity in the interference region to
exhibit a
meet they undergo interference. We
expect the observed neutron intensity in the interference region to
exhibit a
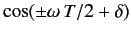 variation,
where
variation,
where 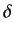 is the phase difference
between paths
is the phase difference
between paths 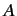 and
and 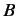 in the absence of a magnetic field. In experiments,
the time of flight
in the absence of a magnetic field. In experiments,
the time of flight 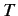 through the magnetic field region is kept constant, while
the field-strength
through the magnetic field region is kept constant, while
the field-strength 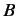 is varied. It follows that the change in magnetic
field required to produce successive maxima is
is varied. It follows that the change in magnetic
field required to produce successive maxima is
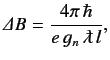 |
(471) |
where  is the path-length through the magnetic field region, and
is the path-length through the magnetic field region, and
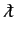 is the de Broglie wavelength over
is the de Broglie wavelength over 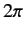 of the neutrons. The above prediction has been verified
experimentally to within a fraction of a percent. This prediction depends crucially
on the fact that it takes a
of the neutrons. The above prediction has been verified
experimentally to within a fraction of a percent. This prediction depends crucially
on the fact that it takes a 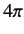 rotation to return a state ket to its
original state. If it only took a
rotation to return a state ket to its
original state. If it only took a 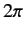 rotation then
rotation then
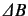 would be half
of the value given above, which does not agree with the experimental data.
would be half
of the value given above, which does not agree with the experimental data.



Next: Pauli Two-Component Formalism
Up: Spin Angular Momentum
Previous: Magnetic Moments
Richard Fitzpatrick
2013-04-08
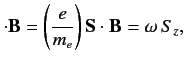
![]() and
and ![]() , and then recombined. Path
, and then recombined. Path ![]() goes through a magnetic field
free region. However, path
goes through a magnetic field
free region. However, path ![]() enters a small region where a static magnetic
field is present. As a result, a neutron state ket going along path
enters a small region where a static magnetic
field is present. As a result, a neutron state ket going along path
![]() acquires a phase-shift
acquires a phase-shift
![]() (the
(the ![]() signs correspond to
signs correspond to
![]() states). Here,
states). Here, ![]() is the
time spent in the magnetic field, and
is the
time spent in the magnetic field, and ![]() is the spin precession frequency
is the spin precession frequency