Next: Plane Waves in Homogeneous Up: Waves in Cold Plasmas Previous: Waves in Cold Plasmas Contents
What role, if any, does the geometry of the plasma equilibrium play in
determining the properties of plasma waves? Clearly, geometry plays a
key role for modes whose wavelengths are comparable to the dimensions of
the plasma. However, it is plausible that waves whose wavelengths are
much
smaller than the plasma dimensions
have properties that are, in a local sense, independent of the
geometry. In other words, the local properties of small wavelength plasma oscillations are
universal in nature. To investigate these properties, we
can, to a first approximation, represent the plasma as a homogeneous
equilibrium (corresponding to the limit
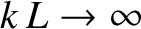 , where
, where  is the magnitude of the wavevector, and
is the magnitude of the wavevector, and 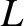 is the characteristic
equilibrium lengthscale).
is the characteristic
equilibrium lengthscale).