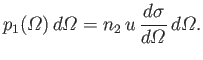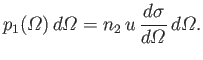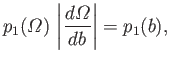Next: Landau Collision Operator
Up: Collisions
Previous: Two-Body Coulomb Collisions
Rutherford Scattering Cross-Section
Consider a particle of type  , incident with relative velocity
, incident with relative velocity 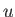 onto an ensemble of particles of type
onto an ensemble of particles of type 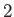 with number density
with number density 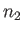 .
If
.
If
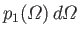 is the probability per unit time of the particle being scattered into the range of solid angle
is the probability per unit time of the particle being scattered into the range of solid angle
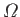 to
to
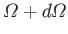 , then the differential scattering cross-section,
, then the differential scattering cross-section,
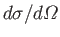 , is defined via (Reif 1965)
, is defined via (Reif 1965)
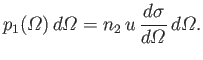 |
(3.83) |
Assuming that the scattering is azimuthally symmetric (i.e., symmetric in 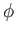 ), we can
write
), we can
write
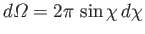 . Now, the probability per unit time of a collision having an impact parameter in the range
. Now, the probability per unit time of a collision having an impact parameter in the range
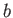 to
to 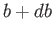 is
is
 |
(3.84) |
Furthermore, we can write
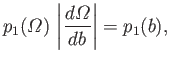 |
(3.85) |
provided that 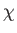 and
and 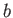 are related according to the two-particle scattering law, Equation (3.82).
It follows that
are related according to the two-particle scattering law, Equation (3.82).
It follows that
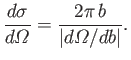 |
(3.86) |
Equation (3.82) yields
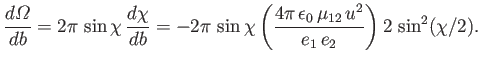 |
(3.87) |
Finally, Equations (3.82), (3.86), and (3.87) can be combined to give the so-called
Rutherford scattering cross-section,
 |
(3.88) |
It is immediately apparent, from the previous formula, that two-particle Coulomb collisions are dominated by
small-angle (i.e., small 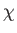 ) scattering events.
) scattering events.



Next: Landau Collision Operator
Up: Collisions
Previous: Two-Body Coulomb Collisions
Richard Fitzpatrick
2016-01-23