


Next: Van Allen Radiation Belts
Up: Charged Particle Motion
Previous: Adiabatic Invariants
Magnetic Mirrors
Consider the important case in which
the electromagnetic fields do not vary in time. It follows that
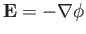 , where
, where 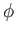 is the electrostatic potential.
Equation (2.64) yields
is the electrostatic potential.
Equation (2.64) yields
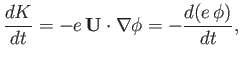 |
(2.83) |
because
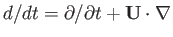 . Thus, we obtain
. Thus, we obtain
 |
(2.84) |
where
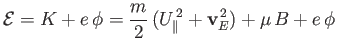 |
(2.85) |
is the lowest order total particle energy.
Not surprisingly, a charged particle neither gains nor loses energy as
it moves around in non-time-varying electromagnetic fields. Because
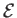 and
and 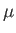 are constants of the motion, we can rearrange
Equation (2.85) to give
are constants of the motion, we can rearrange
Equation (2.85) to give
![$\displaystyle U_\parallel = \pm \left[(2/m)\,({\cal E} -\mu\,B-e\,\phi)-{\bf v}_E^{\,2}\right]^{1/2}.$](img395.png) |
(2.86) |
Thus,
charged particles can drift in either direction along magnetic field-lines in regions where
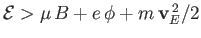 .
However, particles are excluded from regions where
.
However, particles are excluded from regions where
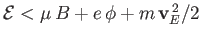 (because they cannot have
imaginary parallel velocities). Evidently, charged particles must reverse direction
at those points on magnetic field-lines where
(because they cannot have
imaginary parallel velocities). Evidently, charged particles must reverse direction
at those points on magnetic field-lines where
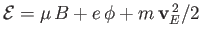 .
Such points are termed bounce points or mirror points.
.
Such points are termed bounce points or mirror points.
Let us now consider how we might construct a device to confine a
collisionless (in other words, very high temperature) plasma. Obviously, we cannot use conventional
solid walls, because they would melt. However, it is possible to confine a
hot plasma using a magnetic field (fortunately, magnetic field-lines cannot melt). This
technique is
called magnetic confinement.
The electric field in confined plasmas is
usually weak (that is, 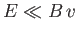 ), so that the
), so that the
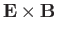 drift
is similar in magnitude to the magnetic and curvature drifts. In this
case, the bounce point condition,
drift
is similar in magnitude to the magnetic and curvature drifts. In this
case, the bounce point condition,
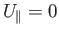 , reduces to
, reduces to
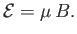 |
(2.87) |
Consider the magnetic field configuration illustrated in Figure 2.1. As indicated, this configuration is most easily
produced by two Helmholtz coils. Incidentally, this type of magnetic
confinement device is called a magnetic mirror machine.
The magnetic field configuration obviously possesses
axial symmetry. Let  be a coordinate that measures distance along the
axis of symmetry. Suppose that
be a coordinate that measures distance along the
axis of symmetry. Suppose that  corresponds to the midplane of the device
(that is, halfway between the two field-coils).
corresponds to the midplane of the device
(that is, halfway between the two field-coils).
Figure 2.1:
Schematic cross-section of a magnetic mirror machine employing two Helmholtz coils.
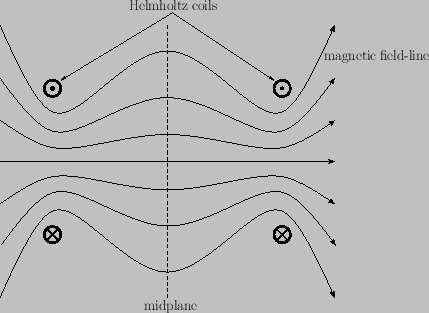 |
It is clear, from the figure, that the magnetic field-strength 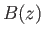 on a magnetic field-line situated close to the axis of the device attains a
local minimum
on a magnetic field-line situated close to the axis of the device attains a
local minimum
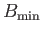 at
at  , increases symmetrically
as
, increases symmetrically
as 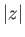 increases until
reaching a maximum value
increases until
reaching a maximum value
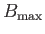 at about the locations of the two
field-coils, and then decreases as
at about the locations of the two
field-coils, and then decreases as 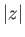 is further increased. According to
Equation (2.87), any particle that satisfies the inequality
is further increased. According to
Equation (2.87), any particle that satisfies the inequality
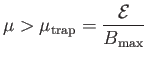 |
(2.88) |
is trapped on such a field-line. In fact, the particle undergoes
periodic motion along the field-line between two symmetrically placed (in  )
mirror points. The magnetic field-strength at the mirror points is
)
mirror points. The magnetic field-strength at the mirror points is
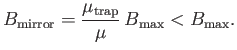 |
(2.89) |
On the midplane,
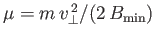 and
and
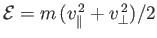 .
(From now on, for ease of notation, we shall write
.
(From now on, for ease of notation, we shall write
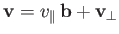 .) Thus, the trapping
condition, Equation (2.88), reduces to
.) Thus, the trapping
condition, Equation (2.88), reduces to
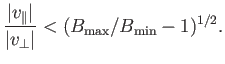 |
(2.90) |
Particles on the midplane that satisfy this inequality are trapped. On the other hand, particles
that do not satisfy the inequality escape along magnetic field-lines.
A magnetic mirror machine is incapable of trapping charged particles that
are moving parallel, or nearly parallel, to the direction of the magnetic field.
In fact, the previous inequality defines a loss cone in velocity space. (See
Figure 2.2.)
Figure 2.2:
A loss cone in velocity space. Particles whose velocity vectors lie inside the cone are
not reflected by the magnetic field.
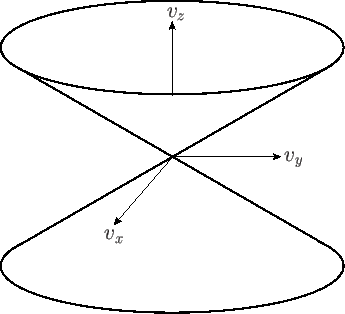 |
If plasma is placed inside a magnetic mirror machine then all
of the particles whose velocities lie in the loss cone promptly escape, but the
remaining particles are confined. Unfortunately, that is not
the end of the story. There is no such thing as an absolutely collisionless
plasma. Collisions take place at a low rate, even in very hot plasmas.
One important
effect of collisions is to cause diffusion of particles in velocity space (Hazeltine and Waelbroeck 2004).
Thus, collisions in a mirror machine continuously scatter trapped particles into
the loss cone, giving rise to a slow leakage of plasma out of the device.
Even worse, plasmas whose distribution functions deviate strongly from an isotropic
Maxwellian (for instance, a plasma confined
in a mirror machine) are prone to velocity-space instabilities (see Chapter 8) that tend to
relax the distribution function back to a Maxwellian. Such instabilities
can have a disastrous effect on plasma confinement in a mirror machine.



Next: Van Allen Radiation Belts
Up: Charged Particle Motion
Previous: Adiabatic Invariants
Richard Fitzpatrick
2016-01-23
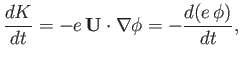
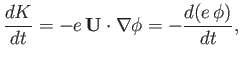

![]() ), so that the
), so that the
![]() drift
is similar in magnitude to the magnetic and curvature drifts. In this
case, the bounce point condition,
drift
is similar in magnitude to the magnetic and curvature drifts. In this
case, the bounce point condition,
![]() , reduces to
, reduces to
![]() on a magnetic field-line situated close to the axis of the device attains a
local minimum
on a magnetic field-line situated close to the axis of the device attains a
local minimum
![]() at
at ![]() , increases symmetrically
as
, increases symmetrically
as ![]() increases until
reaching a maximum value
increases until
reaching a maximum value
![]() at about the locations of the two
field-coils, and then decreases as
at about the locations of the two
field-coils, and then decreases as ![]() is further increased. According to
Equation (2.87), any particle that satisfies the inequality
is further increased. According to
Equation (2.87), any particle that satisfies the inequality
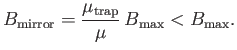
![]() and
and
![]() .
(From now on, for ease of notation, we shall write
.
(From now on, for ease of notation, we shall write
![]() .) Thus, the trapping
condition, Equation (2.88), reduces to
.) Thus, the trapping
condition, Equation (2.88), reduces to
