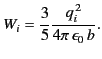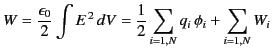Next: Electric Dipoles
Up: Electrostatic Fields
Previous: Electric Scalar Potential
Consider a collection of  static point charges
static point charges  , located at position vectors
, located at position vectors
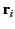 , respectively (where
, respectively (where 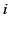 runs from 1 to
runs from 1 to 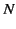 ).
Let us determine the electrostatic energy stored in such a collection. In other words, let us calculate the amount of work required to assemble
the charges, starting from an initial state in which they are all
at rest and very widely
separated.
).
Let us determine the electrostatic energy stored in such a collection. In other words, let us calculate the amount of work required to assemble
the charges, starting from an initial state in which they are all
at rest and very widely
separated.
The work we would have to do against electrical forces in order to slowly
move a charge 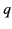 from point
from point 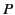 to point
to point 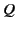 is
is
![$\displaystyle W = \int_P^Q (-{\bf F}) \cdot d{\bf r} =- q \int_P^Q {\bf E} \cdo...
...bf r} =q\int_P^Q \nabla\phi \cdot d{\bf r} = q \left[ \phi(Q) - \phi(P)\right],$](img399.png) |
(164) |
where use has been made of Equations (149) and (154).
Note that to move the charge we have to
exert on it a force 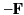 , where
, where 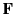 is specified in Equation (149), in order to counteract the force
exerted by the electric field. Recall that the scalar potential field
generated by a point charge
is specified in Equation (149), in order to counteract the force
exerted by the electric field. Recall that the scalar potential field
generated by a point charge 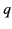 , located at position
, located at position  , is
, is
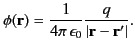 |
(165) |
Let us build up our collection of charges one by one. It takes no work to bring the
first charge from infinity, because there is no electric field to fight against.
Let us clamp this charge in position at 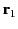 . In order to bring the
second charge into position at
. In order to bring the
second charge into position at 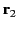 ,
we have to do work against the electric field
generated by the first charge. According to Equations (164) and Equations (165),
this work is given by
,
we have to do work against the electric field
generated by the first charge. According to Equations (164) and Equations (165),
this work is given by
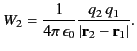 |
(166) |
Let us now bring the third charge into position. Because electric fields
and scalar potentials are
superposable, the work done while moving the third charge from infinity to  is simply the sum of the works done against the electric fields generated by
charges 1 and 2 taken in isolation: that is,
is simply the sum of the works done against the electric fields generated by
charges 1 and 2 taken in isolation: that is,
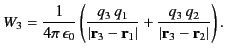 |
(167) |
Thus, the total work done in assembling the three charges is given by
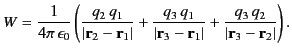 |
(168) |
This result can easily be generalized to 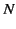 charges:
charges:
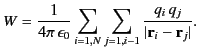 |
(169) |
The restriction that 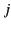 must be less than
must be less than 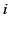 makes the above summation
rather cumbersome. If we were to sum without restriction (other than
makes the above summation
rather cumbersome. If we were to sum without restriction (other than 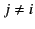 ) then
each pair of charges would be counted twice. It is convenient to do just
this, and then to divide the result by two. Thus, we obtain
) then
each pair of charges would be counted twice. It is convenient to do just
this, and then to divide the result by two. Thus, we obtain
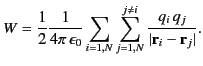 |
(170) |
This expression specifies the electrostatic potential energy of a collection of point charges. We can think of this energy as the
work required to bring stationary charges from infinity and assemble them in the
required formation. Alternatively, it is the kinetic energy that would
be released if the collection were dissolved, and the charges returned to infinity.
Let us investigate how the potential energy of a collection of electric charges is stored.
Equation (170) can be written
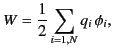 |
(171) |
where
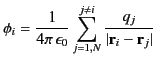 |
(172) |
is the scalar potential experienced by the 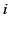 th charge due to the other
charges in the distribution.
th charge due to the other
charges in the distribution.
Let us now consider the potential energy of a continuous charge distribution.
It is tempting to write
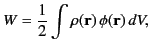 |
(173) |
by analogy with Equations (171) and (172), where
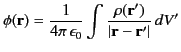 |
(174) |
is the familiar scalar potential generated by a continuous charge distribution
of charge density
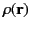 .
Let us try this ansatz out. We know from Equation (159) that
.
Let us try this ansatz out. We know from Equation (159) that
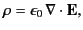 |
(175) |
so Equation (173) can be written
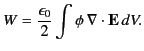 |
(176) |
Making use of the vector identity,
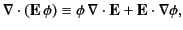 |
(177) |
as well as the fact that
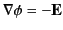 , we obtain
, we obtain
![$\displaystyle W = \frac{\epsilon_0}{2} \left[\int \nabla \cdot ({\bf E}\,\phi)\,dV + \int E^{\,2}\,dV\right].$](img419.png) |
(178) |
Application of the divergence theorem gives
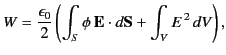 |
(179) |
where  is some volume that contains all of the charges, and
is some volume that contains all of the charges, and  is its bounding
surface. Let us assume that
is its bounding
surface. Let us assume that  is a sphere, centered on the origin, and let
us take the limit in which the radius
is a sphere, centered on the origin, and let
us take the limit in which the radius  of this sphere goes to infinity.
We know that, in general, the electric field a large distance
of this sphere goes to infinity.
We know that, in general, the electric field a large distance  from a
bounded charge
distribution looks like the field of a point charge, and, therefore,
falls off like
from a
bounded charge
distribution looks like the field of a point charge, and, therefore,
falls off like 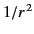 . Likewise, the potential falls off like
. Likewise, the potential falls off like 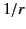 . However,
the surface area of a sphere of radius
. However,
the surface area of a sphere of radius  increases like
increases like 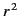 . Hence, it is clear that, in the
limit as
. Hence, it is clear that, in the
limit as
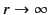 , the surface integral in Equation (179) falls off
like
, the surface integral in Equation (179) falls off
like 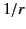 , and is consequently zero.
Thus, Equation (179) reduces to
, and is consequently zero.
Thus, Equation (179) reduces to
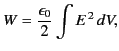 |
(180) |
where the integral is over all space. This expression implies that the potential energy of a continuous
charge distribution is stored in the electric field generated by the distribution, assuming that
this field possesses the energy density (see Section 1.9)
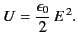 |
(181) |
We can easily check that Equation (180) is correct. Suppose that we have an amount of
charge 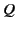 that is uniformly distributed within a sphere of
radius
that is uniformly distributed within a sphere of
radius  , centered on the origin. Let us imagine building up this charge distribution
from a succession of thin spherical layers of infinitesimal thickness. At each
stage, we gather a small amount of charge
, centered on the origin. Let us imagine building up this charge distribution
from a succession of thin spherical layers of infinitesimal thickness. At each
stage, we gather a small amount of charge 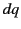 from infinity, and spread it
over the surface of the sphere in a thin
layer extending from
from infinity, and spread it
over the surface of the sphere in a thin
layer extending from  to
to 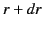 . We continue this process until the final radius of the
sphere is
. We continue this process until the final radius of the
sphere is  . If
. If 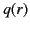 is the sphere's charge when it has attained radius
is the sphere's charge when it has attained radius
 then the work done in bringing a charge
then the work done in bringing a charge 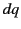 to its surface is
to its surface is
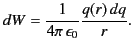 |
(182) |
This follows from Equation (166), because the electric field generated outside a spherical charge
distribution
is the same as that of a point charge 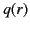 located at its geometric center
(
located at its geometric center
( ). If the uniform charge density of the sphere is
). If the uniform charge density of the sphere is
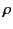 then
then
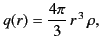 |
(183) |
and
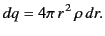 |
(184) |
Thus, Equation (182) becomes
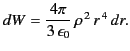 |
(185) |
The total work needed to build up the sphere from nothing to radius  is
plainly
is
plainly
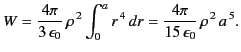 |
(186) |
This can also be written in terms of the total charge
 as
as
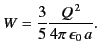 |
(187) |
Now that we have evaluated the potential energy of a spherical charge distribution
by the direct method, let us work it out using Equation (180). We shall assume that the
electric field is both radial and spherically symmetric, so that
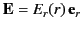 . Application of Gauss' law,
. Application of Gauss' law,
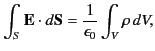 |
(188) |
where  is a sphere of radius
is a sphere of radius  , centered on the origin, gives
, centered on the origin, gives
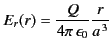 |
(189) |
for 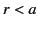 , and
, and
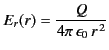 |
(190) |
for 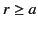 . Equations (180), (189), and (190) yield
. Equations (180), (189), and (190) yield
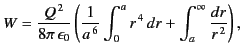 |
(191) |
which reduces to
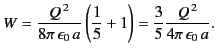 |
(192) |
Thus, Equation (180) gives the correct answer.
The reason that we have checked Equation (180) so carefully is that, on close inspection,
it is found to be
inconsistent with Equation (171), from which it was supposedly derived.
For instance, the energy given by Equation (180) is manifestly positive definite, whereas
the energy given by Equation (171) can be negative (it is certainly negative for
a collection of two point charges of opposite sign). The
inconsistency was introduced into our analysis when we replaced Equation (172) by
Equation (174). In Equation (172), the self-interaction of the 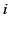 th charge with its
own electric field is specifically excluded, whereas it is included in Equation (174). Thus,
the potential energies
(171) and (180) are different because in the former we start from
ready-made point charges, whereas in the latter we build up the whole
charge distribution from scratch. Consequently, if we were to calculate the
potential energy of a point charge distribution using Equation (180) then
we would obtain the energy (171) plus the energy required to assemble the
point charges. However, the latter energy is infinite. To see this, let us suppose, for the sake of argument, that
our point charges actually consist of charge uniformly distributed in small
spheres of radius
th charge with its
own electric field is specifically excluded, whereas it is included in Equation (174). Thus,
the potential energies
(171) and (180) are different because in the former we start from
ready-made point charges, whereas in the latter we build up the whole
charge distribution from scratch. Consequently, if we were to calculate the
potential energy of a point charge distribution using Equation (180) then
we would obtain the energy (171) plus the energy required to assemble the
point charges. However, the latter energy is infinite. To see this, let us suppose, for the sake of argument, that
our point charges actually consist of charge uniformly distributed in small
spheres of radius 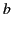 . According to Equation (187), the energy required to assemble the
. According to Equation (187), the energy required to assemble the
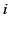 th point charge is
th point charge is
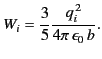 |
(193) |
We can think of this as the self-energy of the 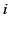 th charge.
Thus, we can write
th charge.
Thus, we can write
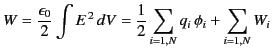 |
(194) |
which enables us to reconcile Equations (171) and (180). Unfortunately, if
our point charges really are point charges then
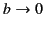 , and the
self-energy of each charge becomes infinite. Thus, the potential
energies specified by Equations (171) and (180) differ by an infinite amount.
We are forced to the conclusion that the idea of locating electrostatic
potential energy in the electric field is inconsistent with the existence of point charges. One way out of this difficulty would be to
say that elementary charges, such as electrons, are not points objects, but instead have finite spatial extents. Regrettably, there is no experimental
evidence to back up this assertion.
Alternatively, we could say that our classical
theory of electromagnetism breaks down on very small length-scales due to
quantum effects. Unfortunately, the quantum mechanical version of electromagnetism
(which is called quantum electrodynamics)
suffers from the same infinities in the self-energies of charged particles as the classical
version. There is a prescription, called renormalization, for steering round these
infinities, and getting finite answers that agree with experimental data to
extraordinary accuracy. However, nobody really understands why this prescription
works.
, and the
self-energy of each charge becomes infinite. Thus, the potential
energies specified by Equations (171) and (180) differ by an infinite amount.
We are forced to the conclusion that the idea of locating electrostatic
potential energy in the electric field is inconsistent with the existence of point charges. One way out of this difficulty would be to
say that elementary charges, such as electrons, are not points objects, but instead have finite spatial extents. Regrettably, there is no experimental
evidence to back up this assertion.
Alternatively, we could say that our classical
theory of electromagnetism breaks down on very small length-scales due to
quantum effects. Unfortunately, the quantum mechanical version of electromagnetism
(which is called quantum electrodynamics)
suffers from the same infinities in the self-energies of charged particles as the classical
version. There is a prescription, called renormalization, for steering round these
infinities, and getting finite answers that agree with experimental data to
extraordinary accuracy. However, nobody really understands why this prescription
works.



Next: Electric Dipoles
Up: Electrostatic Fields
Previous: Electric Scalar Potential
Richard Fitzpatrick
2014-06-27
![]() from point
from point ![]() to point
to point ![]() is
is
![]() . In order to bring the
second charge into position at
. In order to bring the
second charge into position at ![]() ,
we have to do work against the electric field
generated by the first charge. According to Equations (164) and Equations (165),
this work is given by
,
we have to do work against the electric field
generated by the first charge. According to Equations (164) and Equations (165),
this work is given by
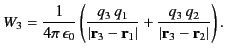
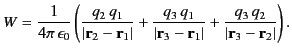
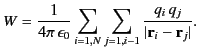
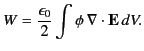
![$\displaystyle W = \frac{\epsilon_0}{2} \left[\int \nabla \cdot ({\bf E}\,\phi)\,dV + \int E^{\,2}\,dV\right].$](img419.png)
![]() that is uniformly distributed within a sphere of
radius
that is uniformly distributed within a sphere of
radius ![]() , centered on the origin. Let us imagine building up this charge distribution
from a succession of thin spherical layers of infinitesimal thickness. At each
stage, we gather a small amount of charge
, centered on the origin. Let us imagine building up this charge distribution
from a succession of thin spherical layers of infinitesimal thickness. At each
stage, we gather a small amount of charge ![]() from infinity, and spread it
over the surface of the sphere in a thin
layer extending from
from infinity, and spread it
over the surface of the sphere in a thin
layer extending from ![]() to
to ![]() . We continue this process until the final radius of the
sphere is
. We continue this process until the final radius of the
sphere is ![]() . If
. If ![]() is the sphere's charge when it has attained radius
is the sphere's charge when it has attained radius
![]() then the work done in bringing a charge
then the work done in bringing a charge ![]() to its surface is
to its surface is
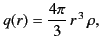
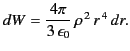
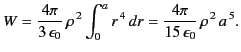
![]() . Application of Gauss' law,
. Application of Gauss' law,
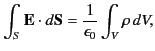
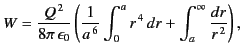
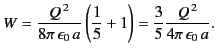
![]() th charge with its
own electric field is specifically excluded, whereas it is included in Equation (174). Thus,
the potential energies
(171) and (180) are different because in the former we start from
ready-made point charges, whereas in the latter we build up the whole
charge distribution from scratch. Consequently, if we were to calculate the
potential energy of a point charge distribution using Equation (180) then
we would obtain the energy (171) plus the energy required to assemble the
point charges. However, the latter energy is infinite. To see this, let us suppose, for the sake of argument, that
our point charges actually consist of charge uniformly distributed in small
spheres of radius
th charge with its
own electric field is specifically excluded, whereas it is included in Equation (174). Thus,
the potential energies
(171) and (180) are different because in the former we start from
ready-made point charges, whereas in the latter we build up the whole
charge distribution from scratch. Consequently, if we were to calculate the
potential energy of a point charge distribution using Equation (180) then
we would obtain the energy (171) plus the energy required to assemble the
point charges. However, the latter energy is infinite. To see this, let us suppose, for the sake of argument, that
our point charges actually consist of charge uniformly distributed in small
spheres of radius ![]() . According to Equation (187), the energy required to assemble the
. According to Equation (187), the energy required to assemble the
![]() th point charge is
th point charge is