


Next: Exercises
Up: Radiation and Scattering
Previous: Thomson Scattering
Let us now consider the scattering of electromagnetic radiation by a
harmonically bound electron: for instance, an electron orbiting
an atomic nucleus. We have seen in Section 7.3 that such an electron satisfies
an equation of motion of the form
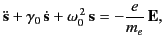 |
(1289) |
where 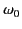 is the characteristic oscillation frequency of the electron,
and
is the characteristic oscillation frequency of the electron,
and
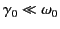 is the damping rate of such oscillations.
Assuming an
is the damping rate of such oscillations.
Assuming an
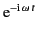 time dependence of both
time dependence of both 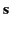 and
and  , we find that
, we find that
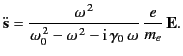 |
(1290) |
It follows, by analogy with the analysis in the previous section, that
the total scattering cross-section is given by
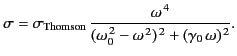 |
(1291) |
The angular distribution of the radiation is the same as that in the
case of a free
electron.
The maximum value of the cross-section (1293) is obtained when
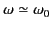 : that is, for resonant scattering. In this
case, the scattering cross-section can become very large. In fact,
: that is, for resonant scattering. In this
case, the scattering cross-section can become very large. In fact,
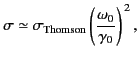 |
(1292) |
which is generally far greater than the
Thomson scattering cross-section.
For the case of strong binding,
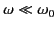 , and Equation (1293) reduces to
, and Equation (1293) reduces to
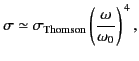 |
(1293) |
giving a scattering cross-section that depends on the inverse fourth
power of the wavelength of the incident radiation. The cross-section (1295)
is known as the Rayleigh scattering cross-section, and is appropriate
to the scattering of visible radiation by gas molecules. This
is the basis of Rayleigh's famous explanation of the blue sky. The air molecules of
the atmosphere preferentially scatter the shorter wavelength blue components
out of ``white'' sunlight which grazes the atmosphere. Conversely, sunlight
viewed directly through the long atmospheric path at sunset appears reddened.
The Rayleigh scattering cross-section is
much less than the Thompson scattering cross-section (for
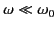 ). However, this effect is offset to some extent by the fact that the
density of neutral molecules in a gas (e.g., the atmosphere) is
much larger than the density of free electrons typically encountered
in a plasma.
). However, this effect is offset to some extent by the fact that the
density of neutral molecules in a gas (e.g., the atmosphere) is
much larger than the density of free electrons typically encountered
in a plasma.



Next: Exercises
Up: Radiation and Scattering
Previous: Thomson Scattering
Richard Fitzpatrick
2014-06-27
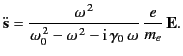
![]() : that is, for resonant scattering. In this
case, the scattering cross-section can become very large. In fact,
: that is, for resonant scattering. In this
case, the scattering cross-section can become very large. In fact,
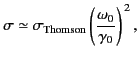
![]() , and Equation (1293) reduces to
, and Equation (1293) reduces to